ML Aggarwal Coordinate Geometry Chapter Test Class 9 ICSE Maths APC Understanding Solutions. Solutions of Chapter Test . This post is the Solutions of ML Aggarwal Chapter 19 – Coordinate Geometry for ICSE Maths Class-9. APC Understanding ML Aggarwal Solutions (APC) Avichal Publication Solutions of Chapter-19 Coordinate Geometry for ICSE Board Class-9. Visit official website CISCE for detail information about ICSE Board Class-9.
ML Aggarwal Coordinate Geometry Chapter Test Class 9 ICSE Maths Solutions
| Board | ICSE |
| Publications | Avichal Publishig Company (APC) |
| Subject | Maths |
| Class | 9th |
| Chapter-19 | Coordinate Geometry |
| Writer | ML Aggarwal |
| Book Name | Understanding |
| Topics | Solution of Chapter Test |
| Edition | 2021-2022 |
Chapter Test Solutions of ML Aggarwal for ICSE Class-9 Ch-19, Coordinate Geometry
Note:- Before viewing Solutions of Chapter – 19 Coordinate Geometry Class-9 of ML Aggarwal Solutions . Read the Chapter Carefully. Then solve all example given in Exercise-19.1, Exercise-19.2, Exercise-19.3, Exercise-19.4, MCQs, Chapter Test.
Coordinate Geometry Chapter Test
ML Aggarwal Class 9 ICSE Maths Solutions
Page 470
Question 1. Three vertices of a rectangle are A (2, -1), B (2, 7) and C(4, 7). Plot these points on a graph and hence use it to find the co-ordinates of the fourth vertex D Also find the co-ordinates of
(i) the mid-point of BC
(ii) the mid point of CD
(iii) the point of intersection of the diagonals. What is the area of the rectangle ?
Answer :
Given three vertices of a rectangle are A (2, -1), B (2, 7) and C(4, 7).
These points are marked on the graph shown below.
Join the points to form rectangle ABCD.
Also join the diagonals AC and BD.
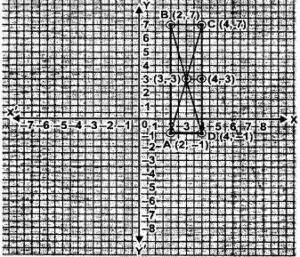
The coordinates of fourth vertex D is (4,-1).
(i) The midpoint of BC is (3,7).
(ii) The midpoint of CD is (4,3).
(iii) The point of intersection of diagonals is (3,3).
Area of the rectangle ABCD = AB×BC
= 8×2
= 16 sq. units.
Hence,
the area of the rectangle is 16 sq. units.
Question 2. Three vertices of a parallelogram are A (3, 5), B (3, -1) and C (-1, -3). Plot these points on a graph paper and hence use it to find the coordinates of the fourth vertex D. Also find the coordinates of the mid-point of the side CD. What is the area of the parallelogram?
Answer :
A (3, 5), B (3, -1) and C (-1, -3) are the three vertices of a parallelogram.
These points are marked on the graph shown below.
Join the points to form parallelogram ABCD.
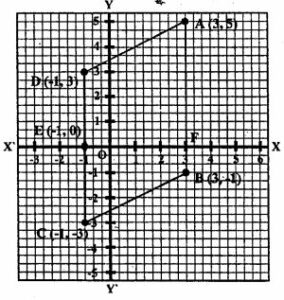
The coordinates of fourth vertex D is (-1,3).
The coordinates of midpoint of CD is (-1,0).
Area of parallelogram ABCD = Base ×height
= AB×EF
= 6×4
= 24 sq. units.
Hence the area of the parallelogram is 24 sq. units.
Question 3. Draw the graphs of the following linear equations.
(i) y = 2x – 1
(ii) 2x + 3y = 6
(iii) 2x – 3y = 4.
Also find slope and y-intercept of these lines.
Answer :
(i) y = 2x-1
when x = 1, y = 2×1 -1 = 1
when x = 2, y = 2×2 -1 = 4-1 = 3
when x = 3, y = 2×3 -1 = 6-1 = 5
| x | 1 | 2 | 3 |
| y | 1 | 3 | 5 |
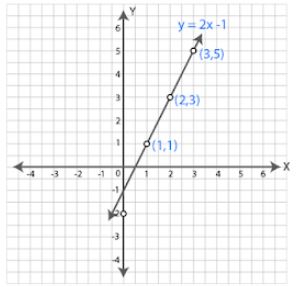
Slope of the line y = mx + c is m.
y intercept is c.
Slope of the line y = 2x-1 is m = 2.
Y intercept c = -1
the slope is 2 and y intercept is -1.
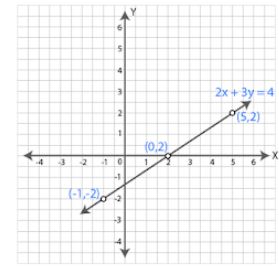
(ii) 2x+3y = 6
⇒ 3y = 6-2x
⇒ y = (6-2x)/3
when x = 0, y = (6- 2×0)/3 = 6/3 = 2
when x = 3, y = (6- 2×3)/3 = 0
when x = 6, y = (6- 2×6)/3 = -6/3 = -2
| x | 1 | 2 | 3 |
| y | 1 | 3 | 5 |

Slope of the line y = mx + c is m.
y intercept is c.
Slope of the line y = (6-2x)/3 is m = -2/3.
Y intercept c = 6/3 = 2
the slope is -2/3 and y intercept is 2.
(iii) 2x-3y = 4
⇒ 3y = 2x-4
⇒ y = (2x-4)/3
⇒ y = (2/3)x-4/3
When x = 2, y = (2×2 -4)/3 = 0
When x = 5, y = (2×5 -4)/3 = (10-4)/3 = 6/3 = 2
When x = -1, y = (2×-1 -4)/3 = -6/3 = -2
| x | 2 | 5 | -1 |
| y | 0 | 2 | -2 |
Slope of the line y = mx+c is m.
y intercept is c.
Slope of the line y = (2/3)x-4/3is m = 2/3.
Y intercept c = -4/3
Hence,
the slope is 2/3 and y intercept is -4/3.
Question 4. Draw the graph of the equation 3x – 4y = 12. From the graph, find :
(i) the value of y when x = -4
(ii) the value of x when y = 3.
Answer :
3x-4y = 12 …(i)
⇒ 4y = 3x-12
⇒ y = (3x-12)/4
When x = 0, y = (3×0 -12)/4 = -12/4 = -3
When x = 4, y = (3×4 -12)/4 = (12-12)/4 = 0
When x = 8, y = (3×8 -12)/4 = (24-12)/4 = 12/4 = 3
| x | 0 | 4 | 8 |
| y | -3 | 0 | 3 |
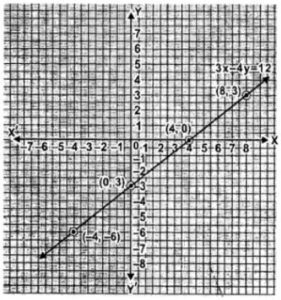
(i) When x = -4, the value of y is -6.
(ii) When y = 3, the value of x is 8.
Question 5. Solve graphically, the simultaneous equations: 2x – 3y = 7; x + 6y = 11.
Answer :
2x-3y = 7 …(i)
⇒ 3y = 2x-7
⇒ y = (2x-7)/3
When x = -1, y = (2×-1 -7)/3 = -9/3 = -3
When x = 2, y = (2×2 -7)/3 = -3/3 = -1
When x = 5, y = (2×5 -7)/3 = 3/3 = 1
| x | 0 | 4 | 8 |
| y | -3 | 0 | 3 |
x+6y = 11 …(ii)
⇒ 6y = 11-x
⇒ y = (11-x)/6
When x = -1, y = (11-(-1))/6 = 12/6 = 2
When x = 5, y = (11-5)/6 = 6/6 = 1
When x = 11, y = (11-11)/6 = 0
| x | -1 | 5 | 11 |
| y | 2 | 1 | 0 |
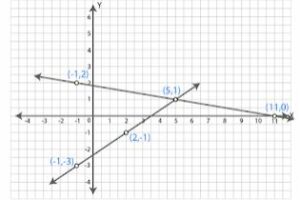
From the graph ,
it is clear that the two lines intersect at (5,1).
Hence,
x= 5 and y = 1.
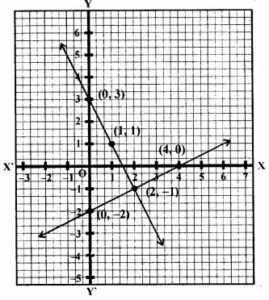
Question 6. Solve the following system of equations graphically: x – 2y – 4 = 0, 2x + y – 3 = 0.
Answer :
x – 2y – 4 = 0, 2x + y – 3 =0
x – 2y – 4 = 0
x = 2y + 4
By giving different values to y, we obtain the corresponding values of x
| x | 4 | 2 | 0 |
| y | 0 | -1 | -2 |
Now plot the points (4, 0), (2, -1) and (0, -2) on the graph and join them to obtain a line.
2x + y – 3 = 0
y = 3 – 2x
| x | 0 | 1 | 2 |
| y | 3 | 1 | -1 |
Now plot the points (0, 3), (1, 1) and (2, -1) on the graph and join them to obtain another line.
Both the lines intersect each other at
x = 2 and y = -1.
Question 7. Using a scale of l cm to 1 unit for both the axes, draw the graphs of the following equations : 6y = 5x:+ 10,y = 5;c-15. From the graph, find
(i) the coordinates of the point where the two lines intersect.
(ii) the area of the triangle between the lines and the x-axis.
Answer :
6y = 5x + 10 and y = 5x – 15
6y = 5x + 10
It can be written as
y = (5x + 10)/6
By giving different values to x, we obtain the corresponding values of y
| x | 1 | -2 | 4 |
| y | 2.5 | 0 | 5 |
Now, plot the points (1, 2.5), (-2, 0) and (4, 5) on the graph and join them to obtain a line.
y = 5x – 15
| x | 2 | 3 | 4 |
| y | -5 | 0 | 5 |
Now plot the points (2, -5), (3, 0) and (4, 5) on the graph and join them to obtain another line.
Both the lines intersect each other at x = 4 and y = 5.

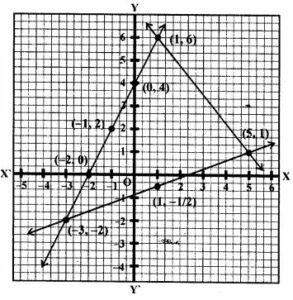
Question 8. Find, graphically, the coordinates of the vertices of the triangle formed by the lines : 8y – 3x + 7 = 0, 2x-y + 4 = 0 and 5x + 4y = 29.
Answer :
8y – 3x + 7 = 0, 2x – y + 4 = 0 and 5x + 4y = 29
8y – 3x + 7 = 0
8y = 3x – 7
⇒ y = (3x – 7)/8
By giving different values to x, we obtain the corresponding values of y
| x | 1 | 5 | -3 |
| y | – ½ | 1 | -2 |
Now plot the points (1, -1/2), (5, 1) and (-3, -2) on the graph and join them to obtain a line.
2x – y + 4 = 0
2x = y – 4
⇒ x = (y – 4)/2
By giving different values to y, we obtain the corresponding values of x
| x | -2 | -1 | 0 |
| y | 0 | 2 | 4 |
Now plot the points (-2, 0), (-1, 2) and (0, 4) on the graph and join them to obtain another line.
5x + 4y = 29
5x = 29 – 4y
⇒ x = (29 – 4y)/5
| x | 5 | 1 | -4 |
| y | 1 | 6 | 9 |
Now plot the points (5, 1), (1, 6) and (-4, 9) on the graph and join them to obtain another line.
These three lines intersect each other at (-3, -2), (1, 5) and (1, 6)
Hence,
the coordinates of the vertices of the triangle formed by these lines are (-3, -2), (1, 5) and (1, 6).
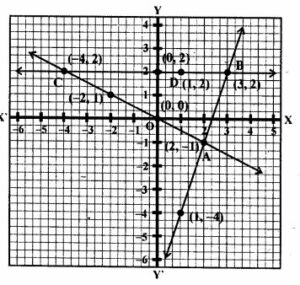
Question 9. Find graphically the coordinates of the vertices of the triangle formed by the lines y-2 = 0,2y + x = 0 and y + 1 = 3(x – 2). Hence, find the area of the triangle formed by these lines.
Answer :
y – 2 = 0
y = 2 which is parallel to x-axis
| x | 0 | 1 | 3 |
| y | 2 | 2 | 2 |
2y + x = 0
x = – 2y
| x | 0 | -2 | -4 |
| y | 0 | 1 | 2 |
Now plot the points (0, 0), (-2, 1) and (-4, 2) on the graph and join them to obtain a line.
y + 1 = 3 (x – 2)
y + 1 = 3x – 6
By giving different values to x, we obtain the corresponding values of y
| x | 1 | 2 | 3 |
| y | -4 | -1 | 2 |
Now plot the points (-2, 0), (-1, 2) and (0, 4) on the graph and join them to obtain another line.
We can see that the three lines intersect each other
Now the coordinates of the vertices of the triangle are (2, 1), (3, 2), (4, -2) and
Area of triangle = (BC × AB)/2
Substituting the values
= (7 × 3)/2
= 21/2
= 10.5 cm2
Question 10. A line segment is of length 10 units and one of its end is (-2,3). If the ordinate of the other end is 9, find the abscissa of the other end.
Answer :
Ordinates of the point on the other end (y) = 9
Consider abscissa = x
Distance between the two ends (-2, 3) and (x, 9) = √(x + 2)2 + (9 – 3)2
√(x + 2)2 + 62 = 10

x2 + 4x + 4 + 36 = 100
x2 + 4x = 100 – 36 – 4
⇒ x2 + 4x – 60 = 0
x2 + 10x – 6x – 60 = 0
⇒ x (x + 10) – 6 (x + 10) = 0
⇒ (x + 10) (x – 6) = 0
Here
x + 10 = 0
x = -10
x – 6 = 0
⇒ x = 6
Hence,
abscissa of the other end is – 10 or 6.
Question 11. A (-4, -1), B (-1, 2) and C (a , 5) are the vertices of an isosceles triangle. Find the value of a, given that AB is the unequal side.
Answer :
A (-4, -1), B (-1, 2) and C (α, 5) are the vertices of an isosceles triangle
AC = BC
AC = √(x2 – x1)2 + (y2 – y1)2
AC = √(α + 4)2 + (5 + 1)2
⇒ AC = √(α + 4)2 + 62
⇒ BC = √(α + 1)2 + (5 – 2)2
BC = √(α + 1)2 + 32
√(α + 4)2 + 62 = √(α + 1)2 + 32
(α + 4)2 + 62 = (α + 1)2 + 32
α2 + 8α + 16 + 36 = α2 + 2α + 1 + 9
8α – 2α = 1 + 9 – 16 – 36
So we get
6α = – 42
⇒ α = -42/6 = -7
Hence, the value of α is -7.
Question 12. If A (-3, 2), B (a, p) and C (-1, 4) are the vertices of an isosceles triangle, prove that α + β = 1, given AB = BC.
Answer :
A (-3, 2), B (α, β) and C (-1, 4) are the vertices of an isosceles triangle
AB = BC
Here
AB = √(α + 3)2 + (β – 2)2
BC = √(α + 1)2 + (β – 4)2
Now
AB = BC
√(α + 3)2 + (β – 2)2 = √(α + 1)2 + (β – 4)2
(α + 3)2 + (β – 2)2 = (α + 1)2 + (β – 4)2
α2 + 6α + 9 + β2 – 4β + 4 = α2 + 2α + 1 + β2 – 8β + 16
6α – 2α – 4β + 8β = 16 – 9 – 4 + 1
⇒ 4α + 4β = 4
Dividing by 4
α + β = 1
Hence, it is proved.
Question 13. Prove that the points (3, 0), (6, 4) and (-1, 3) are the vertices of a right angled isosceles triangle.
Answer :
Consider points A (3, 0), B (6, 4) and C (-1, 3) are the vertices of a right angled isosceles triangle.
AB = √(x2 – x1)2 + (y2 – y1)2
= √(6 – 3)2 + (4 – 0)2
= √32 + 42
= √9 + 16
= √25
= 5
BC = √(-1 – 6)2 + (3 – 4)2
= √(-7)2 + (-1)2
= √49 + 1
= √50
= √(25 × 2)
= 5 √2
AC = √(-1 – 3)2 + (3 – 0)2
= √(-4)2 + 32
= √16 + 9
= √25
= 5
Here
AB2 + AC2 = 52 + 52
= 25 + 25
= 50
= BC2
Hence, it is proved.
Question 14.
(i) Show that the points (2, 1), (0,3), (-2, 1) and (0, -1), taken in order, are the vertices of a square. Also find the area of the square.
(ii) Show that the points (-3, 2), (-5, -5), (2, -3) and (4, 4), taken in order, are the vertices of rhombus. Also find its area. Do the given points form a square?
Answer :
(i) Consider A (2, 1), B (0, 3), C (2, -1) and D (0, -1) taken in order are the vertices of the square
AB = √(x2 – x1)2 + (y2 – y1)2
= √(0 – 2)2 + (3 – 1)2
= √22 + 22
= √4 + 4
= √8
BC = √(-2 – 0)2 + (1 – 3)2
= √(-2)2 + (-2)2
= √4 + 4
= √8
CD = √(0 – 2)2 + (-1 – 1)2
= √22 + 22
= √4 + 4
= √8
CA = √(2 – 0)2 + (1 + 1)2
= √22 + 22
= √4 + 4
= √8
DA = √(2 – 0)2 + (1 + 1)2
= √22 + 22
= √4 + 4
= √8
AB = BC = CD = DA
Here ABCD is a square with side √8
Area = side2 = (√8)2 = 8 sq. units
(ii) Consider A (-3, 2), B (-5, -5), C (2, -3) and D (4, 4) taken in order are the vertices of a rhombus
AB = √(x2 – x1)2 + (y2 – y1)2
= √(-5 + 3)2 + (-5 – 2)2
= √(-2)2 + (-7)2
= √4 + 49
= √53
BC = √(2 + 5)2 + (-3 + 5)2
= √72 + 22
= √49 + 4
= √53
CD = √(4 – 2)2 + (4 + 3)2
= √22 + 72
So we get
= √4 + 49
= √53
DA = √(-3 – 4)2 + (2 – 4)2
= √(-7)2 + (-2)2
= √4 + 49
= √53
AB = BC = CD = DA
Here ABCD is a square or rhombus
Diagonal AC = √(2 + 3)2 + (-3 – 2)2
= √52 + 52
= √25 + 25
= √50
BD = √(4 + 5)2 + (4 + 5)2
= √92 + 92
So we get
= √81 + 81
= √162
AC ≠ BD
So, ABCD is a rhombus not a square
Area = Product of diagonal/2
= √50 × √162/ 2
= √(8100/2)
= 90/2
= 45 sq. units
Coordinate Geometry Chapter Test
ML Aggarwal Class 9 ICSE Maths Solutions
Page 471
Question 15. The ends of a diagonal of a square have co-ordinates (-2, p) and (p, 2). Find p if the area of the square is 40 sq. units.
Answer :
Ends of a diagonal of a square (-2, p) and (p, 2)
Area of square = 40 sq. units
Side = √40 units = 2 √10 units
Diagonal = √2 × side
= √2 × √40
= √80
= 4 √5 unit
Diagonal AC = √(x2 – x1)2 + (y2 – y1)2
√(p + 2)2 + (2 – p)2 = 4√5
By squaring on both sides
(p + 2)2 + (2 – p)2 = 16 × 5 = 80
Expanding using formula
p2 + 4p + 4 + 4 – 4p + p2 = 80
2p2 + 8 = 80
⇒ 2p2 = 80 – 8 = 72
p2 = 72/2 = 36 = (± 6)2
⇒ p = ± 6
Hence, the value of p is (6, -6).
Question 16. What type of quadrilateral do the points A (2, -2), B (7, 3), C (11, -1) and D (6, -6), taken in the order, form?
Answer :
A (2, -2), B (7, 3), C (11, -1) and D (6,-6), taken in that order are the vertices of a quadrilateral ABCD
AB = √(x2 – x1)2 + (y2 – y1)2
= √(7 – 2)2 + (3 + 2)2
= √52 + 52
= √25 + 25
= √50
BC = √(11 – 7)2 + (-1 – 3)2
= √42 + (-4)2
= √16 + 16
= √32
CD = √(6 – 11)2 + (-6 + 1)2
= √(-5)2 + (-5)2
= √25 + 25
= √50
DA = √(6 – 2)2 + (-6 + 2)2
= √42 + (-4)2
= √16 + 16
= √32
Here, AB = CD and BC = DA
Hence,
ABCD is a rectangle as the opposite sides are equal.
Question 17. Find the coordinates of the centre of the circle passing through the three given points A (5, 1), B (-3, -7) and C (7, -1).
Answer :
Consider (x, y) as the coordinates of the centre of the circle
Points A (5, 1), B (- 3, -7) and C (7, -1) are on the circle
OA = OB = OC
OA = √(x2 – x1)2 + (y2 – y1)2
= √(x – 5)2 + (y – 1)2
⇒ OB = √(x + 3)2 + (y + 7)2
⇒ OC = √(x – 7)2 + (y + 1)2
Here OA2 = OB2 and OA2 = OC2
(x – 5)2 + (y – 1)2 = (x + 3)2 + (y + 7)2
x2 – 10x + 25 + y2 – 2y + 1 = x2 + 6x + 9 + y2 + 14y + 49
6x + 14y + 10x + 2y = – 9 – 49 + 25 + 1
16x + 16y = – 32
Divide by 16
x + y = – 2
⇒ x = – 2 – y …(1)
OA2 = OC2
(x – 5)2 + (y – 1)2 = (x – 7)2 + (y + 1)2
Expanding using the formulas
x2 – 10x + 25 + y2 – 2y + 1 = x2 – 14x + 49 + y2 + 1 + 2y
–10x + 14x – 2y – 2y = 49 + 1 – 25 – 1
4x – 4y = 24
Dividing by 4
x – y = 6 …(2)
Substituting the value of (1) in (2)
(-2 – y) – y = 6
⇒ -2 – y – y = 6
-2y = 6 + 2
⇒ y = -8/2 = -4
Substituting the value of y in equation (1)
x = -2 – y
⇒ x = – 2 – (-4)
x = -2 + 4 = 2
Hence, the coordinates of the centre of the circle are (2, -4).
— : End of ML Aggarwal Coordinate Geometry Chapter Test Class 9 ICSE Maths Solutions :–
Return to :- ML Aggarawal Maths Solutions for ICSE Class-9
Thanks
Please Share with Your Friends


