ML Aggarwal Mid Point Theorem Chapter Test Class 9 ICSE Maths Solutions Ch-11. Step by Step Answer of Ch-Test questions on Mid Point Theorem of ML Aggarwal for ICSE Class 9th Mathematics. Visit official website CISCE for detail information about ICSE Board Class-9.
ML Aggarwal Mid Point Theorem Chapter Test Class 9 ICSE Maths Solutions Ch-11
| Board | ICSE |
| Subject | Maths |
| Class | 9th |
| Chapter-11 | Mid Point Theorem |
| Topics | Solution of Ch-Test Questions |
| Academic Session | 2024-2025 |
Ch-Test Questions on Mid Point Theorem
ML Aggarwal Class 9 ICSE Maths Solutions Ch-11
Question 1. ABCD is a rhombus with P, Q and R as midpoints of AB, BC and CD respectively. Prove that PQ ⊥ QR.
Answer : ABCD is a rhombus with P, Q and R as mid-points of AB, BC and CD
To prove: PQ ⊥QR, Join AC and BD
Proof: Diagonals of rhombus intersect at right angle
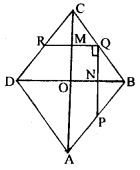
∠MON = 90° …(1)
In Δ BCD
Q and R are mid-points of BC and CD.
RQ || DB and RQ = ½ DB …(2)
RQ || DB
MQ || ON
∠MQN + ∠MON = 180°
∠MQN + 90° = 180°
⇒ ∠MQN = 180° – 90° = 90°
So, NQ ⊥MQ or PQ ⊥QR
Hence proved.
Question 2. The diagonals of a quadrilateral ABCD are perpendicular. Show that the quadrilateral formed by joining the mid-points of its adjacent sides is a rectangle.
Answer : ABCD is a quadrilateral in which diagonals AC and BD are perpendicular to each other

P, Q, R and S are mid-points of AB, BC, CD and DA
To prove: PQRS is a rectangle
Proof: P and Q are the mid-points of AB and BC
PQ || AC and PQ = ½ AC …(1)
S and R are mid-points of AD and DC
SR || AC and SR = ½ AC …(2)
Using both equations
PQ || SR and PQ = SR
So PQRS is a parallelogram
AC and BD intersect at right angles
SP || BD and BD ⊥AC
So SP ⊥ AC i.e. SP ⊥SR
∠RSP = 90°
⇒ ∠RSP = ∠SRQ = ∠RQS = ∠SPQ = 90°
Hence,
PQRS is a rectangle.
Question 3. If D, E, F are mid-points of the sides BC, CA and AB respectively of a ∆ ABC, Prove that AD and FE bisect each other.
Answer : D, E, F are mid-points of sides BC, CA and AB of a Δ ABC
To prove: AD and FE bisect each other
Construction: Join ED and FD
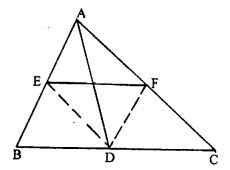
Proof: D and E are the midpoints of BC and AB
DE || AC and DE || AF …(1)
D and F are the midpoints of BC and AC
DF || AB and DF || AE …(2)
Using both equations
ADEF is a parallelogram
the diagonals of a parallelogram bisect each other
AD and EF bisect each other.
Hence proved.
Question 4. In ∆ABC, D and E are mid-points of the sides AB and AC respectively. Through E, a straight line is drawn parallel to AB to meet BC at F. Prove that BDEF is a parallelogram. If AB = 8 cm and BC = 9 cm, find the perimeter of the parallelogram BDEF.
Answer : In Δ ABC, D and E are the mid points of sides AB and AC
DE is joined from E
EF || AB is drawn AB = 8 cm and BC = 9 cm
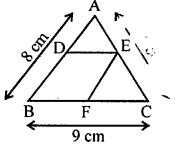
To prove: (i) BDEI is a parallelogram
(ii) Find the perimeter of BDEF
Proof: In Δ ABC
B and E are the mid-points of AB and AC
Here DE || BC and DE = ½ BC
So EF || AB
DEFB is a parallelogram
DE = BF
DE = ½ BC = ½ × 9 = 4.5 cm
EF = ½ AB = ½ × 8 = 4 cm
Perimeter of BDEF = 2 (DE + EF)
= 2 (4.5 + 4)
= 2 ×8.5
= 17 cm
Question 5. In the given figure, ABCD is a parallelogram and E is mid-point of AD. DL EB meets AB produced at F. Prove that B is mid-point of AF and EB = LF.

Answer : ABCD is a parallelogram
E is the mid-point of AD
DL || EB meets AB produced at F
To prove: EB = LF
B is the mid-point of AF
Proof: BC || AD and BE || LD
BEDL is a parallelogram
BE = LD and BL = AE
Here E is the mid-point of AD
L is the mid-point of BC
In Δ FAD
E is the mid-point of AD and BE || LD at FLD
So,
B is the mid-point of AF
EB = ½ FD = LF
Question 6. In the given figure, ABCD is a parallelogram. If P and Q are mid-points of sides CD and BC respectively. Show that CR = 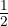 AC.
AC.

Answer : ABCD is a parallelogram
P and Q are mid-points of CD and BC
To prove: CR = ¼ AC
Construction: Join AC and BD
Proof: In parallelogram ABCD
Diagonals AC and BD bisect each other at O
AO = OC or OC = ½ AC …(1)
In Δ BCD
P and Q are mid points of CD and BC
PQ || BD
In Δ BCO,
Q is the mid-point of BC and PQ || OB
Is the mid-point of CO
CR = ½ OC = ½ (½ BC)
⇒ CR = ¼ BC
Hence proved.
— : End of ML Aggarwal Mid Point Theorem Chapter Test Class 9 ICSE Maths Solutions :–
Return to :- ML Aggarawal Maths Solutions for ICSE Class-9
Thanks
Please Share with Your Friends


