Compound Interest Class 9 OP Malhotra Exe-2D ICSE Maths Solutions Ch-2 We Provide Step by Step Solutions / Answer of Questions on Growth and Depreciation OP Malhotra Maths . Visit official Website CISCE for detail information about ICSE Board Class-9 Mathematics.
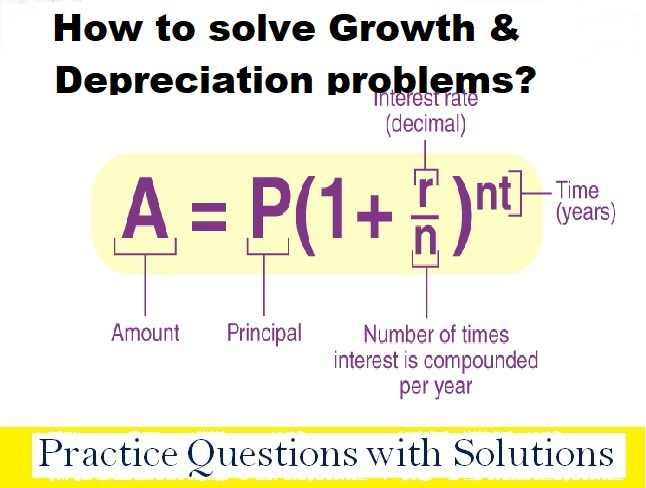
Compound Interest Class 9 OP Malhotra Exe-2D ICSE Maths Solutions Ch-2
| Board | ICSE |
| Publications | S Chand |
| Subject | Maths |
| Class | 9th |
| Chapter-2 | Compound Interest |
| Writer | OP Malhotra |
| Exe-2D | Growth and Depreciation |
| Edition | 2025-2026 |
Exercise- 2D
Compound Interest Class 9 OP Malhotra ICSE Maths Solutions Ch-2
Que-1: The cost of a machine depreciates by 10% every year. If its present value is ₹ 18,000; what will be its value after three years.
Sol: Present value of machine (P) = ₹ 18000
Rate of depreciation (R) = 10% p.a.
Period (n) = 3 years
∴ Value of after 3 years = P (1 – (R/100))^n
= ₹ 18000 (1−(10/100))³
= ₹ 18000 (9/10)³
= ₹ 18000 x (9/10) x (9/10) x (9/10)
= ₹ 13122
Que-2: The population of a town increased by 20% every year. If its present population is 2,16,000, find it population (i) after 2 years (ii) 2 years ago.
Sol: Present population = 216000
Rate of increase = 20% p.a.
Period (n) = 2 years
(i) ∴ Population after 2 years = P ( 1 + (R/100))^n
= 216000 ( 1 + (20/100))²
= 216000 x (6/5)²
= 216000 x (6/5) x (6/5)
= 8640 x 36 = 3,11,040
(ii) Population 2 years ago
= A ÷ ( 1 + (R/100))^n
= 216000 ÷ ( 1 + (20/100))²
= 216000 ÷ (6/5)²
= 216000 × (5/6) x (5/6)
= 1,50,000.
Que-3: The machinery of a certain factory is valued at ₹ 18,400 at the end of 1980. If it is supposed to depreciate each year at 8% of the value at the beginning of the year, calculate the value of the machine at the end of 1979 and 1981.
Sol: Value machinery at the end of 1980 = ₹ 18400
P of depreciation (R) = 8% p.a.
(a) Period = 1 year ago i.e., in 1979
∴ P = A ÷ ( 1 – (R/100))
= 18400 ÷ ( 1 – (8/100))
= 18400 ÷ (23/25)
= 18400 × (25/23)
= 20, 000.
(b) Population after one year i.e., in 1981
= P ( 1 – (R/100))
= 18400( 1 – (8/100))
= 18400 x (23/25)
= 16928.
Que-5: The present value of a scooter is ₹ 15,360.
If its value depreciates 12*(1/2) % every’ year, find its value after 3 years.
Sol: Present value of scooter (P) = ₹ 15360
Rate of depreciation (R) = 12*(1/2) % = 25/2 % p.a.
Period (n) = 3 years
∴ Value after 3 years = P ( 1 – (R/100))^n
= ₹ 15360 x ( 1 – (25/(20×100)))³
= ₹ 15360 x (7/8)³
= ₹ 15360 x (7/8) x (7/8) x (7/8)
= ₹ 10290.
Que-5: A new car is purchased for ₹ 12,50,000. Its value depreciates at the rate of 10% in the first year, 8% in the 2nd year and then 6% every year. Find its value after 4 years.
Sol: Value of car (P) = ₹ 2,50,000
Rate of depreciation (R) = 10% in first year, 8% in second year and then 6% every year Value of car after 4 years
= P(1−(R1)/100) (1−(R2)/100) (1−(R3)/100) (1−(R4)/100)
= ₹ 2,50,000 (1−(10/100)) (1−(8/100)) (1−(6/100)) (1−(6/100))
= ₹ 2,50,000 x (9/10) x (23/25) x (47/50) x (47/50)
= ₹ 182905.20
Que-6: The bacteria in a culture grows by 10% in the first hour, decreases by 10% in the second hour and again increases by 10% in the third hour. If the original count of the bacteria in a sample is 10000, find the bacteria count at the end of 3 hours.
Sol: Original number of bacteria (P) = 10000
Increase in first hour (R1) = 10%
Decrease in second hour (R2) = 10%
Increase in third hour (R3) = 10%
∴ Number of bacteria after 3 hours
= P (1+(R1)/100) (1−(R2)/100) (1+(R3)/100)
= 1000 (1+(10/100)) (1−(10/100)) (1+(10/100))
= 1000 (11/10) × (9/10) × (11/10)
= 10890.
Que-7: The production of refrigerators in factory rose from 40000 to 48400 in 2 years. Find the rate of growth p.a.
Sol: Present production (P) = 40000
Production after 2 years (A) = 48400
Period (n) = 2 years
Let rate of increase = R% p.a.
A = P ( 1 + (R/100))^n
A/P = ( 1 + (R/100))^n
48400/40000 = ( 1 + (R/100))²
121/100 = ( 1 + (R/100))²
(11/10)² = ( 1 + (R/100))²
Comparing both sides,
( 1 + (R/100)) = 11/10
R/100 = (11/10) – 1
R/100 = 1/10
R = 100/10
R = 10%.
Que-8: The value of a flat worth ₹ 5,00,000 is depreciating at the rate of 10% p.a. In how many years will its value be reduced to ₹ 364500?
Sol: Present value of plot (P) = ₹ 5,00,000
Value after depreciation (A) = ₹ 3,64,500
Rate of depreciation (R) = 10% p.a.
Let period = n years
A = P ( 1 – (R/100))^n
A/P = ( 1 – (R/100))^n
364500/500000 = ( 1 – (10/100))^n
729/1000 = (9/10)^n
(9/10)³ = (9/10)^n
Comparing both sides, n = 3
Period = 3 years.
Que-9: Rachit bought a flat for ₹ 10 lakh and a car for ₹ 3,20,000 at the same time. The price of the flat appreciates uniformly at the rate of 20% p.a.; while the price of the car depreciates at the rate of 15% p.a. If Rachit sells the flat and car after 3 years, what will be his profit or loss?
Sol: Price of flat = ₹ 10,00,000
and price of car = ₹ 3,20,000
Rate of appreciation of flat (R1) = 20%
Rate of depreciation of car (R2) = 15%
Period (n) = 3 years
(a) Value of flat after 3 years = P ( 1 + (R/100))^n
= 10,00,000 ( 1 + (20/100))³
= 10,00,000 (6/5)³
= 10,00,000 × (6/5) × (6/5) × (6/5)
= 17,28,000
Value of car after 3 years = P ( 1 – (R/100))^n
= 3,20,000 ( 1 – (15/100))³
= 3,20,000 (17/20)³
= 3,20,000 × (17/20) × (17/20) × (17/20)
= 1,96,520.
Total cost of flat and car = ₹ 10,00,000 + ₹ 3,20,000 = ₹ 3,20,000
and selling price = ₹ 17,28,000 + ₹ 1,96,520 = ₹ 19,24,520
Gain = ₹ 19,24,520 – ₹ 13,20,000
= ₹ 16,04,520
Que-10: 8000 workers were employed by a company to complete a job in 4 years. At the end of first year, 5% of the workers were retrenched. At the end of second year, 5% of those working at that time were retrenched. However to complete the job in time, the number of workers was increased by 10% of those working at the end of third year. How many workers were working during the fouth year ?
Sol: Number of workers in the beginning = 8000
Rate of retrenchment after first year = 5%
Rate of retrenchment after second year = 5%
Rate of increase after the third year = 10%
∴ Number of workers after third year or in the beginning of fourth year
= 8000 (1−(5/100)) (1−(5/100)) (1+(10/100))
= 8000 x (19/20) x (19/20) x (11/10)
= 7942
— : End of Compound Interest Class 9 OP Malhotra Exe-2D ICSE Maths Ch-2 Step by Step Solutions :–
Return to :– OP Malhotra S Chand Solutions for ICSE Class-9 Maths
Thanks
Please Share with Your Friends


