Compound Interest Class 9 RS Aggarwal Exe-2B Goyal Brothers ICSE Foundation Mathmatics Solutions. In this article you will learn how to solve compound interest problems using FORMULA . Visit official Website CISCE for detail information about ICSE Board Class-9 Mathematics.
Compound Interest Class 9 RS Aggarwal Exe-2B Goyal Brothers ICSE Maths Solutions
| Board | ICSE |
| Publications | Goyal brothers Prakashan |
| Subject | Maths |
| Class | 9th |
| Chapter-2 | Compound Interest |
| Writer | RS Aggrawal |
| Book Name | Foundation |
| Topics | Solution of Exe-2B |
| Academic Session | 2024-2025 |
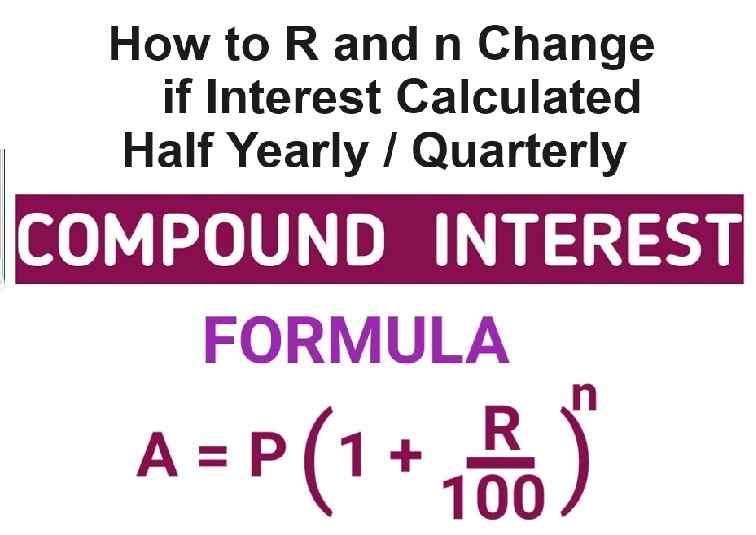
How to Solve Compound Interest Problems using FORMULA
The formula for compound interest is A = P (1+ r / 100 )n ,
- A = Total Amount.
- P = Originall Principal.
- r = Rate of interest per term (not yearly)
- n = number of term. It can be monthly, half-yearly, quarterly, or yearly.
- t = time in years must be converted into n (term) such that if C.I calculated yearly then n = t, if calculated half yearly then n = 2 t and if calculated quaterly then n = 4 t.
Note : Never ignore step 5 before start calculation. following table is suitable if C.I calculated in yearly mode.
|
Time (in years) |
Amount | Compund Interest |
| 1 | P(1 + R/100) | Final Amount – Original Principal |
| 2 | P(1+R100)2 | Final Amount – Original Principal |
| 3 | P(1+R100)3 | Final Amount – Original Principal |
| 4 | P(1+R100)4 | Final Amount – Original Principal |
| n | P(1+R100)n | Final Amount – Original Principal |
Exercise- 2B
Compound Interest Class 9 RS Aggarwal Exe-2B Goyal Brothers ICSE Foundation Mathmatics Solutions
Page- 36,37
Que-1: Calculate the amount and the compound interest on Rs10000 for 2 years at 8% p.a., compounded annually.
Solution- Principal (P) = Rs. 10000
Rate Of Interest = 8%
Time (n) = 2 years
A = P(1+(R/100))^n
A = 10000(1+(8/100))²
A = 10000 (1+0.08)²
A = 10000(1.008)²
A = 10000 x 1.1664
A = 11664 Ans.
C.I. = A-P
C.I. = 11664 – 10000
C.I. = 1664 Ans.
Que-2: Calculate the amount and the compound interest on Rs64000 for 3 years at 7*(1/2)% p.a., compounded annually.
Solution- Principal = 64000
Rate = 7.5%p.a
Time = 3years
Where r is the rate and t is the time.
A = 64000(1+7.5/100)³
= 64000(1+75/1000)³
= 64000(1+3/40)³
= 64000×(43/40)³
= 64000×(43/40×43/40×43/40)
= (43×43×43)
= 79507 Ans.
C.I. = A-P
C.I. = 79507 – 64000
C.I. = 15507
Hence CI will be 15507 rupees
Que-3: How much will Rs12000 amount to in 2 years at compound interest, the rate of interest for the successive years being 10% and 11% respectively.
Solution- P = Rs.12000, Time = 2 Years, Rate = 10% and 11%
A = P(1+R/100)^n
⇒ 12000(1+10/100)(1+11/100)
⇒ 12000×(110/100)×(111/100)
⇒ Rs14652 Ans
Que-4: Calculate the amount and the compound interest on Rs25000 for 3 years, the rates of interest for the successive years being 8%, 9% and 10%, compounded annually.
Solution- Principal (P) = Rs 25,000
Rate of interest for the first year (R1) = 8%
Time (T) = 1 year
𝐴1 = 𝑃(1+(𝑅1/100))
𝐴1 = 25000(1+(8/100))
A1 = 25000(1+0.08)
A1 = 25000×1.08
A1 = 27000
New Principal = Amount at the end of the first year = Rs 27,000
Rate of interest for the second year (R2) = 9%
Time (T) = 1 year
𝐴2 = 𝐴1(1+(𝑅2/100))
𝐴2 = 27000(1+(9/100))
A2 = 27000(1+0.09)
A2 = 27000×1.09
A2 = 29430
New Principal = Amount at the end of the second year = Rs 29,430
Rate of interest for the third year (R3) = 10%
Time (T) = 1 year
A3 = A2(1+(R3/100))
𝐴3 = 29430(1+(10/100))
A3 = 29430(1+0.10)
A3 = 29430×1.10
A3 = 32373
Thus, the amount at the end of 3 years is Rs 32,373 Ans.
Compound Interest (CI) = Amount – Principal
Principal (P) = Rs 25,000
Amount (A) = Rs 32,373
𝐶𝐼 = 𝐴−𝑃
= 32373−25000 = 7373 Ans.
Que-5: Find the amount and the compound interest on Rs7500 for 2 year 8 months at 10% p.a., compounded annually.
Solution-Principal (P) = Rs 7,500
Rate of interest (R) = 10% per annum
Time (T) = 2 years
𝐴2 = 𝑃(1+𝑅/100)^n
𝐴2 = 7500(1+10/100)²
A2 = 7500(1+0.10)²
A2 = 7500×(1.1)²
A2 = 7500×1.21
A2 = 9075
New Principal = Amount at the end of 2 years = Rs 9,075
Time (T) for the remaining months = 8/12 = 2/3 years
𝐴 = 𝐴2[1+(𝑅×𝑇/12)/100]
𝐴 = 9075[1+(10×8/12)/100]
A = 9075(1+(10×23)/100)
A = 9075(1+20/30)
A = 9075(1+0.0667)
A = 9680 Ans.
C.I. = A-P
C.I. = 9680 – 7500
C.I. = 2180 Ans.
Que-6: If the simple interest on the sum of money for 3 years at 8% per annum is Rs7500, find the compound interest on the same sum, for the same period at the same rate.
Solution- Interest (I) = Rs 7500
Rate (R) = 8% p.a
Time (T) = 3years
Interest = P × R × T / 100
⟹ Principal = I × 100/R × T
⟹ Principal = 7500 × 100/8 × 3
⟹ Principal = 750000/24
⟹ Principal = 375000/12
⟹ Principal = 187500/6
⟹ Principal = Rs 31250
⟼ Amount = P [1 + R / 100]^n
⟼ A = 31250 [1 + 8/100]³
⟼ A = 31250 [108/100]³
⟼ A = 31250 [54/50]³
⟼ A = 31250 [27/25]³
⟼ A = 31250 × 27/25 × 27/25 × 27/25
⟼ A = 31250 × 729/625 × 27/25
⟼ A = 31250 × 19683/15625
⟼ A = 2 × 19683
⟼ A = Rs 39366
⟼ CI = Amount – Principal
⟼ CI = 39466 – 31250
⟼ Compound Interest = Rs8,116 Ans.
Que-7: Calculate the amount and the compound interest on Rs16000 for 1 years at 15% p.a., compounded half-yearly.
Solution- A = P(1+r/200)^2n, P = 16000, n = 1 , r= 15%
A = 16000(1+15/200)^(2×1)
=> 16000 ( 1+3/40)²
=> 16000 ( 43/40)²
=> (16000 × 43 × 43)/(40×40)
=> 10×43×43
=> Rs 18,490
A = 18,490
I = A – P
=> 18490 – 16000
=> Rs 2,490
therefore A = 18490 and C.I = Rs 2,490
Que-8: Find the amount and the compound interest on Rs125000 for
1*(1/2) years at 12% p.a., compounded half-yearly.
Solution- P = 125000
T = 1 and 1/2 yrs = 3/2 (3half years)
R = 12% per annum (6%semi annum)
A = {12500(1+6/100)^3}
{12500(100+6/100)^3}
{12500(106/100)^3}
{12500(53/50)^3}
12500 x 53/50 x 53/50 x 53/50
53 x 53 x 53
= 148877 Ans.
C. I = A-P
148877-125000
= 23877 Ans.
Que-9: A sum of Rs12500 is deposited for 1*(1/2) years, compounded half-yearly. Its amount to Rs13000, at the end of first half year. Find :
(i) The rate of interest (ii) The final amount. Give your answer correct to nearest rupee.
Solution- Since, An amount P is deposited in annual rate of r (in decimals) for t years and compounded half years.
Then after t years the final amount is,
A = P(1+r/2)^2n
(i) Here, After 1 year,
A = 13,000
t = 1/2 years
P = 12,500
12500(1+r/2)¹ = 13000
(1+r/2) = 13000/12500
(1+r/2) = 1.04
r/2 = 1.04 – 1
r = 0.-4 x 2
r = 0.08
Thus, the annual rate = 0.08 = 8% Ans.
(ii) Now, P = 125,000
t = 1½ years = 3/2 years
r = 8%
Thus, A = 12500(1+0.08/2)^2×3/2
A = 12500(1+0.08/2)³
A = 12500 x 1.124864
A = 14061 Ans.
Que-10: The simple interest on a sum of money at 12% per annum for 1 year is Rs900. Find : (i) the sum of money and (ii) the compound interest on this sum for 1 year, payable half yearly at the same rate
Solution- Simple interest = Rs.900
Time = 1 year
Rate of interest = 12%
(i) S.I. = (PxRxT)/100
900 = (P x 12 x 1)/100
P = (900 x 100)/12
P = 7500 Ans.
(ii) Sum = 7500
Rate of interest = 12% = 0.12
Time = 1 year
No. of compounds per year = n = 2
A = P(1+R/n)^nt
A = 7500(1+0.12/2)^2×1
A = 7500(1+0.06)²
A = 7500(1.06)²
A = 8427
Compound interest = Amount – Principal
= 8427 – 7500
C.I. = 927 Ans.
Que-11: What sum of money will amount to Rs18150 in 2 year at 10% per annum, compounded annually ?
Solution- A = ₹18,150
T = 2 years
R = 10 % p.a.
P = ?
A = P(1 + R/100)^n
=> 18,150 = P(1 + 10/100)^2
=> 18,150 = P(100 + 10/100)^2
=> 18,150 = P(110/100)^2
=> 18,150 = P(11/10)^2
=> 18,150 = P × 121/100
=> P = 18,150 × 100/121
=> P = ₹15,000 Ans.
Que-12: What sum of money will amount to Rs93170 in 3 year at 10% per annum, compounded annually ?
Solution- A = 93170
r = 10%
n = 3 years
P = ?
A = P(1 + R/100)^n
93170 = P(1+10/100)³
93170 = P(110/100)³
P = 93170 x 100/110 x 100/110 x 100/110
P = Rs70000 Ans.
Que-13: On what sum of money will the compound interest for 2 years at 8% per annum be Rs7488 ?
Solution- C.I. = Rs7488
n = 2 years
r = 8%
P = ?
C.I. = P(1+r/100)^n – 1
7488 = P(1+8/100)² – 1
7488 = P(1+0.08)² – 1
7488 = P(1.08)² – 1
7488 = P(0.1664)
P = 7488/0.1664
P = 45000 Ans.
Que-14: The difference between the simple interest and the compound interest on a sum of money for 2 years at 12% per annum is Rs216. Find the sum.
Solution- (PxRxT)/100 = P(1+r/100) – 1
(Px2x22)/100 – (112/100) – 1
6P/2 – (784-625)/625
159/625 = 216
(159p-150p)625
9p/625 = 216
p = (216×625)/9
p = 15000 Ans.
Que-15: The difference between the simple interest and the compound interest on a sum of money for 3 years at 10% per annum is Rs558. Find the sum.
Solution- The difference in compound interest and simple interest for 3 years = Rs 558
Rate of interest = 10%
Simple interest = principal × rate × time/100
Compound interest = p[(1 + r/100)t – 1]
Let the principal be p
558 = p[(1 + 10/100)3 – 1] – 3p/10
⇒ 558 = p[331/1000 – 3/10]
⇒ p = 558 × (1000/31) = 18000
∴ The sum is Rs 18000 Ans.
Que-16: The difference between the compound interest for 1 year, compounded half-yearly and the simple interest for 1 year on a certain sum of money at 10% per annum is Rs360. Find the sum.
Solution- Let the principal, P = Rs. x, r = 10%, t = 1 year
Using S.I. = Prt/100,
S.I. = Rs.(x × 10 × 1)/100 = Rs. x/10
No of conversion period, n = 2 × 1 = 2,
r = 10/2 = 5% per conversion period, P = x,
Using C.I. = P [(1 + r/100)n – 1],
C.I. = x [(1 + 5/100)2 – 1]
= x(21/20 ×21/20 – 1)
= x(441/400 – 1)
= (41/400)x
As per problem, C.I. – S.I. = Rs360
Or, Rs.(41/400)x – Rs. x/10 = 360
Or, Rs (41/400 – 1/10)x = 360
Or, Rs x/400 = 360
Or, x = Rs360 × 400 = Rs144000 Ans.
Que-17: At what rate per cent per annum compound interest will Rs6250 amount to Rs7290 in 2 years ?
Solution- Principal amount (P) = Rs6250
Amount (A) = Rs7290
Time (t) = 2 years
A = P(1 + r/100)^t
Substituting the given values in the above formula, we get:
7290 = 6250(1 + r/100)^2
Taking square root on both sides, we get:
√(7290/6250) = 1 + r/100
r/100 = √(7290/6250) – 1
r/100 = 0.08
r = 8% Ans.
Que-18: At what rate per cent per annum will Rs3000 amount to Rs3993 in 3 years, the interest being compounded annually ?
Solution- Principal = Rs.3000
Amount after 3 years = Rs.3993
Amount = Principal (1 + Rate/100)^t
Ratio of principal and amount = 3993/3000 = 1331/1000
Cube root of ratio = ∛(1331/1000) = 1.1
After 1-year amount is 1.1 times that of principal
Percentage change = (1.1 -1) × 100 = 10%.
∴ Rate of Interest is 10% per annum.
Que-19: In what time will Rs5120 amount to Rs7290 at 12*(1/2)% per annum, compounded annually ?
Solution- Amount = Rs7290
Principal = Rs5120
rate = 25/2 %
A = P(1 + r/100)^t
7290 = 5120 (1+(25/2×100))^t
7290/5120 = (1+1/8)^t
729/512 = (9/8)^t
(9/8)³ = (9/8)^t
t = 3 years.
Que-20: A certain sum of money amounts to Rs7260 in 2 years and to Rs7986 in 3 years, interest being compounded annually. Find the rate per cent per annum.
Solution- Sum of ₹ P invested at the rate of r % per annum compounded annually amounts to ₹ 7260 in 2 years.
A = P(1 + r/100)^t
7260 = P(1+r/100)² …………..(1)
Sum of ₹ P invested at the rate of r % per annum compounded annually amounts to ₹ 7986 in 3 years.
7986 = P(1+r/100)³ ……………(2)
On dividing equation (2) by (1), we get
1 + r/100 = 7986/7260
r/100 = 7986/7260 – 1
–: End of Compound Interest Class 9 RS Aggarwal Exe-2B Goyal Brothers ICSE Maths Solutions : —
Return to :- RS Aggarwal Solutions for ICSE Class-9 Mathematics (Goyal Brother Prakashan)
Thanks
Please Share with your friends if helpful