Energy and Its Conservation Numerical Class 11 Nootan ISC Physics Solutions Ch-9. Step by step Solutions of Kumar and Mittal Physics of Nageen Prakashan as council latest prescribe guideline for upcoming exam. Visit official Website CISCE for detail information about ISC Board Class-11 Physics.
Energy and Its Conservation Numerical Class 11 Nootan ISC Physics Solutions
| Board | ISC |
| Class | 11 |
| Subject | Physics |
| Writer | Kumar and Mittal |
| Publication | Nageen Prakashan |
| Chapter-9 | Work Energy and Power |
| Topics | Numericals on Energy and Its Conservation |
| Academic Session | 2024-2025 |
Numericals on Energy and Its Conservation
Ch-9 Work Energy and Power Class 11 Nootan ISC Physics Solutions of Kumar and Mittal Physics , Nageen Prakashan
Question-10: A force of 30 N acts on a body of mass 2.0 kg starting from rest up to a distance of 3.0 m. Then the force reduces to 15 N and acts in the same direction up to 2.0 m . Calculate the final kinetic energy of the body .
Answer- Total work done = gain in K.E.
=> F1 s1 + F2 s2
=> 30 x 3 + 15 x 2
Hence > 120 J
Question-11: A 0.2 kg ball is suspended by a thread of length 1 m . It is pulled aside until the thread makes an angle of 30° with the vertical. How much work is done against gravity. The ball is now released. Find its velocity at the lowest point. Ignore air resistant and take g = 10 m/s².
Answer-
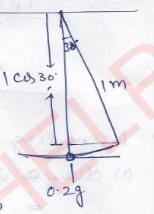
height through which ball is raised
=> 1 – 1 cos 30
=>1 – √3 / 2
=> 2 – 1.732 / 2 = 0.134 m
work done = gain in P.E. = mgh = 0.2 x 10 x 0.134 = 0.268 J
Again,
at lowest point all of its PE is converted into KE
i.e. 1/2 mv² = 0.268
=> v = √0.268 x 2 / m
=> v = √0.268 x 2 / 0.2
=> v = 1.637 m/s
Question-12: A bullet of mass 20 g moving with a speed of 150 m/s strikes a block and comes to rest after piercing 10 cm into it. Find the average force of resistance offered by the block.
Answer- According to work energy theorem
=> ΔK = F x s
=> 1/2 x 20 x 10^-3 x (150)² = F x 10 x 10^-2
=> 10 x 10^-3 x 150 x 150 / 10 x 10^-2 = F
=> F = 2250 N
Question-13: Two identical 5 kg blocks are moving with same speed of 2 metres per second towards each other along a frictionless horizontal surface. The blocks collide, stick together and come to rest. Considering the two blocks as a system, calculate the work done by (i) external forces and (ii) internal forces.
Answer- According to work energy theorem
(i) change in K.E. = work done by external force
=> 1/2 x 5 x 2² + 1/2 x 5 x 2² – 0 = W
=> W = 20 joule
(ii) Work done by internal force is always zero
Question-14 A ball is dropped from rest at a height of 60 m. On striking the ground, it loses 25% of its energy. To what height does it rebound?
Answer- Initial velocity= mgh = m x 60 x g = 60 mg
Energy lost during striking with earth = 25%
∴ energy left = 75% of 60 mg = 45 mg
if new height h’ then
=> mgh’ = 45 mg
=> h’ = 45 m
Question-15: A 10 kg body is dropped from a height of 20 m. What is its potential energy before dropping? What is its kinetic energy when it is 8.0 m high above the ground? What when it hits the ground? (g = 9.8 m/s²)
Answer- Potential energy before dropping
=> mgh = 10 x 9.8 x 20 = 1960 J
again PE at 8 m height
=> 10 x 9.8 x 8 = 784 J
∴ KE at this height = Total energy – PE
=> 1960 – 784 = 1176 J
At the time of lifting the ground all of its PE is converted in KE therefore KE at the time of lifting ground is 1960 J
Question-16: A spring requires 4 J of work to be stretched through 10 cm. Find the spring-constant.
Answer- Work done in stretching a spring = 1/2 kx²
=> 4 = 1/2 k (0.1)²
=> k = 800 N/m
Question-17: A block of mass 2 kg is dropped from a height of 40 cm on a spring whose force-constant is 1960 N/m (figure). What will be the maximum distance x of the compression of the spring? (g=9.8 m/s²)
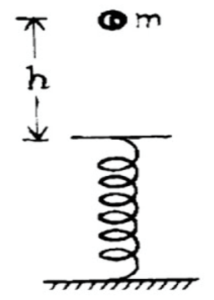
Answer- Total height fallen = h + x+
and compression in spring = x
∴PE of block (loss) = PE of spring (gain)
=> mg(h + x) = 1/2 kx²
=>2 * 9.8 (0.4 + x) = 1/2 1960 * x²
=> 50 x² = 0.4 + x
=> 50 x² – x – 0.4 = 0
=> x = 1 + √1 + 80 / 50 * 2
=> x = 10 cm
Question-18: A solid of mass 2 kg moving with velocity 10 m/s strikes an ideal weightless spring and produces a compression of 25 cm in it. Calculate the force-constant of the spring.
Answer-18 Loss in K.E. of solid = gain in P.E. of spring
=> 1/2 x 2 x (10)² = 1/2 k x (25)²
=> 200 / 0.25 x 0.25 = k
=> k = 3200 N/m
Question-19: A bullet of mass 20 g strikes a block of mass 980 g with a velocity v and is embedded in it. The block is in contact of a spring whose force-constant is 100 N/m. After the collision the spring is compressed up to 10 cm. Find (a) the velocity of the block after the collision, (b) magnitude of the velocity v of the bullet, (c) loss in the kinetic energy due to the collision.
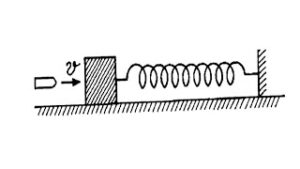
Answer- (a)=> KE of block = PE of spring
=> 1/2 mv² = 1/2 x 100 x (0.1)²
=> mv² = 100 x 0.1
=> (0.980 + 0.020)v² = 1
=> v = 1 m/s
(b)=> Momentum before collision = Momentum after collision
=> 0.02 x v + 0 = (0.98 + 0.02) x 1
=> 0.02 x v = 1 x 1
=> v = 50 m/s
(c)=> loss in energy = 1/2 x 1 x (1)² – 1/2 x 0.02 x (50)²
=> -24.5 J (negative sign shows loss )
Question-20: A smooth sphere (mass 10 kg) rolls on a smooth curved surface from the point A with a speed of 10 m/s. The sphere reaches the point D passing through point B. Find: (a) the total energy of the sphere at the point A (b) the kinetic energy and the potential energy at the point B, (c) the kinetic energy at C, (d) the kinetic energy at D, (e) will the sphere go beyond D? (g = 10 m/s²).
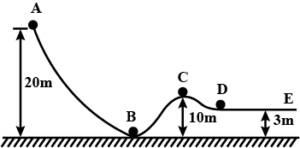
Answer- (a)=> PE at A = mgh = 10 x 10 x 20 = 2000 J
KE at A = 1/2 mv² = 1/2 x 10 x 10² = 500 J
Total energy = 2000 + 500 = 2500 J
(b)=> at point B all of its PE is converted into KE therefore KE at B = 2500 J and PE = 0
(c)=> PE at C = mgh = 10 x 10 x 10 = 1000 J
∴ KE = 2500 – 1000 = 1500 J
(d)=> PE at D = mgh = 10 x 10 x 3 = 300 J
∴ KE at D = 2500 – 300 = 2200 J
(e)=> since it has K.E. , it will go beyond D.
Question-21: A ball is rolling to and fro in a smooth circular cup as shown in the Fig. It goes up on either side of the lowest point A to points 10 cm high above A. What is the speed of the ball at A? (g = 9.8 m/s²)
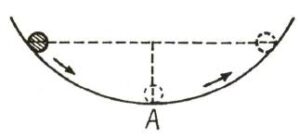
Answer- According to law of conservation of mechanical energy
=> loss in P.E. = gain in K.E.
=> mgh = 1/2 mv²
=> v = √2gh = √2 x 9.8 x 0.1
=> v = 1.4 m/s
Question-22: A smooth body is released from rest A at a point A at the top of a smooth curved track of vertical height 40 cm. What is the speed of the body at the bottom of the curved track? How far along the adjoining smooth inclined plane will the body go?
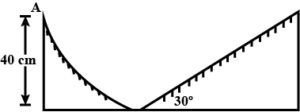
Answer- loss in P.E. = gain in K.E.
=> 1/2 mv² = mgh
=> v = √2gh = √2 x 9.8 x 0.4
=> v = 2.8 m/s
again it will go up to height h = 40 cm
let length of inclined plane l,
then sin 30 = h/l
=> 1/2 = 40/l
=> l = 80 cm
Question-23: An automobile moving at a speed of 72 km/h reaches the foot of a smooth incline when the engines switched off. How much distance does the automobile go up the incline before stopping? The incline makes an angle of 30° with the horizontal.
Answer- K.E. at the foot inclined = 1/2 mv² = 1/2 x m x (72 x 5/18)²
=> 1/2 x m x 400 = 200 m
let it goes up to height h from ground
200 m = mgh
h = 200 /9.8
again length of incline = h / sin 30°
= 200 / 9.8 x 2
= 40.8 m
Question-24: The length of a simple pendulum is 1 m and the mass of the bob is 0.1 kg. The bob is taken towards one side until the thread becomes horizontal and then released. Calculate the kinetic energy of the bob when the thread makes an angle of (i) 0°, (ii) 30° with the vertical.
Answer-
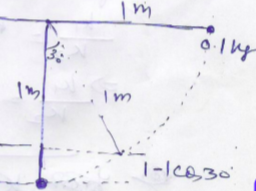
gain in K.E. = loss in P.E.
=> 1/2 mv² = mgh
=> K.E. = 0.1 x 9.8 x 1 = 0.98 J
again when it makes an angle of 30 then height from lowest position = 1 – 1 cos 30°
=> 1 – √3/2
=> (2 – √3) / 2
∴ P.E. = [0.1 x (2 – √3) / 2] x 9.8 => 0.98 (2 – √3) / 2
∴ K.E. = T.E – P.E => 0.98 – 0.98 (2 – √3) / 2 = 0.49 √3 J
Question-25: A 1 kg body falls freely under gravity. Find its momentum and kinetic energy 5 seconds after it starts falling. Take g = 10 m/s².
Answer- mass = 1kg , t = 5 s
v= gt = 10 x 5 = 50 m/s
∴ momentum p = mv
=> 1 x 50 = 50 kg m/s
K.E. = 1/2 mv²
=> 1/2 x 1 x (50)² = 1250 J
Question-26: If the momentum of a body is increased by 50%, what will be the percentage increase in the kinetic energy of the body?
Answer- momentum and Kinetic energy related as
=> K = p² / 2m
=> K1 / K2 = (P1)² / (P2)²
Let p1 = 100 then P2 = 150
K1 / K2 = (100 / 150)² = (100 / 225)
=> % increase in K.E. = 225 – 100 = 125%
Question-27: Find the ratio of kinetic energies of two bodies of masses 1 kg and 4 kg having equal linear momenta.
Answer- K = p² / 2m
for equal momentum k ∝ 1/m
=> k1 / k2 = m2 / m1
=> k1 / k2 = 4 /1 => 4 : 1
Question-28: Find the ratio of linear momenta of two bodies of masses 1 kg and 4 kg having equal kinetic energies.
Answer- from relation — K = p² / 2m
=> p = √2mK
for same K.E.
=> p1 / p2 = √(m1/m2) = √(1/4) = 1 : 2
—: end of Energy and Its Conservation Numerical Class 11 Nootan ISC Solutions Ch-9 Work Energy Power :—
Return to : – Nootan Solutions for ISC Physics Class-11 Nageen Prakashan
Thanks
Please Share with your friends if helpful