Flow of Liquids Numerical on Bernoulli’s Theorem and Velocity of Efflux Class-11 Nootan ISC Physics Solutions Ch-15. Step by step Solutions of Kumar and Mittal Physics of Nageen Prakashan as council latest prescribe guideline for upcoming exam. Visit official Website CISCE for detail information about ISC Board Class-11 Physics.
Flow of Liquids Numerical on Bernoulli’s Theorem and Velocity of Efflux Class-11 Nootan ISC Physics
| Board | ISC |
| Class | 11 |
| Subject | Physics |
| Writer | Kumar and Mittal |
| Publication | Nageen Prakashan |
| Chapter-15 | Flow of Liquids |
| Topics | Numericals on Principle of Continuity, Bernoulli’s Theorem and Velocity of Efflux |
| Academic Session | 2024-2025 |
Numericals on Principle of Continuity, Bernoulli’s Theorem and Velocity of Efflux
Flow of Liquids Class-11 Nootan ISC Solutions Ch-15 Solutions of Kumar and Mittal Physics , Nageen Prakashan
Que-1: Velocity of flow of water in a horizontal pipe is 10.0 m/s. Find the velocity-head of water. (g = 10.00 m s²)
Ans- velocity head = v² / 2g
=> (10 x 10) / (2 x 10)
=> 5.00 m
Que-2: Water is flowing through a cylindrical pipe of cross-sectional area 0.09 π m² at a speed of 1.0.m/s. If the diameter of the pipe is halved, then find the speed of flow of water through it.
Ans-. A1 = 0.09π = πr²
=> r1 = 0.3 , r2 = 0.3/2 = 0.15 m
According to principle of continuity
=> A1 V1 = A2 V2
=>π r1² v1 = π r2² v2
=> r1² v1 = r2² v2
=> (0.3)² x 1 = (0.15)² x v2
=> v2 = (0.3 / 0.15)² x 1
=> v2 = 4 m/s
Que-3: Water is flowing through a horizontal tube of non-uniform cross-section. At a place the radius of the tube is 0.5 cm and the velocity of water there is 20 cm/s. What will be the velocity at another place where the radius of the tube is 1.0 cm?
Ans-. According to principle of continuity
=> A1 V1 = A2 V2
=>π r1² v1 = π r2² v2
=> r1² v1 = r2² v2
=> (0.5)² x 20 = (1)² x v2
=> v2 = 20 x 0.25
=> v2 = 5 cm/s
Que-4: Water is flowing through a horizontal pipe of non-uniform cross-section. The speed of water is 30 cm/s at a place where pressure is 10 cm (of water). Calculate the speed of water at the other place where the pressure is half of that at the first place.
Ans-. According to Bernoulli’s equation
=> P1 + 1/2 ρ v1² = P2 + 1/2 ρ v2²
=> P1 – P2 = 1/2 ρ v2² – 1/2 ρ v1²
=> ρ g (h1 – h2) = 1/2 ρ (v2² – v1²)
=> 2 g (h1 – h2) = (v2² – v1²)
=> 2 x 9.8 (0.1 – 0.05) = v2² – (0.3)²
=> 2 x 9.8 x 0.05 + 0.09 = v2²
=> 1.07 = v2²
=> v2 = √1.07
=> v2 = 1.034 m/s = 103.4 m/s
Que-5: Water enters a horizontal pipe of non-uniform cross-section with a velocity of 0.5 m/s and leaves the other end with a velocity of 0.7 m/s. The pressure of water at the first end is 10³ N/m². Calculate pressure at the other end. (Density of water = 1.0 × 10³ kg/m³.)
Ans-According to Bernoulli’s equation
=> P1 + 1/2 ρ v1² = P2 + 1/2 ρ v2²
=> P1 – P2 = 1/2 ρ v2² – 1/2 ρ v1²
=> 1000 – P2 = 1/2 1000 (0.7² – 0.5²)
=> 1000 – P2 = 500 (0.7 + 0.5) (0.7 – 0.5)
=> 1000 – P2 = 500 x 0.12 x 0.2 = 120
=> P2 = 1000 – 120
=> P2 = 880 pascal
Que-6: Water flows through a horizontal pipe of varying cross-section. The pressure of water equals to 0.1 m of mercury at a place where the velocity of flow is 0.4 m/s. What will be the pressure at another place where the velocity of flow is 0.5 m/s?
Ans- P1 – P2 = 1/2 ρ v2² – 1/2 ρ v1²
=> (0.1 – h2) x 13.6 x 10³ x 9.8 = 1/2 x 10³(0.5² – 0.4²)
=> (0.1 – h2) x 133280 = 500 x 0.9
=> h2 = 0.1 – (500 x 0.09 / 133280)
=> h2 = 0.99966 m of Hg
Que-7: Water is flowing through a horizontal pipe of varying cross-section. At any two places, the diameters of the tube are 4 cm and 2 cm. If the pressure difference between these two places be equal to 4.5 cm (water), then determine the rate of flow of water in the tube.
Ans-7 Q = A1A2 √(2gh / A1² – A2²)
=> 4 x π x π √(2 x 980 x 4.5 / 16π² – π²)
=> 4 π √(2 x 980 x 4.5 / 10 x 15)
=> 4 π √ 588
=> 304.72 cm³/s
Que-8: Water is flowing steadily through a horizontal pipe of non-uniform cross-section. At a point, where the cross-sectional area of the pipe is 0.02 m², the pressure of water is 4.0 × 10^4 N/m² (or Pa) and its velocity of flow is 2.0 m/s. What is the pressure at a point where the cross-sectional area is 0.01 m² ? The density of water is 10³ kg/m³.
Ans- According to Bernoulli’s equation
=> P1 + 1/2 ρ v1² = P2 + 1/2 ρ v2²
According to principle of continuity
=> A1 V1 = A2 V2
=> 0.02 x 2 = 0.1 x V2
=> V2 = 4 m/s
again
=> P1 – P2 = 1/2 ρ v2² – 1/2 ρ v1²
=> 4 x 10^4 – P2 = 1/2 x 10³ (4² – 2²)
=> 4 x 10^4 – P2 = 500 (12)
=> P2 = 4 x 10^4 – 0.6 x 10^4
=> P2 = 3.4 x 10^4 Pa
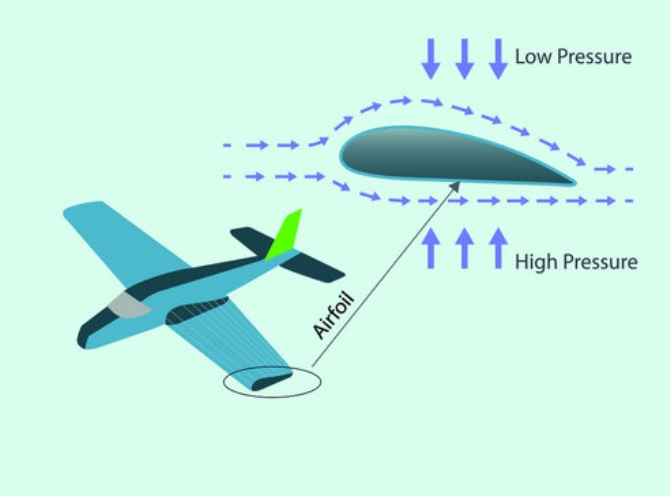
Que-9: Air is streaming past a horizontal airplane wing such that its speed is 120 m/s at the upper surface and 90 m/s at the lower surface. If the density of air is 1.3 kg/m³, find the difference in pressures between the two surfaces of the wing. If the wing is 10 m long and has an average width of 2 m, then calculate the gross lift on it.
Ans- Δp = 1/2 ρ (v2² – v1²)
=> 1/2 x 1.3 x (120² – 90²)
=> 1.3/2 x (120-90) (120 + 90)
=> 1.3/2 x 30 x 210 = 4095 Pa
and force = Δp x A
=> 4095 x 10 x 2
=> 8.19 x 10^4 N
Que-10: A water tank has a hole in its wall at a distance of 40 m below the free surface of water. Compute the velocity of efflux of water from the hole. If the radius of the hole be 1 mm, find the rate of flow of water.
Ans- v = √2gh = √ 2 x 9.8 x 40 = 28 m/s
rate of flow of water = Av = (π x 1.0 x 10^-3)² x 28
=> 8.8 x 10^-5 m³/s
—: end of Flow of Liquids Numerical on Bernoulli’s Theorem and Velocity of Efflux Class-11 Nootan ISC Solutions :—
Return to : – Nootan Solutions for ISC Physics Class-11 Nageen Prakashan
Thanks
Please Share with your friends if helpful