ML Aggarwal Linear Inequation Exe-4 Class 10 ICSE Maths Solutions . We Provide Step by Step Answer of Exe-4 Questions for Linear Inequation as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-10.
ML Aggarwal Exe-4 Linear Inequation Class 10 ICSE Maths Solutions
| Board | ICSE |
| Subject | Maths |
| Class | 9th |
| Chapter-4 | Linear Inequation |
| Writer / Book | Understanding |
| Topics | Solutions of Exe-4 |
| Academic Session | 2024-2025 |
ML Aggarwal Linear Inequation Exe-4 Class 10 ICSE Maths Solutions
Question -1. Solve the inequation 3x -11 < 3 where x ∈ {1, 2, 3,……, 10}. Also represent its solution on a number line
Answer :
3x – 11 < 3 => 3x < 3 + 11 => 3x < 14 x < (14/3)
But x ∈ 6 {1, 2, 3, ……., 10}
Solution set is (1, 2, 3, 4}.
Solution set on number line
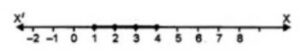
Question- 2. Solve 2(x – 3)< 1, x ∈ {1, 2, 3, …. 10}
Answer :
2(x – 3) < 1 => x – 3 < 1/2 => x < 1/2 + 3 => x < 3(1/2)
But x ∈ {1, 2, 3 …..10}
Solution set = {1, 2, 3}
![]()
Question -3. Solve : 5 – 4x > 2 – 3x, x ∈ W. Also represent its solution on the number line.
Answer:
5 – 4x > 2 – 3x
– 4x + 3x > 2 – 5
=> – x > – 3
=> x < 3
x ∈ w,
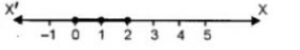
solution set {0, 1, 2}
Solution set on number line:
Question- 4. List the solution set of 30 – 4 (2.x – 1) < 30, given that x is a positive integer.
Answer :
30 – 4 (2x – 1) < 30
30 – 8x + 4 < 30
– 8x < 30 – 30 – 4
– 8x < – 4 x > (-4/-8)
=> x > 1/2
x is a positive integer
x = {1, 2, 3, 4…..} Ans.
Question -5. Solve : 2 (x – 2) < 3x – 2, x ∈ { – 3, – 2, – 1, 0, 1, 2, 3} .
Answer:
2(x – 2) < 3x – 2
=> 2x – 4 < 3x – 2
=> 2x – 3x < – 2 + 4
=> – x < 2
=> x > – 2
Solution set = { – 1, 0, 1, 2, 3} Ans.
Question -6. If x is a negative integer, find the solution set of 2/3+1/3 (x + 1) > 0.
Answer :
2/3+1/3 x + 1/3 > 0
=> 1/3 x + 1 > 0
=> 1/3 x > – 1
⇒ x > – 1 × 3/1 ⇒ x > – 3
x is a negative integer
Solution set = {- 2, – 1} Ans.
Question -7. Solve x – 3 (2 + x) > 2 (3x – 1), x ∈ { – 3, – 2, – 1, 0, 1, 2, 3}. Also represent its solution on the number line.
Answer :
x – 3 (2 + x) > 2 (3x – 1)
=> x – 6 – 3x > 6x – 2
=> x – 3x – 6x > – 2 + 6
=> – 8x > 4
=> x < -4/8 => x < -1/2
x ∈ { – 3, – 2, – 1, 0, 1, 2}
.’. Solution set = { – 3, – 2, – 1}
Solution set on Number line:

Question- 8. Given x ∈ {1, 2, 3, 4, 5, 6, 7, 9} solve x – 3 < 2x – 1.
Answer:
x – 3 < 2x – 1
x – 2x < – 1 + 3 => – x < 2 x > – 2
But x ∈ {1, 2, 3, 4, 5, 6, 7, 9}
Solution set = {1, 2, 3, 4, 5, 6, 7, 9} Ans.
Question -9. List the solution set of the inequation
1/2 + 8x > 5x -3/2, x ∈ Z
Answer :
1/2 +8x > 5x -3/2
⇒ 8x – 5x > – 3/2 – 1/2
⇒ 3x > – 2 ⇒ x > – 2/3
∵ x ∈ Z,
∴ Solution set = {0, 1, 2, 3, 4 ,…….}
Question -10. List the solution set of 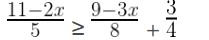
x ∈ N
Answer:
![]()
=> 88 – 16x ≥ 45 – 15x + 30
(L.C.M. of 8, 5, 4 = 40}
=> – 16x + 15x ≥ 45 + 30 – 88
=> – x ≥ – 13
=>x ≤ 13
x ≤ N.
Solution set = {1, 2, 3, 4, 5, .. , 13} Ans.
Question -11. Find the values of x, which satisfy the inequation : 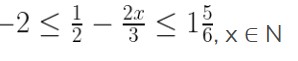
x ∈ N.
Graph the solution set on the number line.
Answer :
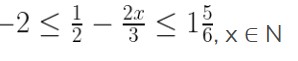 , x ∈ N
, x ∈ N
⇒ – 2 – 1/2 ≤ 1/2 – 2x/3 – 1/2 ≤ 11/6 – 1/2
[By subtracting 1/2 on both sides of inequality]
⇒ – 5/2 ≤ 2x/3 ≤ 8/6
⇒ – 15 ≤ – 4x ≤ 8
⇒ 15 ≥ 4x ≥ – 8
⇒ 15/4 ≥ x ≥ – 2
3.3/4 ≥ x ≥ – 2
But x ∈ N, hence only possible solution for x = {1, 2, 3}
Question -12. If x ∈ W, find the solution set of
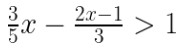
Also graph the solution set on the number line, if possible.
Answer :
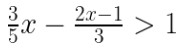
9x – (10x – 5) > 15 (L.C.M. of 5, 3 = 15)
=> 9x – 10x + 5 > 15
=> – x > 15 – 5
=> – x > 10
=> x < – 10
But x ∈ W
Solution set = Φ
Hence it can’t be represented on number line.
Question-13. Solve:
where x is a positive odd integer.
(ii) (2x + 3)/3 ≥ (3x – 1)/4 where x is positive even integer.
Answer :
⇒ x/2 – x/3, ≤ 6 – 5
⇒ (3x – 2x)/6 ≤ 1
⇒ x/6 ≤ 1
⇒ x ≤ 6
∵ x is a positive odd integer
∴ x = {1, 3, 5}
(ii) (2x + 3)/3 ≥ (3x – 1)/4
⇒ 2x/3 + 3/3 ≥ 3x/4 – 1/4
⇒ 2x/3 – 3x/4 ≥ -1/4 – 1
⇒ (8x – 9x)/12 ≥ – 5/4
⇒ -x/12 ≥ -5/4
⇒ x/12 ≤ 5/4
⇒ x ≤ 5/4 × 12
⇒ x ≤ 15
∵ x is positive even integer
∴ x = {2, 4, 6, 8, 10, 12, 14}
Question -14. Given that x ∈ I, solve the inequation and graph the solution on the number line :
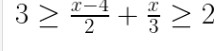
Answer :
3 ≥ (3x – 12 + 2x)/6
⇒ 3 ≥ (5x – 12)/6
⇒ 18 ≥ 5x – 12
⇒ 5x – 12 ≤ 18
⇒ 5x ≤ 18 + 12
⇒ 5x ≤ 30
⇒ x ≤ 6
Question -15. Solve : 1 ≥ 15 – 7x > 2x – 27, x ∈ N
Answer :
1 ≥ 15 – 7x > 2x – 27
1 ≥ 15 – 7x and 15 – 7x > 2x – 27
⇒ 7x ≥ 15 – 1 and – 7x – 2x > – 27 – 15
⇒ 7x ≥ 14 and – 9x > – 42
⇒ x ≥2 and – x > – 42/9
⇒ 2 ≤ x and – x > – 14/3 and x < 14/3
2 ≤ x < 14/3
But x ∈ N
∴ Solution set = {2, 3, 4}
Question-16. If x ∈ Z, solve 2 + 4x < 2x – 5 ≤ 3x. Also represent its solution on the number line.
Answer:
2 + 4x < 2x – 5 ≤ 3x
2 + 4x < 2x – 5 and 2x – 5 ≤ 3x
⇒ 4x – 2x < -5 – 2, and 2x – 3x ≤ 5
⇒ 2x < – 7 and – x ≤ 5
⇒ x < – (7/2) and x ≥ – 5 and – 5 ≤ x
∴ – 5 ≤ x < -(7/2)
∵ x ∈ Z
∴ Solution set = {- 5, -4}
Solution set on Number line
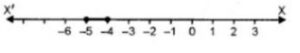
Question -17. Solve : (4x-10)/3 ≤ (5x-7)/2 x ∈ R and represent the solution set on the number line.
Answer :
(4x – 10)/3 ≤ (5x – 7)/2
⇒ 8x – 20 ≤ 15x – 21
(L.C.M. of 3, 2 = 6)
⇒ 8x – 15x ≤ – 21 + 20
⇒ – 7x ≤ – 1 ⇒ – x ≤ – (1/7)
⇒ x > 1/7
∵ x ∈ R
∴ Solution set = {x : x ∈ R, x > 1/7}
Solution set on the number line

Question -18
Solve 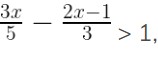
x ∈ R and represent the solution set on the number line.
Answer -18
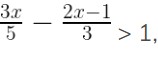
=> 9x – (10x – 5) > 15
=> 9x – 10x + 5 > 15
=> – x > 15 – 5
=> – x > 10
=> x < – 10
x ∈ R.
.’. Solution set = {x : x ∈R, x < – 10}
Solution set on the number line
![]()
ML Aggarwal Linear Inequation Exe-4 Class 10 ICSE Maths Solutions
page-53
Question -19. Given that x ∈ R, solve the following inequation and graph the solution on the number line: – 1 ≤ 3 + 4x < 23. (2006)
Answer :
We have
– 1 ≤ 3 + 4x < 23 => – 1 – 3 ≤ 4x < 23 – 3 => – 4 ≤ 4x < 20 => – 1 ≤ x < 5, x ∈ R
Solution Set = { – 1 ≤ x < 5; x ∈ R}
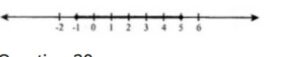
Question-20. Solve the following inequation and graph the solution on the number line. (2007)
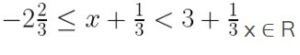
Answer :
Given
Given,
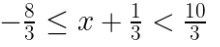
Multiplying by 3, L.C.M. of fractions, we get
– 8 ≤ 3x + 1 < 10
– 8 – 1 ≤ 3x + 1 – 1 < 10 – 1 [Add – 1]
– 9 ≤ 3x < 9
– 3 ≤ x < 3 [Dividing by 3]
Hence, the solution set is {x : x ∈ R, – 3 ≤ x < 3}
Question-21. Solve the following inequation and represent the solution set on the number line :
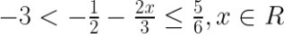
Answer:
![]()
(i) – 3 < – 1/2 – 2x/3
⇒ – 3 < (1/2 + 2x/3
⇒ – (1/2 + 2x/3) > – 3
⇒ – 2x/3 > – 3 + 1/2
⇒ – 2x/3 > -5/2
⇒ 2x/3 < 5/2
⇒ x < 5/2 × 3/2
⇒ x < 15/4
or
⇒ – (2x/3) ≤ 5/6 + 1/2
⇒ -2x/3 ≤ (5 + 3)/6
⇒ -2/3.x ≤ 8/6
⇒ 2/3.x ≥ -8/6
⇒ x ≥ – 8/6 ×3/2
⇒ x ≥ – 2
⇒ – 2 ≤ x …(ii)
From (i) and (ii),
– 2 ≤ x ≤ 15/4
∴ Solution = {x : x ∈ R, – 2 ≤ x < 15/4}
Now solution on number line
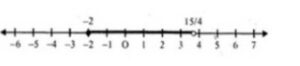
Question -22. Solving the following inequation, write the solution set and represent it on the number line. – 3(x – 7)≥15 – 7x > (x+1)/3, n ∈R
Answer :
– 3(x – 7)≥15 – 7x > (x+1)/3, n ∈R
⇒ -3(x – 7) ≥ 15 – 7x ⇒ – 3x + 21 ≥ 15 – 7x
⇒ – 3x + 7x ≥ 15 – 21 ⇒ 4x ≥ – 6
⇒ x ≥ -6/4
⇒ x ≥ -3/2
⇒ -3/2 ≤ x
And 15 – 7x > (x + 1)/3
⇒ 45 – 21x > x + 1
⇒ 45 – 1 > x + 21x
⇒ 44 > 22x
2 > x ⇒ x = 2
∴ -3/2 ≤ x < 2, x ∈ R
Question -23. Solving the following inequation , write the solution set and represent it on the real number line. -2+10x ≤13x +10<24+10x, x ∈ Z.
Answer:
(a) Given that :
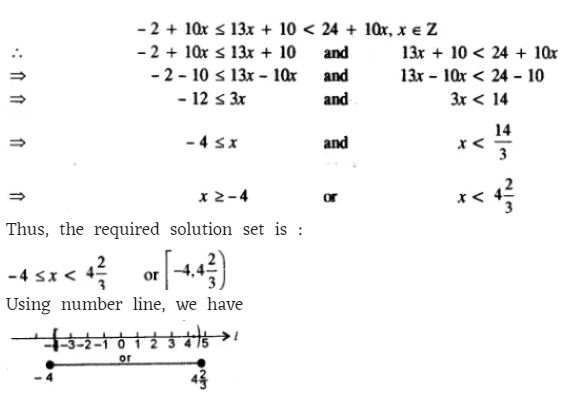
Question -24. Solve the inequation 2x – 5 ≤ 5x + 4 < 11, where x ∈ I. Also represent the solution set on the number line. (2011)
Answer :
2x – 5 ≤ 5x + 4 < 11 2x – 5 ≤ 5x + 4
=> 2x – 5 – 4 ≤ 5x and 5x + 4 < 11
=> 2x – 9 ≤ 5x and 5x < 11 – 4
and 5x < 7
=> 2x – 5x ≤ 9 and x <
=> 3x > – 9 and x< 1.4
=> x > – 3
Question-25. If x ∈ I, A is the solution set of 2 (x – 1) < 3 x – 1 and B is the solution set of 4x – 3 ≤ 8 + x, find A ∩B.
Answer:
2 (x – 1) < 3 x – 1
2x – 2 < 3x – 1
2x – 3x < – 1 + 2 => – x < 1 x > – 1
Solution set A = {0, 1, 2, 3, ..,.}
4x – 3 ≤ 8 + x
4x – x ≤ 8 + 3
=> 3x ≤ 11
=> x ≤ 11/3
Solution set B = {3, 2, 1, 0, – 1…}
A ∩ B = {0, 1, 2, 3} Ans.
Question -26. If P is the solution set of – 3x + 4 < 2x – 3, x ∈ N and Q is the solution set of 4x – 5 < 12, x ∈ W, find
(i) P ∩ Q
(ii) Q – P.
Answer:
(i) – 3 x + 4 < 2 x – 3
– 3x – 2x < – 3 – 4
=> – 5x < – 7
– x < – 7/5
⇒ x > 7/5
∴ Solution set P = {2, 3, 4, 5, …….}
4x – 5 < 12
4x < 12 + 5 ⇒ 4x < 17
x < 17/4
∵ x ∈ W
∴ Solution set Q = {4, 3, 2, 1, 0}
(i) P ∩ Q = {2, 3, 4}
(ii) Q – P = {1, 0}
Question -27. A = {x : 11x – 5 > 7x + 3, x ∈R} and B = {x : 18x – 9 ≥ 15 + 12x, x ∈R}
Find the range of set A ∩ B and represent it on a number line
Answer :
A = {x : 11x – 5 > 7x + 3, x ∈R}
B = {x : 18x – 9 ≥ 15 + 12x, x ∈R}
Now, A = 11x – 5 > 7x + 3
=> 11x – 7x > 3 + 5
=> 4x > 8
=>x > 2, x ∈ R
B = 18x – 9 ≥ 15 + 12x
⇒ 18x – 12x ≥ 15 + 9
⇒ 6x ≥ 24
⇒ x ≥ 4
∴ A ∩ B = x ≥ 4, x ∈ R
Hence Range of A ∩ B = {x : x ≥ 4, x ∈ R} and its graph will be.
Question -28.
Given: P {x : 5 < 2x – 1 ≤ 11, x∈R)
Q{x : – 1 ≤ 3 + 4x < 23, x∈I) where
R = (real numbers), I = (integers)
Represent P and Q on number line. Write down the elements of P ∩ Q. (1996)
Answer :
P= {x : 5 < 2x – 1 ≤ 11}
5 < 2x – 1 ≤ 11
⇒ 5 < 2x – 1 and 2x – 1 ≤ 11
⇒ – 2x < – 5 – 1 and 2x ≤ 11 + 1
⇒ – 2x < – 6 and 2x ≤12
⇒ – x < – 3 and x ≤ 6
⇒ x > 3 or 3 < x
∴ Solution set = 3 < x ≤ 6 = {4, 5, 6}
Solution set on number line.
Q = {-1 ≤ 3 + 4x < 23}
– 1 ≤ 3 + 4x < 23
⇒ – 1 < 3 + 4x and 3 + 4x < 23
⇒ – 4x < 3 + 1 4x < 23 – 3
⇒ – 4x < 4 4x < 20
⇒ – x < 1 x < 5
⇒ x > – 1
– 1 < x
∴ – 1 < x < 5
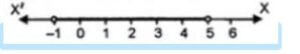
∴ Solution set = {1, 1, 2, 3, 4}
Solution set on number line
Question -29. If x ∈ I, find the smallest value of x which satisfies the inequation 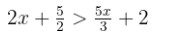
Answer :
![]()
=> ![]()
=>12x – 10x > 12 – 15
=> 2x > – 3
=> x > -3/2
Smallest value of x = – 1 Ans.
Question- 30. Given 20 – 5 x < 5 (x + 8), find the smallest value of x, when.
(i) x ∈ I
(ii) x ∈ W
(iii) x ∈ N.
Answer :
20 – 5 x < 5 (x + 8)
⇒ 20 – 5x < 5x + 40
⇒ – 5x – 5x < 40 – 20
⇒ – 10x < 20
⇒ – x < 2
⇒ x > – 2
(i) When x ∈ I, then smallest value = – 1.
(ii) When x ∈ W, then smallest value = 0.
(iii) When x ∈ N, then smallest value = 1. Ans.
Question- 31. Solve the following inequation and represent the solution set on the number line :
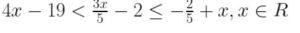
Answer :
We have
![]()
Hence, solution set is {x : -4 < x < 5, x ∈ R}
The solution set is represented on the number line as below.
⇒ 4x – 19 < 3x/5 – 2 and 3x/5 – 2 ≤ -2/5 + x, x ∈ R
⇒ 4x – 3x/5 < 17 and – 2 + 2/5 ≤ x – 3x/5, x ∈ R
⇒ 17x/5 < 17 and -8/5 ≤ 2x/5, x ∈ R
⇒ x < 5 and – 4 ≤ x, x ∈ R
⇒ – 4 ≤ x < 5, x ∈ R
Hence, solution set is {x : 4 ≤ x < 5, x ∈ R}
The solution set is represented on the number line as below.
![]()
Question- 32. Solve the given inequation and graph the solution on the number line :
2y – 3 < y + 1 ≤ 4y + 7; y ∈ R.
Answer :
2y – 3 < y + 1 ≤ 4y + 7; y ∈ R.
(a) 2y – 3 < y + 1
⇒ 2y – y < 1 + 3
⇒ y < 4
⇒ 4 > y ….(i)
(b) y + 1 ≤ 4y + 7
⇒ y – 4y ≤ 7 – 1
⇒ 3y ≤ 6
⇒ y ≤ 6/-3
⇒ y ≥ – 2 ….(ii)
From (i) and (ii),
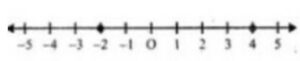
4 > y ≥ – 2 or – 2 ≤ y < 4
Now representing it on a number given below
Question -33. Solve the inequation and represent the solution set on the number line.
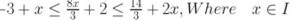
Answer -33
Given : ![]()
(i) – 3 + x ≤ 8x/3 + 2
⇒ – 3 – 2 ≤ 8x/3 – x
⇒ – 5 ≤ 5x/3
⇒ – 1 ≤ x/3
⇒ – 3 ≤ x ….(i)
And 8x/3 = 2 ≤ 14/3 + 2x
8x/3 – 2x ≤ 14/3 – 2
⇒ 2x/3 ≤ 8/3
⇒ x ≤ 4 ….(ii)
From (i) and (ii)
⇒ – 5 ≤ 5x/3 and 2x/3 ≤ 8/3
⇒ x ≥ – 3 and x ≤ 4
∴ – 3 ≤ x ≤ 4
Solution set = {-3, -2, -1, 0, 1, 2, 3, 4}
Solution set on number line
Question -34. Find the greatest integer which is such that if 7 is added to its double, the resulting number becomes greater than three times the integer.
Answer :
Let the greatest integer = x
According to the condition,
2x + 7 > 3x
⇒ 2x – 3x > – 7
⇒ – x > – 7
⇒ x < 7
Value of x which is greatest = 6 Ans.
Question -35. One-third of a bamboo pole is buried in mud, one-sixth of it is in water and the part above the water is greater than or equal to 3 metres. Find the length of the shortest pole.
Answer :
Let the length of the shortest pole = x metre
Length of pole which is buried in mud = x/3
Length of pole which is in the water = x/6
According to this problem,
x – [x/3 + x/6] ≥ 3
⇒ x – (2x + x)/6 ≥ 3
⇒ x – x/2 ≥ 3
⇒ x/2 ≥ 3
⇒ x ≥ 6
∴ Length of pole (shortest in length) = 6 metres
— : End of ML Aggarwal Linear Inequation Exe-4 Class 10 ICSE Maths Solutions :–
Return to :- ML Aggarwal Solutions for ICSE Class-10
Thanks
Please Share with Your Friends



Many images aren’t available especially those of number line.
it is visible now
when will soulution of new book of session 24 25 solution will come
very soon