ML Aggarwal Linear Equations and Inequalities in One Variable MCQs Class 8 ICSE Ch-12 Maths Solutions. We Provide Step by Step Answer of MCQs Questions for Linear Equations and Inequalities in One Variable as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-8.
ML Aggarwal Linear Equations and Inequalities in One Variable MCQs Class 8 ICSE Maths Solutions
| Board | ICSE |
| Publications | Avichal Publishig Company (APC) |
| Subject | Maths |
| Class | 8th |
| Chapter-12 | Linear Equations and Inequalities in One Variable |
| Writer | ML Aggarwal |
| Book Name | Understanding |
| Topics | Solution of MCQs |
| Edition | 2023-2024 |
Linear Equations and Inequalities in One Variable MCQs
ML Aggarwal Class 8 ICSE Maths Solutions
Page-219
Mental Maths
Question 1. Fill in the blanks:
(i) An equation of the type ax + b = 0 where a ≠ 0 is called a …………. in variable x.
(ii) Any value of the variable which satisfies the equation is called a …………. of the equation.
(iii) The process of finding all the solutions of an equation is called ………….
(iv) We can add the …………. to both sides of an equation.
(v) We can divide both sides of an equation by the same …………. number.
(vi) The solution set of the inequality 3x ≤ 10, x ϵ N is ………….
Answer:
(i) An equation of the type ax + b = 0 where a ≠ 0 is called a linear equation in variable x.
(ii) Any value of the variable which satisfies the equation is called a solution of the equation.
(iii) The process of finding all the solutions of an equation is called solving the equation.
(iv) We can add the same number to both sides of an equation.
(v) We can divide both sides of an equation by the same non-zero number.
(vi) The solution set of the inequality 3x ≤ 10, x ϵ N is (1, 2, 3).
Question 2. State whether the following statements are true (T) or false (F):
(i) An equation is a statement that two expressions are equal.
(ii) We cannot subtract the same number from both sides of an equation.
(iii) 3x + 2 = 4(x + 7) + 9 is a linear equation in variable x.
(iv) x = 1 is the solution of equation 4(x + 5) = 24.
Answer:
(i) An equation is a statement that two expressions are equal. True
(ii) We cannot subtract the same number from both sides of an equation. False
(iii) 3x + 2 = 4(x + 7) + 9 is a linear equation in variable x. True
(iv) x = 1 is the solution of equation 4(x + 5) = 24. True
Linear Equations and Inequalities in One Variable MCQs
ML Aggarwal Class 8 ICSE Maths Solutions
Page-220
Choose the correct answer from the given four options (3 to 16):
Question 3. Which of the following is not a linear equation in one variable?
(a) 3x + 2 = 0
(b) 2y – 4 = y
(c) x + 2y = 7
(d) 2(x – 3) + 7 = 0
Answer:
x + 2y = 7 is not a linear equation in one variable
as there are two variables x and y. (c)
Question 4. The solution of the equation 2/3x + 1 = 15/9 is
(a) 1
(b) 3/2
(c) 2
(d) 2/3
Answer:
(a) 1
Question 5. The solution of the equation 4z + 3 = 6 + 2z is
(a) 1
(b) 3/2
(c) 2
(d) 3
Answer:
Solution of equation 4z + 3 = 6 + 2z
⇒ 4z – 2z = 6 – 3 ⇒ 2z = 3 ⇒ z = 3/2 (b)
(ML Aggarwal Linear Equations and Inequalities in One Variable MCQs Class 8)
Question 6. The solution of the equation 3x/5 + 1 = 4x/15 is +7 is
(a) 12
(b) 14
(c) 16
(d) 18
Answer:
(d) 18
Question 7. The solution of the equation x/2 – 1/5 = x/3 + 1/4 is
(a) 2.7
(b) 1.8
(c) 2.9
(d) 1.7
Answer:
(a) 2.7
Question 8. The solution of the equation (8x – 3)/3x = 2 is
(a) 1/2
(b) 2/3
(c) 3/2
(d) 1/3
Answer:
(c) 3/2
Question 9. If we subtract 1/2 from a number and multiply the result by 1/2, we get 1/8, then the number is
(a) 1/2
(b) 3/4
(c) 1/4
(d) none of these.
Answer:
(b) 3/4
Question 10. Fifteen years from now Ravi’s age will be four times his present age. What is Ravi’s present age?
(a) 4 years
(b) 5 years
(c) 6 years
(d) 3 years
Answer:
Let present age of Ravi = x years
After 15 years, his age will be = (x + 5) years
∴ x + 15 = 4x
⇒ 15 = 4x – x = 3x
⇒ x = 15/3 = 5
∴ His present age = 5 years (b)
Question 11. If the sum of three consecutive integers is 51, then the largest integer is
(a) 16
(b) 17
(c) 18
(d) 19
Answer:
Let first integers = x
Then next two integers = x + 1, x + 2
∴ x + x + 1 + x + 2 = 51
⇒ 3x + 3 = 51
⇒ 3x = 51 – 3 = 48
⇒ x = 48/3 = 16
∴ First integer = 16
and other two integer = 17, 18
Largest integers =18 (c)
Question 12. If the perimeter of a rectangle is 13 cm and its Width is 2(3/4) cm, then its length is
(a) 2(3/4) cm
(b) 3(3/4) cm
(c) 4(3/4) cm
(d) 5(3/4) cm
Answer:
(b) 3(3/4) cm
(ML Aggarwal Linear Equations and Inequalities in One Variable MCQs Class 8)
Question 13. What should be added to twice the rational number -7/3 to get 3/7 ?
(a) 58/21
(b) 29/21
(c) 89/21
(d) 107/21
Answer:
(d) 107/21
Question 14. Sum of digits of a two digit number is 8. If the number obtained by reversing the digits is 18 more than the original number, then the original number is
(a) 35
(b) 53
(c) 26
(d) 62
Answer:
Sum of digits of a two digit number = 8
Let unit digit = x
Then tens digit = 8 – x
∴ Number = x + 10(8 – x) = x + 80 – 10x = 80 – 9x
By reversing the digits,
Unit digit = 8 – x
and tens digit = x
∴ Number = 8 – x + 10x = 8 + 9x
∴ 8 + 9x = 80 – 9x + 18
⇒ 9x + 9x = 80 + 18 – 8
⇒ 18x = 90
⇒ x = 90/18 =5
∴ Number = 80 – 9x = 80 – 9 × 5 = 80 – 45 = 35 (a)
Question 15. Arjun is twice as old as Shriya. If five years ago his age was three times Shriya’s age, then Arjun’s present age is
(a) 10 years
(b) 15 years
(c) 20 years
(d) 25 years
Answer:
Let Shriya’s age = x years
Then Arjun’s age = 2x
5 years ago,
Age of Shriya was = (x – 5) years
and age of Arjun’s = (2x – 5) years
∴ 2x – 5 = 3(x – 5)
⇒ 2x – 5 = 3x – 15
⇒ 3x – 2x = 15 – 5 = 10
⇒ x = 10
∴ Arjun’s present age = 2x = 2 × 10 = 20 years (c)
Question 16. If the replacement set is {-5, -3, -1,0, 1, 3}, then the solution set of the inequation -3 < x < 3 is
(a) {-2,-1, 0, 1, 2}
(b) {-1, 0, 1, 2}
(c) {-3,-1, 0, 1, 3}
(d) {-1,0, 1}
Answer:
Replacement set = {-5, -3, -1, 0, 1,3}
-3 < x < 3
∴ x = {-2, -1, 0, 1, 2} from the replacement set,
Solution set x = {-1, 0, 1} (d)
Linear Equations and Inequalities in One Variable HOTS
ML Aggarwal Class 8 ICSE Maths Solutions
Page-220
Question 1. A man covers a distance of 24 km in 3(1/2) hours partly on foot at the speed of 4.5 km/h and partly on bicycle at the speed of 10 km/h. Find the distance covered on foot.
Answer
Total distance = 24 km
Time taken = 3(1/2) hours = 7/2 hours
Let a man travels x km on foot at the speed of 4.5 km
and (24 – x) km on bicycle at the speed of 10 km/hr
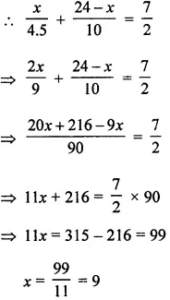
∴ He travelled 9 km on foot.
Question 2. A person preparing a medicine wants to convert 15% alcohol solution into 32% alcohol solution. Find how much pure alcohol he should mix in 400 mL of 15% alcohol solution to obtain required solution?
Answer:
15% of alcohol mixture = 400 mL
∴ Alcohol = 15/100 × 400 = 60 mL
and other solution = 400 – 60 = 340 mL
In new mixture alcohol = 32%
Other solution = 100 – 32 = 68%
In 86 mL, alcohol = 32
and in 340 mL, alcohol will be = (32 x 340)/68 = 160 mL
Already alcohol = 60 mL
∴ More alcohol required = 160 – 60 = 100 mL
Question 3. Rahul covers a distance from P to Q on bicycle at 10 km/h and returns back at 9 km/h. Anuj covers the distance from P to Q and Q to P both at 12 km/h. On calculating we find that Anuj took 10 minutes less than Rahul. Find the distance between P and Q.
Answer: Update soon
Question 4. Solve: (x-b-c)/a + (x-c-a)/b + (x-a-b)/c = 3
Answer: Update soon
— End of Linear Equations and Inequalities in One Variable MCQs Class 8 ICSE Maths Solutions :–
Return to : – ML Aggarwal Maths Solutions for ICSE Class -8
Thanks


