ML Aggarwal Rational and Irrational Number Exe-1.5 Class 8 ICSE Maths Solutions. We Provide Step by Step Answer of Exe-1.5 Questions for Rational and Irrational Number as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-8.
ML Aggarwal Rational and Irrational Number Exe-1.5 Class 8 ICSE Maths Solutions
| Board | ICSE |
| Publications | Avichal Publishig Company (APC) |
| Subject | Maths |
| Class | 8th |
| Chapter-1 | Rational and Irrational |
| Writer | ML Aggarwal |
| Book Name | Understanding |
| Topics | Solution of Exe-1.5 Questions |
| Edition | 2023-2024 |
Rational and Irrational Number Exe-1.5
ML Aggarwal Class 8 ICSE Maths Solutions
Page-24
Question 1. Represent the following rational numbers on the number line:
(i) 11 /4
(ii) 4(3/5)
(iii) – 9 / 7
(iv) – 2 / – 5
Answer :
(i) 11 /4
= 2(3/4)
The given rational number on the number line is as shown below:

(ii) 4(3/5)
The given rational number on the number line is as shown below:

(iii) – 9 / 7
The given rational number on the number line is as shown below:
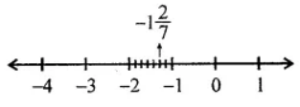
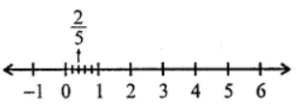
(iv) – 2 / – 5
The given rational number on the number line is as shown below:
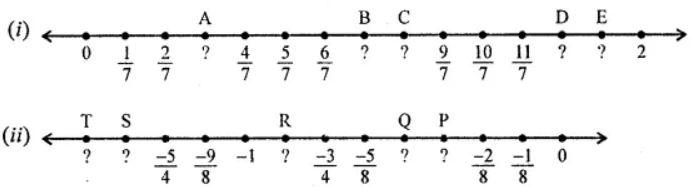
Question 2. Write the rational numbers for each point labelled with a letter:
Answer :
(i) The rational numbers for each point labelled with a letter are as follows:
A = 3 / 7
B = 7 / 7 = 1
C = 8 / 7 = 1(1/7)
D = 12 / 7 = 1(5/7)
E = 13 / 7 = 1(6/7)
(ii) The rational numbers for each point labelled with a letter are as follows:
P = – 3 / 8
Q = – 4 / 8 or – 1 / 2
R = – 7 / 8
S = – 11 / 8
T = – 12 / 8 or – 3 / 2
Rational and Irrational Number Exe-1.5
ML Aggarwal Class 8 ICSE Maths Solutions
Page-25
Question 3. Which of the two rational number is greater in each of the following pairs ?
(i) -1/2, 4/-5
(ii) -9/13, -11/-17
(iii) -5/11, 13/-17
Answer :
(i) -1/2, 4/-5
-1/2 > 4/-5
(ii) -9/13, -11/-17
-9/13 < -11/-17
(iii) -5/11, 13/-17
-5/11 > 13/-17
Question 4. Arrange the following rational number in ascending order.
-2/3, 1/-4, -5/7, 2/11
Answer :
-5/7, -2/3, 1/-4, 2/11
Question 5. Arrange the following rational number in descending order.
-3/10, -2/3, 4/-5, 0, 7/8
Answer :
7/8, 0, -3/10, -2/3, 4/-5
Question 6. Find three rational numbers between – 2 and – 1
Answer :
Three rational numbers between – 2 and – 1 can be calculated as shown below:
First rational number = 1 / 2 (- 1 – 2)
= – 3 / 2
Second rational number – 2 and – 3 / 2
= 1 / 2 {- 2 – (3 / 2)}
= 1 / 2 (- 7 / 2)
= – 7 / 4
The third rational number between – 3 / 2 and – 1
= 1 / 2 {(- 3 / 2) – 1}
= 1 / 2 (- 5 / 2)
= 1 / 2 × – 5 / 2
= – 5 / 4
Hence, three rational numbers are – 7 / 4, – 3 / 2, – 5 / 4.
Question 7. Write five rational numbers which are smaller than -4.
Answer :
Five rational numbers which are smaller than – 4
These can be a finite number of rational numbers smaller than – 4
Here, we shall take only 5 rational numbers.
The numbers are as follows:
(- 9 / 2), – 5, (- 11 / 2), – 6, (- 13 / 2), etc.
Question 8. Find twenty rational numbers between – 3 / 7 and 2 / 3
Answer :
Twenty rational numbers between – 3 / 7 and 2 / 3 can be calculated as follows:
LCM of 7, 3 = 21
Hence,
– 3 / 7 = (- 3 × 3) / (7 × 3)
= – 9 / 21
2 / 3 = (2 × 7) / (3 × 7)
= 14 / 21
Now, twenty rational numbers between – 9 / 21 and 14 / 21 are,
– 8 / 21, – 7 / 21, – 6 / 21, – 5 / 21, – 4 / 21, – 3 / 21, – 2 / 21, – 1 / 21, 0, 1 / 21, 2 / 21, 3 / 21, 4 / 21, 5 / 21, 6 / 21, 7 / 21, 8 / 21, 9 / 21, 10 / 21, 11 / 21, 12 / 21 and 13 / 21
Question 9. Find six rational numbers between – 1 / 2 and 5 / 4
Answer :
Six rational numbers between – 1 / 2 and 5 / 4 can be calculated as shown below:
LCM of 2, 4 = 4
– 1 / 2 = (- 1 × 2) / (2 × 2)
= – 2 / 4
Now, six rational numbers between – 1 / 2 and 5 / 4 are as follows:
– 1 / 4, 0, 1 / 4, 2 / 4, 3 / 4 and 4 / 4
Question 10. Identify the rational number which is different from the other three. Explain your reasoning
(- 5 / 11), (- 1 / 2), (- 4 / 9), (- 7 / 3)
Answer :
-7/3 is different as its denominator is less than its numerator,
in others, denominators are greater than their numerators respectively.
(- 5 / 11), (- 1 / 2), (- 4 / 9), (- 7 / 3)
— : End of ML Aggarwal Rational and Irrational Number Exe-1.5 Class 8 ICSE Maths Solutions :–
Return to – ML Aggarwal Maths Solutions for ICSE Class -8
Thanks
Please Share with Your Friends


