ML Aggarwal Squares and Squares Roots Check Your Progress Class 8 ICSE Ch-3 Maths Solutions. We Provide Step by Step Answer of Check Your Progress Questions for Squares and Squares Roots as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-8.
ML Aggarwal Squares and Squares Roots Check Your Progress Class 8 ICSE Maths Solutions
| Board | ICSE |
| Publications | Avichal Publishig Company (APC) |
| Subject | Maths |
| Class | 8th |
| Chapter-3 | Squares and Squares Roots |
| Writer | ML Aggarwal |
| Book Name | Understanding |
| Topics | Solution of Check Your Progress Questions |
| Edition | 2023-2024 |
Squares and Squares Roots Check Your Progress
ML Aggarwal Class 8 ICSE Maths Solutions
Page-66
Question 1: Show that 1089 is a perfect square. Also find the number whose square is 1089.
Answer:
1089 = 3 × 3 × 11 × 11
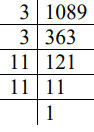
∵ Prime factors are in pairs and no factor is left.
∴ It is a perfect square and its square root = 3 × 11 = 33
Question 2: Find the smallest number which should be multiplied by 3675 to make it a perfect square. Also find the square root of this perfect square.
Answer:
3675
Factorising, we get
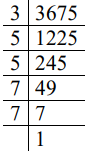
3675 = 3 × 5 × 5 × 7 × 7
Pairing the same kinds of factors, one factor 3 is left unpaired
3675 should be multiplied by 3
We get 11025 is a perfect square of 3 × 5 × 7 = 105
Question 3: Express 144 as the sum of 12 odd numbers.
Answer:
144 = 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 + 17 + 19 + 21 + 23
Question 4: How many numbers lie between 99² and 100² ?
Answer:
Total numbers which lie between 99²
and 1002
= (99 + 100 – 1) = 198
Question 5: Write a Pythagorean triplet whose one number is 17.
Answer:
One number of Pythagorean triplet = 17
Let n² + 1 = 17 ⇒ n² = 17 – 1 = 16 = (4)²
∴ n = 4
∴ Numbers will be 2n = 2 × 4 = 8
n² – 1 = 4² – 1 = 15 and n² + 1 = 17
∴ Triplet is (8, 15, 17)
Question 6: Find the smallest square number which is divisible by each of the numbers 6, 8, 9.
Answer:
smallest Square which is divisible by 6, 8, 9

LCM of 6, 8, 9 = 2 × 3 × 3 × 4
= 2 × 2 × 2 × 3 × 3 = 72
Smallest square = 2 × 72 = 144
Question 7: In an auditorium the number of rows is equal to number of chairs in each row. If the capacity of the auditorium is 1764. Find the number of chairs in each row.
Answer:
In an auditorium, there are
Number of rows = Number of chairs in each row
But, capacity is = 1764 persons
∴ Number of chairs = √1764 = 42

Question 8: Find the length of diagonal of a rectangle whose length and breadth are 12 m and 5 m respectively.
Answer:
Length of a rectangle = 12 m
and breadth = 5 m

Question 9: Find the square root of following numbers by prime factorisation:
(i) 5625
(ii) 1521
Answer:
(i) 5625 = 3 × 3 × 5 × 5 × 5 × 5
Square root of 5625 = √5625
= 3 × 5 × 5 = 75

(ii) 1521 = 3 × 3 × 13 × 13
Square root of 1521 = √1521
= 3 × 13 = 39

Question 10: Find the square root of following numbers by long division method:
(i) 21904
(ii) 108241
Answer:
(i) √21904 = 148
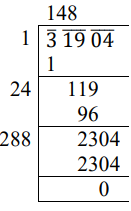
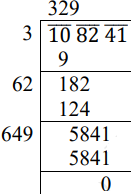
(ii) √108241 = 329
Question 11: Find the square root of following decimal numbers:
(i) 17.64
(ii) 13.3225
Answer:
(i) √17.64 = 4.2

(ii) √13.3225 = 3.65

Question 12: Find the square root of following fractions:
(i) 1(25/144)
(ii) 11(225/576)
Answer:

Question 13: Find the least number which must be subtracted from 2311 to make it a perfect square.
Answer:
2311
Taking square root, we see that 7 is left as remainder.
So, 7 is to be subtracted from 2311

Question 14: Find the least number which must be added to 520 to make it a perfect square.
Answer:
520
Taking square root of 520, we see that
(22)² < 520
Then we should take (23)²
which is 529
So, 529 – 520 = 9 is to be added
So, that we resultant number will be a perfect
square.
∴ Required number = 9

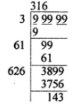
Question 15: Find the greatest number of 5 digits which is a perfect square.
Answer:
Greatest 5 digits number = 99999
Taking square root we see that 143 is left as remainder.
So, by subtracting 143 from 99999,
we get the greatest 5 digits which is a perfect square.
Required number = 99999 – 143 = 99856
— : End of ML Aggarwal Squares and Squares Roots Check Your Progress Class 8 ICSE Maths Solutions :–
Return to – ML Aggarwal Maths Solutions for ICSE Class -8
Thanks
Please Share with Your Friends