ML Aggarwal Three Dimensional Geometry ISC Class-12 Maths Solutions Chapter-2 Section-B. Step by step Solutions of ML Aggarwal ISC Class 12 Mathematics for Exercise Questions with Chapter Test. Visit official Website CISCE for detail information about ISC Board Class-12 Mathematics.

ML Aggarwal Three Dimensional Geometry ISC Class-12 Maths Solutions Chapter-2 Section-B
| Board | ISC |
| Class | 12 |
| Subject | Mathematics |
| Chapter-2 | Three Dimensional Geometry |
| Session | 2024-25 |
| Topics | Solutions of ML Aggarwal |
Three Dimensional Geometry
Direction Cosines of a Line: If the directed line OP makes angles α, β, and γ with positive X-axis, Y-axis and Z-axis respectively, then cos α, cos β, and cos γ, are called direction cosines of a line. They are denoted by l, m, and n. Therefore, l = cos α, m = cos β and n = cos γ. Also, sum of squares of direction cosines of a line is always 1,
i.e. l2 + m2 + n2 = 1 or cos2 α + cos2 β + cos2 γ = 1
Note: Direction cosines of a directed line are unique.
Straight line: A straight line is a curve, such that all the points on the line segment joining any two points of it lies on it.
Condition of Perpendicularity:
Two lines are said to be perpendicular, when in vector form 𝑏1⃗⋅𝑏2⃗=0; in cartesian form a1a2 + b1b2 + c1c2 = 0 or l1l2 + m1m2 + n1n2 = 0 [direction cosine form]
Plane:
A plane is a surface such that a line segment joining any two points of it lies wholly on it. A straight line which is perpendicular to every line lying on a plane is called a normal to the plane.
Equations of a Plane in Normal form
Vector form: The equation of plane in normal form is given by 𝑟⃗ ⋅𝑛⃗ =𝑑, where 𝑛⃗ is a vector which is normal to the plane.
Cartesian form: The equation of the plane is given by ax + by + cz = d, where a, b and c are the direction ratios of plane and d is the distance of the plane from origin.
Another equation of the plane is lx + my + nz = p, where l, m, and n are direction cosines of the perpendicular from origin and p is a distance of a plane from origin.
Note: If d is the distance from the origin and l, m and n are the direction cosines of the normal to the plane through the origin, then the foot of the perpendicular is (ld, md, nd).
Cartesian form:
If the equation of planes are a1x + b1y + c1z = d1 and a2x + b2y + c2z = d2, then equation of any plane passing through the intersection of planes is a1x + b1y + c1z – d1 + λ (a2x + b2y + c2z – d2) = 0 where, λ is a constant and calculated from given condition.
Exercise – 2.1
ML Aggarwal Three Dimensional Geometry ISC Class-12 Maths Solutions Chapter-2 Section-B
Exercise – 2.2
Three Dimensional Geometry ISC Class-12 Maths Solutions
Exercise – 2.3
Three Dimensional Geometry ISC Class-12 Maths Solutions Chapter-2 Section-B
Exercise – 2.4
ML Aggarwal ISC Class-12 Maths Solutions
Exercise – 2.5
ML Aggarwal Three Dimensional Geometry ISC Class-12 Maths Solutions Chapter-2 Section-B
Exercise – 2.6
Three Dimensional Geometry ISC Class-12 Maths Solutions
Exercise – 2.7
Three Dimensional Geometry ISC Class-12 Maths Solutions
Exercise – 2.8
Three Dimensional Geometry ISC Class-12 Maths Solutions Chapter-2 Section-B
Chapter Test
ML Aggarwal Three Dimensional Geometry
-: End of Three Dimensional Geometry ISC Class-12 Maths Solutions Chapter-2 Section-B :-
Return to :- ML Aggarwal ISC Class 12 Maths Vol-2 Solutions
Thanks
Please share with your friends
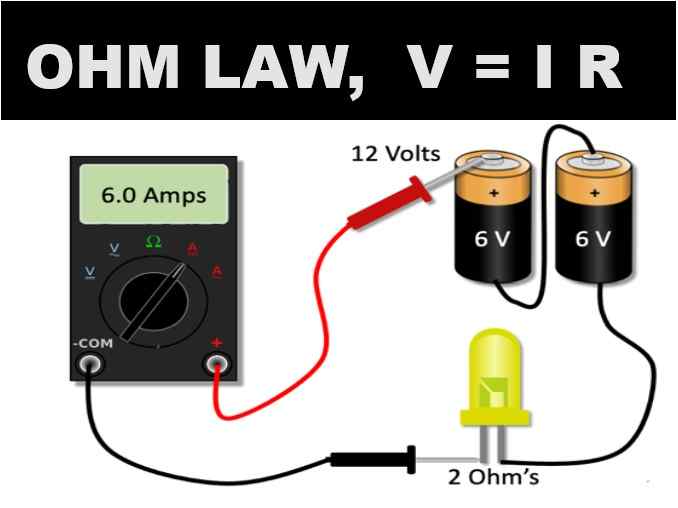
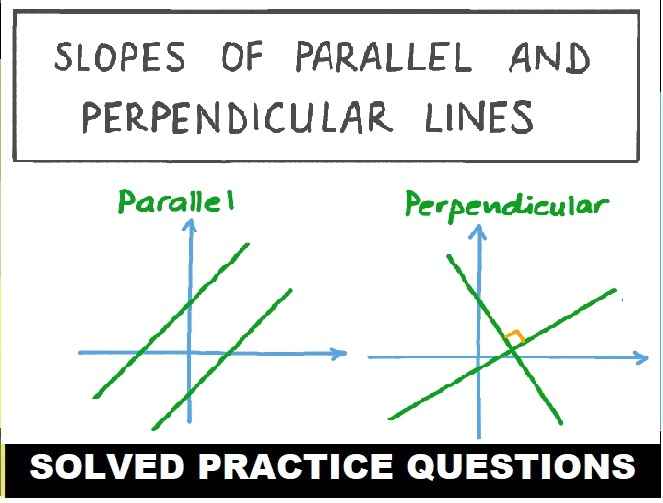

Please upload three dimensional geometry it is not showing the solutions.
now full chapter PDF showing/ working
please visit again for analysis