Rotational Motion of a rigid body Nootan Solutions ISC Physics Part-1 Class-11 Nageen Prakashan Chapter-11 Numericals of latest edition. Step by step Solutions of Kumar and Mittal ISC Physics Class-11 Nageen Prakashan Numericals Questions. Visit official Website CISCE for detail information about ISC Board Class-11 Physics.
Rotational Motion of a rigid body Nootan Solutions ISC Physics Class-11 Nageen Prakashan Chapter-11 Numericals of Kumar and Mittal.
| Class: | 11 |
| Subject: | Physics Part-1 |
| Chapter | Ch:11 Rotational Motion of a rigid body |
| Board | ISC |
| Writer / Publications | Nootan / Nageen Prakashan / Kumar and Mittal |
| Topics | Solved Numericals of page 463, 464 |
ISC Physics Class-11 Nageen Prakashan Chapter-11 Rotational Motion of a rigid body Numericals of Kumar and Mittal
Rotational motion is more complicated than linear motion, and only the motion of rigid bodies will be considered here. A rigid body is an object with a mass that holds a rigid shape, such as a phonograph turntable, in contrast to the sun, which is a ball of gas. Many of the equations for the mechanics of rotating objects are similar to the motion equations for linear motion.
Torque,
It is easier to open a door by pushing on the edge farthest from the hinges than by pushing in the middle. It is intuitive that the magnitude of the force applied and the distance from the point of application to the hinge affect the tendency of the door to rotate. This physical quantity, torque, is t = r × F sin θ, where F is the force applied, r is the distance from the point of application to the center of the rotation, and θ is the angle from r to F.
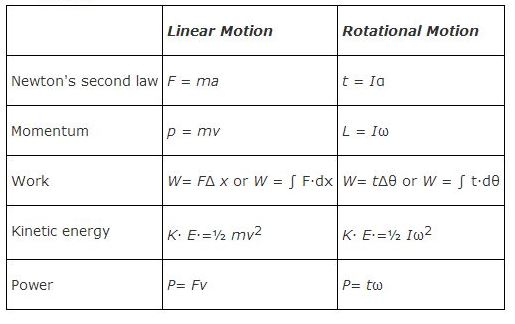
Comparison of dynamics equation for linear and rotational motion. The dynamic relations are given to compare the equation for linear and rotational motion .
Comparison of dynamics equation for linear and rotational motion
Angular momentum
Angular momentum is rotational momentum that is conserved in the same way that linear momentum is conserved. For a rigid body, the angular momentum (L) is the product of the moment of inertia and the angular velocity: L = Iω. For a point of mass, angular momentum can be expressed as the product of linear momentum and the radius ( r): L = mvr. L is measured in units of kilograms‐meters 2 per second or more commonly joule‐seconds. The law of conservation of angular momentum can be stated that the angular momentum of a system of objects is conserved if there is not external net torque acting on the system .
Types of Motion involving Rotation
- Rotation about a fixed axis (Pure rotation)
- Rotation about an axis of rotation (Combined translational and rotational motion)
- Rotation about an axis in the rotation.
Rotation About a Fixed Axis
Rotation of a ceiling fan, opening and closing of the door, rotation of our planet, rotation of hour and minute hands in analogue clocks are few examples of this type.
Kinetic Energy of Rotation
The rapidly rotating blades of a table saw machine and the blades of a fan certainly have kinetic energy due to the rotation. If we apply the familiar equation to the saw machine as a whole, it would give us kinetic energy of its centre of mass only, which is zero.
Chapter-11 Rotational Motion of a rigid body
ISC Physics Class-11 Nootan Solutions Nageen Prakashan Numericals of Kumar and Mittal
Page No. 463, 464 (Numericals)
CONTACT FOR LIVE CLASSES- 9335725646
-: End of Rotational Motion of a rigid body Nootan Solutions :–
Return to – Nootan Solutions for ISC Physics Class-11 Nageen Prakashan



I’m really thankful for this. Please do update, thank you once again.
This is really helpful!
Thanks…