Quadratic Equations Class 10 Exe- 5B RS Aggarwal Goyal Brothers ICSE Maths Solutions

Quadratic Equations Class 10 Exe- 5B RS Aggarwal Goyal Brothers ICSE Maths Solutions Ch-5. Step by step solutions of Quadratic Equation problems using Formula as latest prescribe guideline for upcoming exam. Visit official Website CISCE for detail information about ICSE Board Class-10

Quadratic Equations Class 10 Exe- 5B RS Aggarwal Goyal Brothers ICSE Maths Solutions

Board	ICSE
Subject	Maths
Class	10th
Chapter-5	Quadratic Equations
Writer/ Book	RS Aggarwal
Topics	Solution of Exe-5B Questions
Academic Session	2024-2025

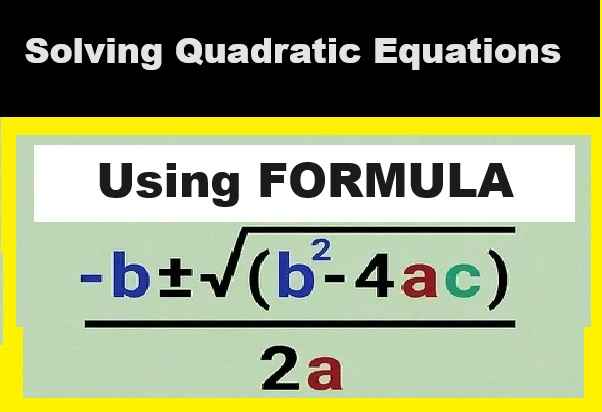
How to Solve Quadratic Equation Problems Using Formula

Change the equation as ax²+bx+c=0, where a, b, and c are real number  . Then, put the value of a. b , c,  coefficients in the given formula: x = (-b±√(b²-4ac))/(2a)

Exercise- 5B

Solve each of the following equations using quadratic formula :

Que-1: x²-4x+1 = 0

Solution- x² – 4x + 1 = 0
a = 1, b = -4, c = 1
x = [-b±√(b²-4ac)]/2a
x = [-(-4)±√(-4)²-4x1x1]/2×1
x = [4±√(16-4)]/2
x = [4±√12]/2
x = [4±2√3]/2
x = [4+2√3]/2  or  x = [4-2√3]/2
x = 2[2+√3]/2  or  x = 2[2-√3]/2
x = 2+√3  or x = 2-√3 Ans.

Que-2: 9x²+7x-2 = 0

Solution-9x²+7x-2 = 0
x = [-b±√(b²-4ac)]/2a
9x²+7x−2 = 0
⇒ x = [−7±√49+72]/18
= [−7±√121]/18
= [−7±11]/18 = 4/18, −18/18
= 2/9, −1
∴ x = 2/9, −1 Ans.

Que-3: (3x²/4)-x-1 = 0

Solution- (3x²/4)-x-1 = 0
[3x²-4x-4]/4 = 0
3x²-4x-4 = 0
from above equation : a = 3, b= -4 and c = -4
x = [-b±√(b²-4ac)]/2a
x = [-(-4)±√(-4)²-4x3x(-4)]/2×3
x = [4±√(16+48)]/6
x = [4±√64]/6
x = [4±8]/6
x = 12/6, -4/6
x = 2, -2/3 Ans.

Que-4: 4-11x = 3x²

Solution- 3x²+11x-4 = 0
⇒ 3𝑥²+12𝑥-𝑥-4 = 0
⇒ 3𝑥(𝑥+4)-1(𝑥+4) = 0
⇒ (𝑥+4)(3𝑥-1) = 0
or ⇒ 𝑥+4 = 0 or 3𝑥-1 = 0
or ⇒ 𝑥 = -4 or 𝑥 = 1/3
Hence, the roots of the equation are -4 and 1/3 Ans. 

Que-5: 25x²+30x+7 = 0

Solution-  25x² + 30x + 7 = 0
Here a = 25, b = 30, c = 7
D = b² – 4ac
= (30)² – 4 x 25 x 7
= 900 – 700
= 200
∵ x = [-𝑏±√D]/2𝑎
= [-30±√200]/2×25
= [-30±√(100×2)]/50
= [-30±10√2]/50
= [-3±√2]/5
∴ and 𝑥1 = [-3+√2]/5  and  𝑥2 = [-3-√2]/5
Hence x = [-3+√2]/5, [-3-√2]/5 Ans.

Que-6: 5x²-19x+17 = 0

Solution- 5x² – 19x + 17 = 0
a = 5 ; b = -19 ; c = 17
D = b² – 4ac
= (-19)² – 4(5)(17)
= 361 – 340
= 21
x = [-b±√(b²-4ac)]/2a
x = [19±√21]/10
x = [19+√21]/10  , x = [19-√21]/10 Ans.

Que-7: 3x²-8x+2 = 0

Solution- x = [−b±√(b²−4ac)]/2a
= [8±√(−8)²−(4⋅3⋅2)]/2⋅3
= [8±√(64−24)]6
= [8±√40]/6
= [8±√2²⋅10]/6
= [8±2√10]/6
= [4±√10]/3
x = [4+√10]/3 , [4-√10]/3 Ans.

Que-8: √3x²+10x-8√3 = 0

Solution- Consider √3𝑥²+10𝑥-8√3 = 0
Factorizing by splitting the middle term;
√3𝑥²+12𝑥-2𝑥-8√3 = 0
⇒ √3𝑥(𝑥+4√3)-2(𝑥+4√3) = 0
⇒ (√3𝑥-2)(𝑥+4√3) = 0
⇒ √3𝑥-2 = 0  or  𝑥+4√3 = 0
⇒ 𝑥 = 2/√3  or  𝑥 = -4√3
Hence, the roots of the given equation are 2/√3 and -4√3 Ans..

Que-9: 2x²+√7x-7 = 0

Solution- 2x²+√7x-7 = 0
To solve the equation,
Where,
a = 2
b = √7
c = -7
D = b² – 4ac
Putting the values in the equation,
= (√7)² – 4x2x(-7)
= 7+56 = 63
√D = √63
x = [-b±√D]/2a
x = [-(√7)±√63]/2×2
x = [-√7±√63]/4
x = -√7(1±3)/4
x = -√7(1+3)/4  or  x= √7(1-2)/4
x = (-√7×4)/4  or  x = (-√7x-2)/4
x = -√7, -√7/2 Ans.

Que-10: 6x²-31x = 105

Solution-6x²−31x−105 = 0
Applying Quadratic Formula
x = [−b±√(b²−4ac)]/2a
x = [31±√31²+4∗105∗6]/12
= [31±59]/12
⇒ x = 90/12, −28/12
x = 15/2, -7/3 Ans.

Que-11: (x+3)/(2x+3) = (x+1)/(3x+2)

Solution-  (x+3)/(2x+3) = (x+1)/(3x+2)
(x + 1) (2x + 3) = (3x + 2) (x + 3)
⇒ 2x² + 3x + 2x + 3 = 3x² + 9x + 2x + 6
⇒ 2x² + 5x + 3 – 3x² – 11x – 6 = 0
⇒ -x² – 6x – 3 = 0
⇒ x² + 6x + 3 = 0
Here a = 1, b = 6, c = 3
D = b² – 4ac
= (6)² – 4 x 1 x 3
= 36 – 12 = 24
x = [-b±√D]/2a
x = [-6±√24]/2×1
x = [-6±2√6]/2
x = -3±√6
x = -3+√6, -3-√6 Ans.

Que-12: (x-1)/(x-2) + (x-3)/(x-4) = 3*(1/3)

Solution- (x-1)/(x-2) + (x-3)(x-4) = 3*(1/3)
[(x-1)(x-4) + (x-3)(x-2)]/(x-2)(x-4) = 10/3
[x²-5x+4+x²-5x+6]/(x²-6x+8) = 10/3
[2x²-10x+10]/(x²-6x+8) = 10/3
6x^²-30x+30 = 10x^²– 60x+80
4x^²-30x+50 = 0
2x^²-15x+25 = 0
x²-(15x/2)+25/2 = 0
x²-5x-(5x/2)+25/2 = 0
(x-5)-5/2(x-5) = 0
(x-5)(x-5/2) = 0
x = 5 , x = 5/2 Ans.

Solve for x and give your answer correct to 2 decimal places :

Que-13: x²-10x+6 = 0

Solution- x²+10x+6 = 0
x = [−b±√(b²−4ac)]/2a
= [−10±√(10²−4×6×1)]/2
= [−10±√76]/2
x = −5±√19
⇒ x =−5−√19 = -5-4.36
x = −5+√19  = -5+4.36
x = 9.36, 0.64 Ans.

Que-14: 2x²-6x+3 = 0

Solution-  2x² – 6x + 3 = 0
Here a = 2, b = -6, c = 3
then D = b² – 4ac
= (-6)² – 4 x 2 x 3
= 36 – 24 = 12
Now
x = [-𝑏±√D]/2𝑎
= [(-6)±√12]/2×2
= [6±2√3]/4
∴ x₁ = [6+2√3]/4
= 2(3+√3)/4
= (3+√3)/2
x₂ = (6-2√3)/4
= 2(3-√3)/4
= (3-√3)/2
Hence x = (3+√3)/2, (3-√3)/2
x = (3+1.732)/2, (3-1.732)/2
x = 2.37, 0.64 Ans.

Que-15: 3x²-32+12 = 0

Solution- 3x²-32+12 = 0
a = 3
b = -32
c = 12
x = [-b±√(b²-4ac)]/2a
x = [-(-32±√(32²-4x3x12))]/2×3
x = [32±√(1024-144)]/6
x = [32±√880]/6
x = [32±29.66]/6
x = [32+29.66]/6  or  x = [32-29.66]/6
x = 10.28, 0.39 Ans.

Que-16: x²+7x = 7

Solution- Given quadratic equation is x^²+7x = 7
⇒𝑥²+7𝑥-7=0
Comparing with 𝑎𝑥²+𝑏𝑥+𝑐 = 0
we have a = 1, b = 7 and c = -7
𝑥 = [-𝑏±√(𝑏²-4𝑎𝑐)]/2𝑎
⇒ 𝑥 = [-7±√(7²-4×1×(-7))]/2×1
⇒ 𝑥 = [-7±√77]/2
⇒ 𝑥 = -7±8.772
⇒ 𝑥 = -7 + 8.772 and 𝑥 = -7 – 8.772
⇒ 𝑥 = 1.772 and 𝑥 = -15.772
x = 0.89 and x = -7.89 (correct to two decimal places) Ans.

Que-17: 3x²-x-7 = 0 

Solution- 3x² – x – 7 = 0
a = 3, b = -1, c = -7
x = [-𝑏±√(𝑏²-4𝑎𝑐)]/2𝑎
= [-(-1)±√(-1)²-4.3.(-7)]/2×3
= [1±√(1+84)]/6
= [1±√85]/6
= [1±9·216]/6
x = [1+9.216]/6  and  [1-9.216]/6
= 10.216/6  and  -8.216/6
= 1·703 and -1·37 Ans.

Que-18: 4x²-7x+2 = 0

Solution- 4x²-7x+2 = 0
x = [-𝑏±√(𝑏²-4𝑎𝑐)]/2𝑎
a = 4
b = -7
c = 2
x = [-(-7)±√(-7²-4x4x2)]/2×4
x = [7±√(49-32)]/8
x = [7±√17]/8
x = [7±4.12]/8
x = [7+4.12]/8  or  x = [7-4.12]/8
x = 1.39, 0.36 Ans.

Que-19: x²-7x+3 = 0

Solution- x² – 7x + 3 = 0
Comparing the above equation with the general equation ax² + bx + c = 0, we get
a = 1, b = – 7 and c = 3
Therefore the required solution is
x = {- b ± √(b² – 4ac)}/2a
or, x = {7 ± √(49 – 12)}/2
or, x = (7 ± √37)/2
or, x = 6.45138, 0.45862
or, x = 6.45, 0.46  Ans.

Que-20: Solve for x the quadratic equation x²-4x-8 = 0,

Give your answer correct to three significant figures.

Solution- 𝑥²-4𝑥-8=0
Comparing the above equation by 𝑎𝑥²+𝑏𝑥+𝑐 = 0
a = 1 , b = -4 , c = – 8
𝑥 = [-2±√(𝑏²-4𝑎𝑐)]/2𝑎
𝑥 = [4±√(-4)²-4×1×(-8)]/2×1
𝑥 = [4±√(16+32)]/2
𝑥 = [4±√48]/2
𝑥 = 4±6.9282
x = 5.46 and -1.46 Ans.

Que-21: Solve the following quadratic equation : x²+4x-8 = 0

Give your answer correct to one decimal place. (Use mathematical table if necessary)

Solution- x² + 4x – 8 = 0
Here a = 1, b = 4, c = – 8
D = b² – 4ac
= (4)² – 4 × 1 × (– 8)
= 16 + 32
= 48 > 0
∴ Roots are real.
So, x = [-𝑏±√𝐷]/2𝑎
x = [-4±√48]/2×1
= [-4±4√3]/2
= [-2±2√3]
x = [-2±(2×1.73)]
= -2 ± 3.46
⇒ x = 1.46, – 5.46
or x = 1.5, – 5.5 Ans.

–: End of Quadratic Equations Class 10 Exe- 5B RS Aggarwal Goyal ICSE Solutions  :–

Return to :–  RS Aggarwal ICSE Class 10 Solutions Goyal Brothers

Thanks

Please, Share with your friends