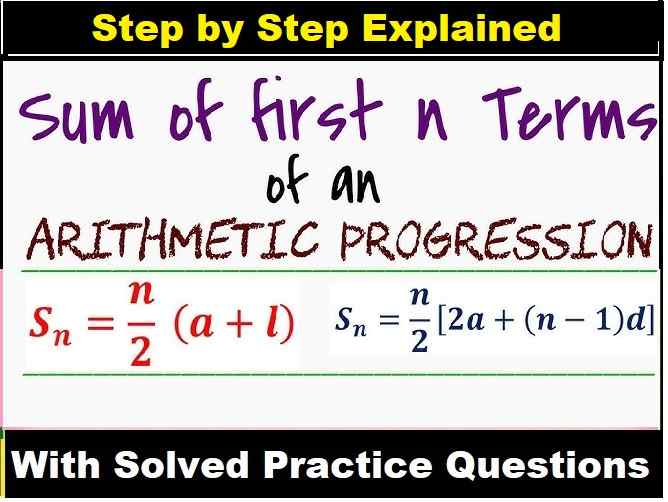
Sets Class 11 OP Malhotra Exe-1B ISC Maths Solutions Ch-1 Latest editions. In this article you would learn about Subsets, Some Theorems on Subsets and Definition. Step by step solutions of latest textbook has been given as latest syllabus. Visit official Website CISCE for detail information about ISC Board Class-11 Mathematics.
Sets Class 11 OP Malhotra Exe-1B ISC Maths Solutions Ch-1 Latest editions.
| Board | ISC |
| Publications | S Chand |
| Subject | Maths |
| Class | 11th |
| Chapter-1 | Sets |
| Writer | OP Malhotra |
| Exe-1(B) | Subsets, Some Theorems on Subsets and Definition. |
Exercise- 1B
Sets Class 11 OP Malhotra Exe-1B ISC Maths Solutions Ch-1 Latest editions
Que-1: Find the subsets of
(i) (a)
(ii) {Reena, Sonu}
(iii) Φ
(iv) {5, {7}}
Sol: (i) Required subsets are ; Φ, {a}
(ii) Required subsets are ; Φ, {Sonu}, {Reena}, {Sonu, Reena}
(iii) Required subsets are ; Φ
(iv) Required subsets are ; Φ, {5}, {{7}}, {5, {7}}
Que-2: Let A = {p, q, r}
(i) List all the subsets of A.
(ii) List all the proper subsets of A.
Sol: Given A = {p, q, r}
(i) Φ, {P}, {q}, {r}, {p, q}, {q, r), {p, r}, {p, q, r)
(ii) Φ, {p}, {q}, {r}, {p, q}, {q, r}, {p, r}
Que-3: Let P = {whole numbers less than 30}
(i) List the subsets Q {even numbers}
(ii) List the subset R {odd numbers}
(iii) List the subset S {prime numbers}
(iv) List the subset T {square numbers}
(v) List the subset U {triangle numbers}
Sol: Given P = {0, 1, 2, 3 29}
(i) Q = {0, 2, 4, 6, , 28}
(ii) R = {1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25, 27, 29}
(iii) S = {2, 3, 5, 7, 11, 13, 17, 19, 23, 29}
(iv) T = {0, 1,4,9, 16,25}
(v) U = {0, 1, 3, 6, 10, 15, 21, 28}
Que-4: Tell in each of the following, whether first set is a subset of the second set or not.
(i) A = Set of letters in the word ‘LATE’
B = Set of letters in the word ‘PLATE’
(ii) P = Set of even prime numbers.
Q = {x | x = 2p, p ∈ N and 1 ≤ p ≤ 3}
(iii) L = Set of digits in the number 1590
M = Set of digits in the number 178902
(iv) E = Set of all triangles having 4 sides.
F = Set of digits in the number ‘100’.
Sol: (i) A = {L, A, T, E} and B = {P, L, A, T, E}
Since every element of set A is a member of set B.
∴ A be a subset of B.
(ii) Given P = {2} since 2 be the only even prime number
and Q = {x | x = 2p, p ∈ N and 1 ≤ p ≤ 3}
Since p ∈ N and 1 ≤ p ≤ 3 ∴ p = { 1, 2, 3}
When p = 1 ⇒ x = 2 x 1 = 2
When p = 2 ⇒ x = 2 x 2 = 4
When p = 3 ⇒ x = 2 x 3 = 6
∴ Q = {2, 4, 6}
Since every member of set A is a member of set B.
∴ A is a subset of B.
(iii) L = {0, 1,5,9} and M = {0, 1, 2, 7, 8, 9}
since 5 ∈ L but 5 ∉ M.
∴ L is not a subset of M.
(iv) E = set of all triangles having 4 sides since their is no triangle having four sides
∴ E = Φ and F = {0, 1}
Clearly E is a subset of F as empty set is a subset of every set.
Que-5: Write the proper subsets of the following sets :
(i) {7}
(ii) {1, 3}
(iii) {c, a, b}
(iv) Φ
Sol: (i) Φ
(ii) Φ, {1}, {3}
(iii) Φ, {c}, {a}, {b}, {c, a}, {a, b}, {c, b}
(iv) it has no proper subset
Que-6: How many subsets do the following sets have ?
(i) A set having 5 elements.
(ii) The set of letters of the word ‘CENTENARY’
Sol: (i) We know that a set having n elements has 2n subsets.
given set having 5 elements the no. of subsets of given set be 25 i.e. 32.
(ii) Given set = {C, E, N, T, A, R, Y}
Clearly no. of elements in given set be 7.
∴ no. of subsets of given set = 2^7 = 128.
Que-7: How many proper subsets do the following sets have ?
(i) The set of factors of 12.
(ii) The set A {x | x is a prime numbers, x < 20}
Sol: We know that if a set A has n elements. Then number of proper subsets of A are 2n – 1.
(i) A = set of all factors of 12 = {1, 2, 3, 4, 6, 12}
∴ no. of proper subsets of A = 2n – 1
= 26 – 1 = 63
(ii) A = {2, 3, 5, 7, 11, 13, 17, 19}
Here n = 8
∴ number of proper subsets of A = 28 – 1
= 255
Que-8: Answer true or false
(i) 3 ⊆ {3, 0}
(ii) {3} ⊆ {3, 0}
(iii) Φ ⊂ {3, 0}
(iv) 0 ∈ {3, 0}
(v) Φ ⊂ {3, 0}
(vi) Φ ⊆ {Φ}
(vii) For any two sets A and B either A ⊆ B or B ⊆ A.
(viii) Every set has a proper subset.
(ix) Every subset of a finite set is finite.
(x) Every subset of an infinite set is infinite.
Sol: (i) 3 ∈ {3, 0} but 3 is not a subset of {3, 0}
∴ given statement is false.
(ii) since {3} is a subset of {3, 0}
∴ given statement is true.
(iii) since φ is not a member of {3, 0}
∴ φ ∉ {3, 0}
Hence given statement is false.
(iv) Since 0 be a member of {3, 0}
∴ 0 ∈ {3, 0}
Thus given statement is true.
(v) Since φ i.e. empty set is proper subset of {3, 0}.
∴ φ ⊂ {3, 0}
Thus given statement is true.
(vi) empty set is a subset of every set.
∴ given statement is true.
(vii) False, A = {1, 2} and B = {4, 5}
A ⊄ B orB ⊄ A
(viii) Since empty set has no proper subset
∴ given statement is false.
(ix) True statement
(x) False, since empty set is a subset of every set and φ is a finite set.
Que-9: Find the power set of each of the following sets :
(i) A = {digits in the number 98}
(ii) B = {letters in the word ‘KID’}
(iii) S = {2, 3}
(iv) T = {4, 7, 9}
Sol: We know that, power set is the set of all subsets of given set.
(i) Given A = {8, 9}
∴ P (A) = {φ, {8}, {9}, {8, 9}}
(ii) B = {K, I, D}
∴ P (A) = {φ, {K}, {I}, {D}, {K, I}, {K, D}, {I, D}, {K, I, D}}
(iii) S = {2, 3}
∴ P (S) = {Φ, {2}, {3}, {2, 3}}
(iv) T = {4, 7, 9}
∴ P(T) = {Φ, {4}, {7}, {9}, {4, 7}, {4, 9}, {7, 9}, {4, 7, 9}}
–: End Sets Class 11 OP Malhotra Exe-1B ISC Maths Solutions Ch-1 Latest editions :–
Return to :- OP Malhotra ISC Class-11 S Chand Publication Maths Solutions
Thanks
Please share with your friends