Sets Class 11 OP Malhotra Exe-1G ISC Maths Solutions

Sets Class 11 OP Malhotra Exe-1G ISC Maths Solutions Ch-1 Latest editions. In this article you would learn about Symmetric Difference of two Sets. Step by step solutions of latest textbook has been given as latest syllabus. Visit official Website CISCE for detail information about ISC Board Class-11 Mathematics.

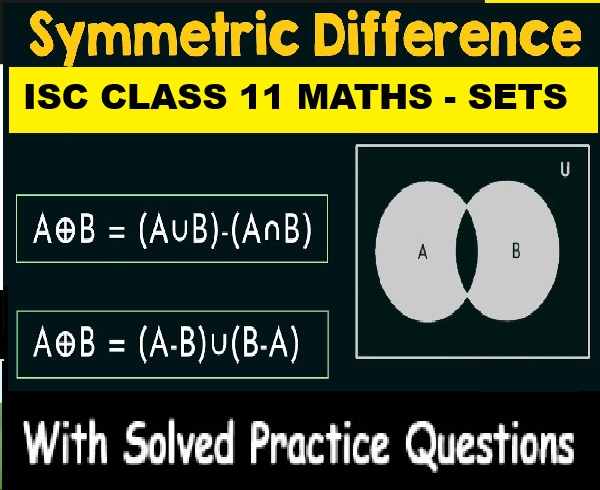
Sets Class 11 OP Malhotra Exe-1G ISC Maths Solutions Ch-1

Board	ISC
Publications	S Chand
Subject	Maths
Class	11th
Chapter-1	Sets
Writer	OP Malhotra
Exe-1(G)	Symmetric Difference of two Sets.

Exercise- 1G

Sets Class 11 OP Malhotra Exe-1G ISC Maths Solutions Ch-1 Latest editions.

Find A Δ B if

Que-1: A = {a, b, c, d}, B = {a, c, e, f}

Sol: AΔB = (A−B)∪(B−A)
A-B = {b, d}
B-A = {e, f}
AΔB = (A−B)∪(B−A)
= {b, d}∪{e, f}
= {b, d, e, f}

Que-2: A = {5, 10, 15, 25}, B = {10, 25, 30, 40}

Sol: AΔB = (A−B)∪(B−A)
A-B = {5, 15}
B-A = {30, 40}
AΔB = (A−B)∪(B−A)
= {5, 15}∪{30, 40}
= {5, 15, 30, 40}

Que-3: A = {letters of words ‘RIVER’}, B = {letters of word ‘WRITE’}

Sol: AΔB = (A−B)∪(B−A)
A = {R, I, V, E}
B = {W, R, I, T, E}
A-B = {V}
B-A = {W, T}
AΔB = (A−B)∪(B−A)
= {V}∪{W, T}
= {V, W, T}

Que-4: A = {c, a, r}, B = {m, u, t, e}

Sol: AΔB = (A−B)∪(B−A)
A-B = {c, a, r}
B-A = {m, u, t, e}
AΔB = (A−B)∪(B−A)
= {c, a, r}∪{m, u, t, e}
= {c, a, r, m, u, t, e}

Que-5: A = {x | x ∈ N, x ≤ 10}, B = {x | x is a prime number x < 6}

Sol: AΔB = (A−B)∪(B−A)
A = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}
B = {2, 3, 5}
A-B = {1, 4, 6, 7, 8, 9, 10}
B-A = {}
AΔB = (A−B)∪(B−A)
= {1, 4, 6, 7, 8, 9, 10}∪{}
= {1, 4, 6, 7, 8, 9, 10}

Que-6: A = Φ, B = {5, 7, 9, 11}

Sol: AΔB = (A−B)∪(B−A)
A-B = {Φ}
B-A = {5, 7, 9, 11}
AΔB = (A−B)∪(B−A)
= {Φ}∪{5, 7, 9, 11}
= {5, 7, 9, 11}

Que-7: If A = {3, 6, 8, 15, 19} and B = {1, 2, 6, 8, 14, 15}, then verify that AΔB = (A∪B) – (A∩B).

Sol: Given sets are :
A = {3, 6, 8, 15, 19} and B = {1, 2, 6, 8, 14, 15}
Taking RHS
A∪B = {1, 2, 3, 6, 8, 14, 15, 19}
A∩B = {6, 8, 15}
(A∪B)−(A∩B)
= {1, 2, 3, 6, 8, 14, 15, 19} − {6, 8, 15}
= {1, 2, 3, 14, 19}
Taking LHS
AΔB = (A−B)∪(B−A)
A-B = {3, 19}
B-A = {1, 2, 14}
AΔB = (A−B)∪(B−A)
= {1, 2, 3, 14, 19}
LHS = RHS
AΔB = (A∪B) – (A∩B)
Hence Proved.