ML Aggarwal Squares and Squares Roots Exe-3.1 Class 8 ICSE Ch-3 Maths Solutions. We Provide Step by Step Answer of Exe-3.1 Questions for Squares and Squares Roots as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-8.
ML Aggarwal Squares and Squares Roots Exe-3.1 Class 8 ICSE Maths Solutions
| Board | ICSE |
| Publications | Avichal Publishig Company (APC) |
| Subject | Maths |
| Class | 8th |
| Chapter-3 | Squares and Squares Roots |
| Writer | ML Aggarwal |
| Book Name | Understanding |
| Topics | Solution of Exe-3.1 Questions |
| Edition | 2023-2024 |
Squares and Squares Roots Exe-3.1
ML Aggarwal Class 8 ICSE Maths Solutions
Page-47
Question 1. Which of the following natural numbers are perfect squares? Give reasons in support of your answer.
(i) 729
(ii) 5488
(iii) 1024
(iv) 243
Answer :
(i) 729

729 = 3 × 3 × 3 × 3 × 3 × 3
729 is the product of pairs of equal prime factors
Hence, 729 is a perfect square.
(ii) 5488

5488 = 2 × 2 × 2 × 2 × 7 × 7 × 7
After pairing the same prime factors, one factor 7 is left unpaired.
Hence, 5488 is not a perfect square.
(iii) 1024

1024 = 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2 × 2
After pairing the same prime factors, there is no factor left.
Hence, 1024 is a perfect square.
(iv) 243
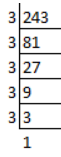
243 = 3 × 3 × 3 × 3 × 3
After pairing the same prime factors, factor 3 is left unpaired.
Hence, 243 is not a perfect square.
Question 2. Show that each of the following numbers is a perfect square. Also, find the number whose square is the given number.
(i) 1296
(ii) 1764
(iii) 3025
(iv) 3969
Answer :
(i) 1296

1296 = 2 × 2 × 2 × 2 × 3 × 3 × 3 × 3
After pairing the same prime factors, no factor is left.
Hence, 1296 is a perfect square of 2 × 2 × 3 × 3 = 36.
(ii) 1764

1764 = 2 × 2 × 3 × 3 × 7 × 7
After pairing the same factors, no factor is left.
Hence, 1764 is a perfect square of 2 × 3 × 7 = 42.
(iii) 3025

3025 = 5 × 5 × 11 × 11
After pairing the same prime factors, no factor is left.
Hence, 3025 is a perfect square of 5 × 11 = 55.
(iv) 3969

3969 = 3 × 3 × 3 × 3 × 7 × 7
After pairing the same prime factors, no factor is left.
Hence, 3969 is a perfect square of 3 × 3 × 7 = 63.
Question 3. Find the smallest natural number by which 1008 should be multiplied to make it a perfect square.
Answer :
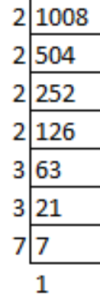
1008 = 2 × 2 × 2 × 2 × 3 × 3 × 7
After pairing the same kind of prime factors, one factor 7 is left.
Now multiplying 1008 by 7
We get a perfect square
Hence, the required smallest number is 7.
Question 4. Find the smallest natural number by which 5808 should be divided to make it a perfect square. Also, find the number whose square is the resulting number.
Answer :

5808 = 2 × 2 × 2 × 2 × 3 × 11 × 11
After pairing the same kind of prime factors, factor 3 is left.
Now dividing the number by 3, we get a perfect square.
Hence, the square root of the resulting number is 2 × 2 × 11 = 44.
— : End of ML Aggarwal Squares and Squares Roots Exe-3.1 Class 8 ICSE Maths Solutions :–
Return to – ML Aggarwal Maths Solutions for ICSE Class -8
Thanks
Please Share with Your Friends