Trigonometrical Functions Class 11 OP Malhotra Exe-4B ISC Maths Solutions Ch-4 Solutions. In this article you would learn about Providing Simple Identities. Step by step solutions of latest textbook has been given as latest syllabus. Visit official Website CISCE for detail information about ISC Board Class-11 Mathematics.

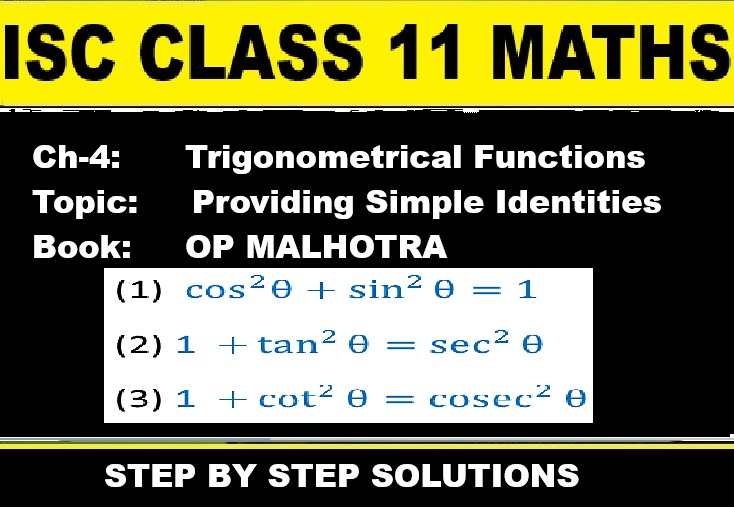
Trigonometrical Functions Class 11 OP Malhotra Exe-4B ISC Maths Solutions Ch-4

Board	ISC
Publications	S Chand
Subject	Maths
Class	11th
Chapter-4	Trigonometrical Functions
Writer	OP Malhotra
Exe-4(B)	Providing Simple Identities.

Exercise- 4B

Trigonometrical Functions Class 11 OP Malhotra Exe-4B Solution.

Prove that :

Que-1: sec θ cot θ = cosec θ

Sol: L.H.S = sec θ cot θ
= (1/cosθ) . (cosθ/sinθ) = 1/sinθ
= cosec θ

Que-2: tan θ + cot θ = sec θ cosec θ

Sol: L.H.S = tan θ + cot θ = (sinθ/cosθ) + (cosθ/sinθ)
= (sin²θ+cos²θ)/sinθ.cosθ
= 1/sinθ.cosθ
= sec θ cosec θ = R.H.S.

Que-3: cosθ/(secθ−tanθ) = 1 + sin θ.

Sol: L.H.S = cosθ/(secθ−tanθ)
= cosθ/{(1/cosθ)−(sinθ/cosθ)} = cos²θ/(1−sinθ)
= (1−sin²θ)/(1−sinθ)
= 1 + sin θ = R.H.S.

Que-4: {1+cosθ−sin²θ}/{sinθ(1+cosθ)} = cot θ.

Sol: LHS = {1+cosθ−sin²θ}/{sinθ(1+cosθ)}
= {cos²θ+cosθ}/{sinθ(1+cosθ)}
= {cosθ(1+cosθ)}/{sinθ(1+cosθ)}
= cot θ  = R.H.S

Que-5: {3−4sin²θ}/cos²θ = 3 – tan² θ.

Sol: LHS = {3−4sin²θ}/cos²θ
= 3 sec² θ – 4 tan² θ
= 3(1 + tan² θ) – 4 tan² θ
= 3 – tan² θ = R.H.S.

Que-6: (tan A + cot A) sin A x cos A = 1.

Sol: L.H.S = (tan A + cot A) sin A cos A
= {(sinA/cosA)+(cosA/sinA)} sinA.cosA
= [(sin²A+cos²A)/sinA.cosA] sinA.cosA
= 1 = R.H.S.

Que-7: cos A = cotA/cosecA = cotA√{1+cot²A} (A ∉ III or IV quad.)

Sol:

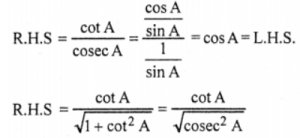
= cotA/cosecA
= (cosA/sinA)/(1/sinA)
= cos A. = LHS.   [∴ A lies in the Ist and IInd Quadrant and cosec A is positive]

Que-8: sin⁴ θ – cos⁴ θ = sin² θ – cos² θ.

Sol: L.H.S = sin⁴ θ – cos⁴ θ
= (sin² θ – cos² θ) (sin² θ + cos² θ)
= (sin² θ – cos2 θ) x 1
= sin² θ – cos² θ
= R.H.S

Que-9: sec² A + cosec² A = sec² A cosec² A.

Sol: L.H.S = sec² A + cosec² A
= (1cos²A) + (1/sin²A)
= (sin²A+cos²A)/sin²Acos²A
= 1/(sin²Acos²A) = sec² A cosec² A
= R.H.S

Que-10: sin³ θ + cos³ θ = (sin θ + cos θ) (1 – sin θ cos θ).

Sol: sin³ θ + cos³ θ
= (sin θ + cos θ) [sin² θ + cos² θ – sin θ cos θ]
= (sin θ + cos θ) (1 – sin θ cos θ) [∵ a³ + b³ = (a + b) (a² – ab + b²)]

Que-11: sin² Φ (1 + cot² Φ) = 1.

Sol: L.H.S = sin² Φ (1 + cot² Φ)
= sin² Φ cosec² Φ = 1
= R.H.S.

Que-12: (tan A + cot A)² = cosec² A + sec² A.

Sol: L.H.S = (tan A + cot A)²
= tan² A + cot² A + 2 tan A cot A
= sec² A – 1 + cosec² A – 1 + 2
= sec² A + cosec² A
= R.H.S.

Que-13: sec⁴ A – sec² A = tan² A + tan4 A.

Sol: L.H.S = sec⁴ A – sec² A
= sec² A (sec² A – 1)
= sec² A tan² A
= tan² A (1 + tan² A)
= R.H.S.

Que-14: (cosec θ – sin θ) (sec θ – cos θ) (tan θ + cot θ) = 1.

Sol: (cosecθ-sinθ) (secθ-cosθ) (tanθ+cotθ)
Simplifying the given expression, we have
(cosecθ-sinθ) (secθ-cosθ) (tanθ+cotθ)
= ((1/sinθ)-sinθ) ((1/cosθ)-cosθ) ((sinθ/cosθ)+(cosθ/sinθ))
= {1-(sin²θ/sinθ)} × {1-(cos²θ/cosθ)} × {(sin²θ+cos²θ)/sinθcosθ}
= (cos²θ/sinθ) × (sin²θ/cosθ) × {1/(sinθcosθ)}
= (cos²θsin²θ)/(sin²θcos²θ)
= 1

Que-15: (cotA+tanB)/(cotB+tanA) = cot A tan B

Sol: (cotA+tanB)/(cotB+tanA) = cotA tanB
= (cotA+tanB)/(cotB+tanA)
= {(1/tanA)+tanB}/{(1/tanB)+tanA}
= {(1+tanAtanB)/tanA}/{(1+tanAtanB)/tanB}
= {(1+tanAtanB)/tanA} . {tanB/(1+tanAtanB)}
= tanB/tanA
= (1/tanA) . tanB
= cotA tanB.

Que-16: {sinα/(1+cosα)} + {(1+cosα)/sinα} = 2 cosec α.

Sol:  LHS = {sinα/(1+cosα)} + {(1+cosα)/sinα}
= {(sinα)²+(1+cosα)²}/{sinα(1+cosα)}
= {sin²α+cos²α+1+2cosα}/{sinα(1+cosα)}
= {2+2cosα}/{sinα(1+cosα)}
= {2(1+cosα)}/{sinα(1+cosα)}
= 2/sinα
= 2 cosecα

Que-17: 1 + (1/cosA) = tan²A/(secA−1)

Sol:  R.H.S = tan²A/(secA−1)
= (sec²A−1)/(secA−1)
= {(secA−1)(secA+1)}/(secA−1)
= Sec A + 1
= (1/cosA) + 1 = L.H.S

Que-18: (1+cosA)/(1−cosA) = (cosec A + cot A)²

Sol:   RHS = (cosec A + cot A)²
= {(1/sinA)+(cosA/sinA)}²
= {(1+cosA)²}/sin²A
= {(1+cosA)²}/(1-cos²A)
= {(1+cosA)²}/{(1-cosA)(1+cosA)}
= (1+cosA)/(1-cosA) = LHS.

Que-19: If tan θ + sin θ = m and tan θ – sin θ = n, show that m² – n² = 4 sin θ tan θ.

Sol:  Given tan θ + sin θ = m … (1)
and tan θ – sin θ = n … (2)
LHS = m² – n²
= (tan θ + sin θ)² – (tan θ – sin θ)²
= tan²θ + sin²θ + 2tanθsinθ – tan²θ – sin²θ + 2tanθsinθ
= 4 tanθ sinθ RHS.

Que-20: Prove that 1 – {sin²y/(1-cos y)} + {(1+cos y)/sin y} – {sin y/(1-cos y)} = cos y.

Sol:  1 – {sin²y/(1-cos y)} + {(1+cos y)/sin y} – {sin y/(1-cos y)}
= 1 – {(1-cos²y)/(1-cos y)} + {(1+cos y)/sin y} – {sin y/(1-cos y)}
= 1 – [{(1+cosy)(1-cosy)}/(1-cos y)] + {(1+cos y)/sin y} – {sin y/(1-cos y)}

= cos y.

Que-21: Prove that √{(1+cosθ)/(1-cosθ))} = cosecθ + cotθ

Sol:  LHS = √{(1+cosθ)/(1-cosθ))}
= √{(1+cosθ)(1+cosθ)/(1-cosθ)(1+cosθ))}
= √{(1+cosθ)²/sin²θ}
= (1+cosθ)/sinθ
= (1/sinθ) + (cosθ/sinθ)
= cosecθ + cotθ.

Que-22: If sinx + sin²x = 1, prove that (cos^8)x + (2cos^6)x + (cos^4)x = 1.

Sol: sinx + sin²x = 1
⇒ sinx = 1 – sin²x
⇒ sinx = cos²x
Again,
sinx + sin²x = 1
Squaring both side;
⇒ (sin^4)x + 2sin³x + sin²x = 1
⇒ (cos^8)x + (2cos^6)x + (cos^4)x = 1 {Putting cos2x = sinx}

–: End Trigonometrical Functions Class 11 OP Malhotra Exe-4B ISC Math Ch-4 Solutions :–

Return to :- OP Malhotra ISC Class-11 S Chand Publication Maths Solutions

Thanks

Please share with your friends