Area Trapezium and Polygon ICSE Class-8th Concise Selina Mathematics Solutions Chapter-20 . We provide step by step Solutions of Exercise / lesson-20 Area of Trapezium and a Polygon for ICSE Class-8 Concise Selina Mathematics.
Our Solutions contain all type Questions with Exe-20 A, Exe-20 B and Exe-20 C to develop skill and confidence . Visit official Website CISCE for detail information about ICSE Board Class-8 Mathematics.
Area of Trapezium and a Polygon ICSE Class-8th Concise Selina Mathematics Solutions Chapter-20
–: Select Topics :–
Exe – 20 A, Area of Trapezium and a Polygon ICSE Class-8th
Question 1.
Find the area of a triangle, whose sides are :
(i) 10 cm, 24 cm and 26 cm
(ii) 18 mm, 24 mm and 30 mm
(iii) 21 m, 28 m and 35 m
Answer:

(ii)
Sides of ∆ are
a = 18 mm
b = 24 mm
c = 30 mm


Question 2.
Two sides of a triangle are 6 cm and 8 cm. If height of the triangle corresponding to 6 cm side is 4 cm ; find :
(i) area of the triangle
(ii) height of the triangle corresponding to 8 cm side.
Answer:

BC = 6 cm
height AD = 4 cm
area of Δ = (1/2) base×height
= (1/2)×BC×AD
= (1/2)×6×4=12 cm²
Again area of Δ = (1/2)AC×BE
12=(1/2)×8×BE
∴ BE = (12×2)/8
BE = 3 cm
∴
(i) 12 cm2 (ii) 3 cm
Question 3.
The sides of a triangle are 16 cm, 12 cm and 20 cm. Find :
(i) area of the triangle ;
(ii) height of the triangle, corresponding to the largest side ;
(iii) height of the triangle, corresponding to the smallest side.
Answer:
Sides of ∆ are
a = 20 cm
b = 12 cm
c = 16 cm
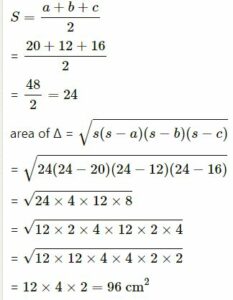
AD is height of Δ corresponding to largest side.
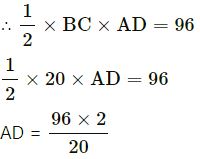
AD = 9.6 cm
BE is height of Δ corresponding to smallest side.
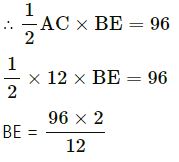
BE = 16 cm
(i) 96 cm2
(ii) 9.6 cm
(iii) 16 cm
Question 4.
Two sides of a triangle are 6.4 m and 4.8 m. If height of the triangle corresponding to 4.8 m side is 6 m; find :
(i) area of the triangle ;
(ii) height of the triangle corresponding to 6.4 m side.
Answer:
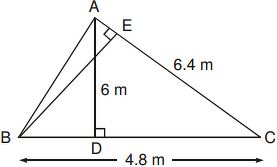
ABC is the Δ in which BC = 4.8 m
AC = 6.4m and AD = 6 m
∴ area of ΔABC = (1/2)BC×AD
= (1/2)×4.8×6
= 14.4 m2
BE is height of Δ corresponding to 6.4 m
∴ (1/2)AC×BE=14.4
(1/2)×6.4×BE=14.4
BE = (14.4×2)/6.4
BE = 14.4/3.2
= 9/2 = 4.5 m
Hence (i) 14.4 m2 (ii) 4.5 m
Question 5.
The base and the height of a triangle are in the ratio 4 : 5. If the area of the triangle is 40 m2; find its base and height.
Answer:
The base of ∆ = 4x m
and height of ∆ = 5x m
area of ∆ = 40 m2
∵ (1/2)base×height=area of Δ
(1/2)×4x×5x=40
10x²=40
x2=4
x = √4
x = 2
base = 4x = 4×2=8 m
height = 5x = 5×2=10 m
∴ 8 m; 10 m
Question 6.
The base and the height of a triangle are in the ratio 5 : 3. If the area of the triangle is 67.5 m2; find its base and height.
Answer:
Let base = 5x m
height = 3x m
Area of Δ = (1/2) base×height
∴ (1/2)×5x×3x=67.5
x²=67.5×215
x²=4.5×2
x²=9.0
x= √9
x = 3
base = 5x = 5 x 3 = 15 m
height = 3x = 3 x 3 = 9 m
Question 7.
The area of an equilateral triangle is 144√3 cm2; find its perimeter.
Answer:
Let each side of an equilateral triangle = x cm
∴ Its area = √3/4 (side)2
= (√3/4)x² = 144√3 (given)
⇒ x² = 144√3 ×(4/√3)
⇒ x² = 144×4⇒x² =576
⇒ x = √576 = 24 cm.
⇒ Each side = 24 cm
Hence perimeter = 3(24) = 72 cm.
Question 8.
The area of an equilateral triangle is numerically equal to its perimeter. Find its perimeter correct to 2 decimal places.
Answer:
Let each side of the equilateral triangle = x
∴ Its area = (√3/4)x²
Area perimeter = 3x

∴ Perimeter = 12√3 units
= 12 (1.732) = 20.784 = 20.78 units
Question 9.
A field is in the shape of a quadrilateral ABCD in which side AB = 18 m, side AD = 24 m, side BC = 40m, DC = 50 m and angle A = 90°. Find the area of the field.
Answer:
Since ∠A = 90°
By Pythagoras Theorem,
In ∆ABD,
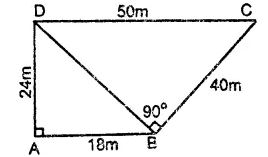

Now, the area of ΔABD = 1/2(18)(24)
= (18) (12) = 216 m2
Again in ΔBCD; sides are 30,40,50
⇒ By Pythagoras Theorem ∠CBD = 90°
[∴ DC2 = BD2 + BC2, Since (50)2 = (30)2 + (40)2]
Area of ΔBCD = 1/2(40)(30)=600 m2
Hence, area of quadrilateral ABCD = Area of ΔABD + area of ΔBCD
= 216 + 600 = 816 m2.
Question 10.
The lengths of the sides of a triangle are in the ratio 4 : 5 : 3 and its perimeter is 96 cm. Find its area.
Answer:
Let the sides of the triangle ABC be 4x, 5x and 3x
Let AB = 4x, AC = 5x and BC = 3x
Perimeter = 4x + 5x + 3x = 96
=> 12x = 96
⇒ x = 96/12
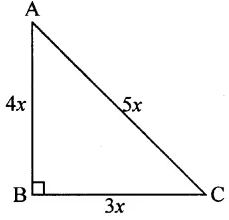
∴ x = 8
∴ Sides are
BC = 3(8) = 24 cm, AB = 4(8) = 32 cm,
AC = 5(8) = 40 cm
Since (AC)2 = (AB)2 + (BC)2 [∵ (5x)2 = (3x)2 + (4x)2]
∴ By Pythagoras Theorem, ∠B = 90°
∴ Area of ΔABC = 1/2(BC)(AB) = 1/2(24)(32)
= 12×32=384 cm²
Question 11.
One of the equal sides of an isosceles triangle is 13 cm and its perimeter is 50 cm. Find the area of the triangle.
Answer:
In isosceles ∆ABC
AB = AC = 13 cm But perimeter = 50 cm
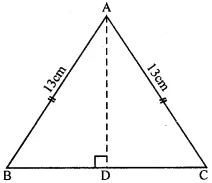
∴ BC = 50 – (13 + 13) cm
= 50 – 26 = 24 cm
AD ⊥ BC
∴ AD = DC = 24/2=12 cm.
In right ΔABD,
AB2 = AD2 + BD2 (Pythagoras Theorem)
(13)²=AD²+(12)²
⇒ 169 = AD²+144
⇒ AD²=169-144
= 25 = (5)2
∴ AD = 5 cm.
Now area of ΔABC = (1/2) Base×Altitude
= (1/2)×BC×AD
= (1/2)×24×5=60 cm2
Question 12.
The altitude and the base of a triangular field are in the ratio 6 : 5. If its cost is ₹ 49,57,200 at the rate of ₹ 36,720 per hectare and 1 hectare = 10,000 sq. m, find (in metre) dimensions of the field,
Answer:
Total cost = ₹ 49,57,200
Rate = ₹ 36,720 per hectare
The total area of the triangular field
= (4957200/36720)×10000 m2= 1350000m2
The ratio in altitude and base of the field = 6: 5
Let altitude = 6x
and base = 5x
∴ Area = (1/2) Base×Altitude
⇒ 1350000 = (1/2)×5x×6x
⇒ 15x²=1350000
⇒ x² = 1350000/15
⇒ x² = 90000 = (300)²
∴ x = 300
∴ Base = 5x = 5×300=1500 m
and altitude = 6x=6×300=1800m
Question 13.
Find the area of the right-angled triangle with hypotenuse 40 cm and one of the other two sides 24 cm.
Answer:
In right angled triangle ABC Hypotenuse AC = 40 cm
One side AB = 24 cm
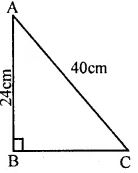
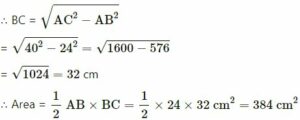
Question 14.
Use the information given in the adjoining figure to find :
(i) the length of AC.
(ii) the area of a ∆ABC
(iii) the length of BD, correct to one decimal place.

Answer:

ICSE Class-8 Exe-20 B Maths Area of Trapezium and a Polygon Concise Selina Mathematics
Question 1.
Find the length and perimeter of a rectangle, whose area = 120 cm2 and breadth = 8 cm
Answer::
area of rectangle = 120 cm2
breadth, b = 8 cm
Area = l x b
l x 8 = 120
l = 120/8 = 15 cm
Perimeter = 2 (l+b) = 2(15+8) = 2 x 23 = 46 cm
Length = 15 cm; Perimeter = 46 cm
Question 2.
The perimeter of a rectangle is 46 m and its length is 15 m. Find its :
(i) breadth
(ii) area
(iii) diagonal.
Answer::
(i)
Perimeter of rectangle = 46 m
length, l = 15 m
2 (l+b) = 46
2(15 + b) = 46
15+b = 46/2 = 23
b = 23 – 15
b = 8 m
(ii)
area = l×b=15×8=120 m2
(iii)
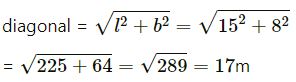
Hence
(i) 8 m
(ii) 120 m2
(iii) 17 m
Question 3.
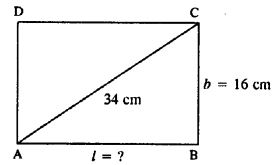
The diagonal of a rectangle is 34 cm. If its breadth is 16 cm; find its :
(i) length
(ii) area
Answer::
AC2 = AB2+BC2 (By Pythagoras theorem)
(34)2 = l2 + (16)2
1156 = l2 + 256
l2 = 1156 – 256
l2 = 900
l = √900 = 30 cm
area = l x b = 30 x 16 = 480 cm2
(i) 30 cm
(ii) 480 cm2
Question 4.
The area of a small rectangular plot is 84 m2. If the difference between its length and the breadth is 5 m; find its perimeter.
Answer::
Area of a rectangular plot = 84 m2
Let breadth = x m
Then length = (x + 5) m
Area = l x b
x(x + 5) = 84
x2 + 5x – 84 = 0
=> x2+ 12x – 7x – 84 = 0
=> x(x + 12) – 7(x + 12) = 0
=> (x + 12) (x – 7) = 0
Either x + 12 = 0, then x
= -12 which is not possible being negative
or x – 7 = 0, then x = 7
Length = x + 5 = 7 + 5 = 12m
and breadth = x = 7 m
Perimeter = 2(l + b) = 2(12+ 7) = 2 x 19 m = 38 m
Question 5.
The perimeter of a square is 36 cm; find its area
Answer::
Perimeter of Square = 36 cm
Side = (Perimeter/4) = 36/4 = 9 cm
∴ Area of square = side × side
= 9 × 9
= 81 cm2
Question 6.
Find the perimeter of a square; whose area is : 1.69 m2
Answer::
Side = √area = √1.69 = 1.3 m
Perimeter = 4 x side = 4 x 1.3 = 5.2 m
Question 7.
The diagonal of a square is 12 cm long; find its area and length of one side.
Answer::
Let side of square = a cm
diagonal = 12 cm
By Pythagoras Theorem, a2 + a2 = (12)2
2a2 = 144
a2 = 72
Area of square = a2 = 72 cm2
a2 = 72
a = √72 = 8.49 cm
Question 8.
The diagonal of a square is 15 m; find the length of its one side and perimeter.
Answer::
Diagonal of square = 15 m
Let side of square = a
a2 + a2 = (15)2 = 225
a2 = 225/2 = 112.50
a = √112.50 = 10.6 m
Perimeter = 4 x a = 10.6 x 4 = 42.4 m
Question 9.
The area of a square is 169 cm2. Find its:
(i) one side
(ii) perimeter
Answer::
Let each side of the square be x cm.
Its area = x2 = 169 (given)
x = √169
x = 13 cm
(i) Thus, side of the square = 13 cm
(ii) Again perimeter = 4 (side)
= 4 x 13
= 52 cm
Question 10.
The length of a rectangle is 16 cm and its perimeter is equal to the perimeter of a square with side 12.5 cm. Find the area of the rectangle.
Answer::
Length of the rectangle is = 16 cm
Let its breadth be x cm
Perimeter = 2 (16 + x) = 32 + 2x
Also perimeter = 4(12.5) = 50 cm.
According to statement,
32 + 2x = 50
= 2x = 50 – 32
= 18
= x = 9
Breadth of the rectangle = 9 cm.
Area of the rectangle (l x b)= 16 x 9 = 144 cm2
Question 11.
The perimeter of a square is numerically equal to its area. Find its area.
Answer::
Let each side of the square be x cm.
Its perimeter = 4x,
Area = x2
By the given condition 4x = x2
= x2 – 4x = 0
= x (x – 4) = 0
= x = 4 [x ≠ 0]
Area = x2 = (4)2
= 4 x 4 = 16 sq.units.
Question 12.
Each side of a rectangle is doubled. Find the ratio between :
(i) perimeters of the original rectangle and the resulting rectangle.
(ii) areas of the original rectangle and the resulting rectangle.
Answer::
Let length of the rectangle = x
and breadth of the rectangle = y
(i)
Perimeter P = 2(x + y)
Again, new length = 2x
New breadth = 2y
∴ New perimeter P’ = 2(2x + 2y)
= 4(x + y) = 2.2(x + y) = 2P
= P/P′ = 1/2
i.e. P : P’ = 1 : 2
(ii)
Area A = xy
New Area A’ = (2x)(2y) = 4xy = 4A
∴ A/A′ = 1/4 i.e. A : A’ = 1 : 4
Question 13.
In each of the following cases ABCD is a square and PQRS is a rectangle. Find, in each case, the area of the shaded portion.
(All measurements are in metre).

Answer::
(i)
Area of the shaded portion
= Area of the rectangle PQRS – Area of square ABCD
= 3.2 x 1.8 – (1.4)2 (∵ PQ = 3.2 and PS = 1.8) Side of square AB = 1.4
= 5.76 – 1.96 = 3.80 = 3.8 m2
(ii)
Area of the shaded portion = Area of square ABCD – Area of rectangle PQRS
= 6 x 6 – (3.6) (4.8) = 36 – 17.28 = 18.72 m2
Question 14.
A path of uniform width, 3 m, runs around the outside of a square field of side 21 m. Find the area of the path.
Answer::
According to the question the figure will be as shown alongside.
Clearly, the length of the square field excluding path = 21 m.

Area of the square side excluding the path = 21 x 21 = 441 m2
Again, length of the square field including the path = 21 + 3 + 3 = 27 m
Area of the square field including the path = 27 x 27 = 729 m2
Area of the path = 729 – 441 = 288 m2
Question 15.
A path of uniform width, 2.5 m, runs around the inside of a rectangular field 30 m by 27 m. Find the area of the path.
Answer::
According to the question, the figure will be as shown alongside.
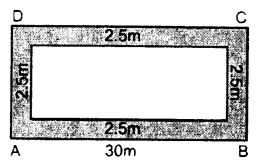
Clearly, the length of the rectangular field including the path = 30 m.
Breadth = 27 m.
Its Area = 30 x 27 = 810 m2
Width of the path = 2.5 m
Length of the rectangular field including the path = 30 – 2.5 – 2.5 = 25 m.
Breadth = 27 – 2.5 – 2.5 = 22m
Area of the rectangular field including the path = 25 x 22 = 550 m2
Hence, area of the path = 810 – 550 = 260 m2.
Question 16.
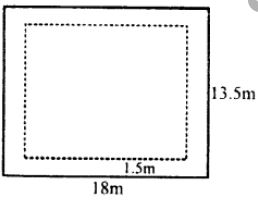
The length of a hall is 18 m and its width is 13.5 m. Find the least number of square tiles, each of side 25 cm, required to cover the floor of the hall,
(i) without leaving any margin.
(ii) leaving a margin of width 1.5 m all around. In each case, find the cost of the tiles required at the rate of Rs. 6 per tile
Answer::
(i) Length of hall (l) = 18 m and breadth (b) = 13.5 m
∴ Area of the floor = l × b
=18×13.5 m²=243.0 m²
Side of each square tiles (a) = 25 cm
= 25/100 = 1/4m
∴ Area of one tile = a2 = (1/4) × (1/4)
= 1/16 m²
No. of tiles required = 243+(1/16)
= (243×16)/1=3888
Rate of tiles = Rs. 6 per tile
∴ Total cost = Rs. 3888 × 6
= Rs. 23328
(ii)
Width of margin left in side = 1.5 m
∴ Inner length = 18-2×1.5=18-3=15 m
and breath = 13.5-2×1.5=13.5-3
= 10.5 m
∴ Inner area = 15×10.5 m²
= 157.5 m2
∴ No. of tiles = 157.5 + (1/16)
= 157.5×16=2520
∴ Cost of files = 2520 × 6 = Rs. 15120
Question 17.
A rectangular field is 30 m in length and 22m in width. Two mutually perpendicular roads, each 2.5 m wide, are drawn inside the field so that one road is parallel to the length of the field and the other road is parallel to its width. Calculate the area of the crossroads.
Answer::
Length of the rectangular field (l) = 30 m and breadth (b) = 22m
width of parallel roads perpendicular to each other inside the field =2.5m
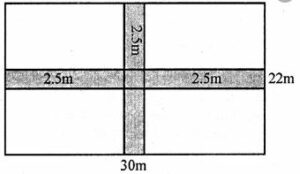
Area of cross roads = width of roads (Length + breadth) – area of middle square
= 2.5 (30 + 22) – (2.5)2
= 2.5 x 52 – 6.25 m2
= (130 – 6.25) m = 123.75 m2
Question 18.
The length and the breadth of a rectangular field are in the ratio 5 : 4 and its area is 3380 m2. Find the cost of fencing it at the rate of ₹75 per m.
Answer::
Ratio in length and breadth = 5 : 4
Area of rectangular field = 3380 m2
Let length = 5x and breadth = 4x
5x x 4x = 3380
=> 20x2= 3380
x2 = 3380/20 = 169 = (13)2
x = 13
Length = 13 x 5 = 65 m
Breadth =13 x 4 = 52 m
Perimeter = (l + b) = 2 x (65 + 52) m = 2 x 117 = 234 m
Rate of fencing = ₹ 75 per m
Total cost = 234 x 75 = ₹ 17550
Question 19.
The length and the breadth of a conference hall are in the ratio 7 : 4 and its perimeter is 110 m. Find:
(i) area of the floor of the hall.
(ii) number of tiles, each a rectangle of size 25 cm x 20 cm, required for flooring of the hall.
(iii) the cost of the tiles at the rate of ₹ 1,400 per hundred tiles.
Answer::
Ratio in length and breadth = 7 : 4
Perimeter = 110 m
∴ Length + Breadth = 110/2=55 m
Sum of ratios = 7 + 4 = 11
∴ Length = (55×7)/11=35 m
and breath = (55×4)/11=20 m
(i)
Area of floor = l × b
= 35×20=700 m²
(ii)
Size of tile = 25 cm × 20 cm

(iii)
Cost of tiles = ₹ 1400 per 100 tiles
∴ Total cost = (14000×1400)/100
= ₹ 196000
Exe- 20 C Area of Trapezium and a Polygon ICSE Class-8th Concise Selina Mathematics
Question 1.
The following figure shows the cross-section ABCD of a swimming pool which is trapezium in shape.
If the width DC, of the swimming pool is 6.4cm, depth (AD) at the shallow end is 80 cm and depth (BC) at deepest end is 2.4m, find Its area of the cross-section.

Answer::
Area of the cross-section = Area of trapezium ABCD
= 1/2(Sum of parallel sides)×height
= 1/2(80+240)×6.4
= (320) (3.2) = (32) (32)
= 1024 cm2 or = 10.24 sq.m.
Question 2.
The parallel sides of a trapezium are in the ratio 3 : 4. If the distance between the parallel sides is 9 dm and its area is 126 dm2 ; find the lengths of its parallel sides.
Answer::
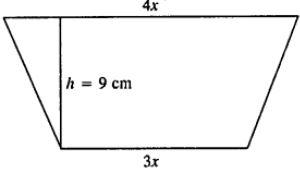
Let parallel sides of trapezium be
a = 3x
b = 4x
Distance between parallel sides, h = 9 dm
area of trapezium = 126 dm2
1/2(a+b)×h=126
1/2(3x + 4x) × 9=126
7x×9=126×2
x= (126×2)/(7×9)
x = 4
a = 3x = 3 × 4 = 12 dm
b = 4x = 4 × 4 = 16 dm
12 dm , 16 dm
Question 3.
The two parallel sides and the distance between them are in the ratio 3 : 4 : 2. If the area of the trapezium is 175 cm2, find its height.
Answer::
The two parallel sides and the distance between them be 3x, 4x, 2x cm respectively
Area = 1/2 (sum of parallel sides) x (distance between parallel sides)
= 1/2 (3x + 4x) x 2x = 175 (given)
=> 7x x x = 175
=> 7x2 = 175
=> x2 = 25
=> x = 5
Height i.e. distance between parallel sides = 2x = 2 x 5 = 10 cm
Question 4.
A parallelogram has sides of 15 cm and 12 cm; if the distance between the 15 cm sides is 6 cm; find the distance between 12 cm sides.
Answer::
Base, AB = 15 cm
Distance between 15 cm sides
i.e. height DP = 6 cm
∴ Area of ||gm = Base × height
= AB × DP
= 15 × 6 = 90 cm2
Let BQ be the distance between 12 cm sides
∴ AD × BQ = area of ||gm ABCD
∴ 12 × BQ = 90
BQ = 90/12
BQ = 15/2=7.5 cm
Question 5.
A parallelogram has sides of 20 cm and 30 cm. If the distance between its shorter sides is 15 cm; find the distance between the longer sides.
Answer::
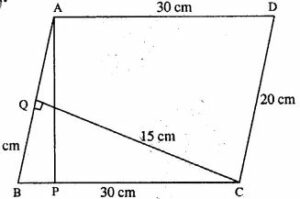
ABCD be the ||gm in which
BC = 30 cm
and CD = 20 cm
Distance between shorter sides,
i.e. CQ = 15 cm
∴ area of ||gm = AB × CQ
= 20 × 15
= 300 cm2
Again BC × AP = Area of || gm
30 × AP = 300
AP = 300/30
AP = 10 cm
∴ Distance between larger sides is = 10 cm
Question 6.
The adjacent sides of a parallelogram are 21 cm and 28 cm. If its one diagonal is 35 cm; find the area of the parallelogram.
Answer::

First, we find the area of ΔABC.
Sides are, a = 28 cm
b = 35 cm
and c = 21 cm

= 2×21×7
= 294 cm2
∵ Diagonal of ||gm divides it into two equal parts.
∴ area of ||gm = 2×area of ΔABC
= 2 × 294
= 588 cm2
Question 7.
The diagonals of a rhombus are 18 cm and 24 cm. Find:
(i) its area ;
(ii) length of its sides.
(iii) its perimeter;
Answer::
(i)
The diagonal of the rhombus is 18 cm and 24 cm.
area of rhombus = 1/2 x Product of diagonals
= (1/2) x 18 x 24
= 216 cm2
(ii)
Diagonals of a rhombus bisect each other at right angles.
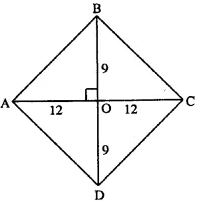
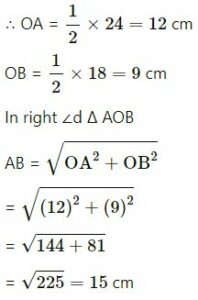
∴ Side of rhombus = 15 cm
(iii)
Perimeter of rhombus = 4×side
= 4×15=60 cm
(i) 216 cm2 (ii) 15 cm (iii) 60 cm.
Question 8.
The perimeter of a rhombus is 40 cm. If one diagonal is 16 cm; find :
(i) its another diagonal
(ii) area
Answer::
(i)
Perimeter of rhombus = 40 cm
side = (1/2) x 40 = 10 cm
One diagonal = 16 cm
Diagonals of rhombus bisect each other at right angles.
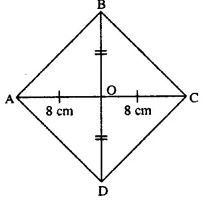

= 6 cm
∴ diagonal BD = 6×2=12 cm
(ii)
Area of rhombus =(1/2)×product of diagonals
= (1/2)×12×16
= 96 cm2
∴ (i) 12 cm (ii) 96 cm2
Question 9.
Each side of a rhombus is 18 cm. If the distance between two parallel sides is 12 cm, find its area.
Answer:
Each side of the rhombus = 18 cm
base of the rhombus = 18 cm
Distance between two parallel sides = 12 cm
Height = 12 cm
Area of the rhombus = base x height = 18 x 12 = 216 cm2
Question 10.
The length of the diagonals of a rhombus is in the ratio 4 : 3. If its area is 384 cm2, find its side.
Answer:
Let the lengths of the diagonals of a rhombus are 4x, 3x.
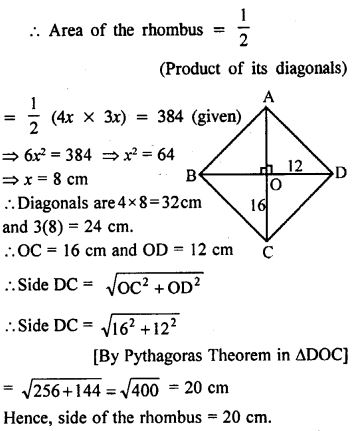
Question 11.
A thin metal iron-sheet is rhombus in shape, with each side 10 m. If one of its diagonals is 16 m, find the cost of painting its both sides at the rate of ₹ 6 per m2.
Also, find the distance between the opposite sides of this rhombus.
Answer:
Side of rhombus-shaped iron sheet = 10 m and one diagonals (AC) = 16 m
Join BD diagonal which bisects AC at O
The diagonals of a rhombus bisect each other at the right angle
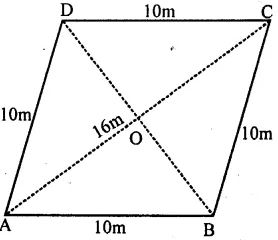
∴ AO = OC = 16/2 = 8m.
Now in right ΔAOB
AB2 = AO2 + BO2 ⇒ (10)2 = (8)2 + BO2
⇒ 100 = 64 + BO2 ⇒ BO2 = 100 – 64 = 36
= (6)2
∴ BO = 6 m
∴ BD = 2 × BO = 2 × 6 = 12 m
Now, the area of rhombus = d1×d2/2
= (16×12)/2=96 m²
Rate of painting = ₹ 6 per m2
∴ The total cost of painting both sides,
= 2×96×6 = ₹ 1152
Distance between two opposite sides,
= Area Base = 9610 = 9.6 m
Question 12.
The area of a trapezium is 279 sq.cm and the distance between its two parallel sides is 18 cm. If one of its parallel sides is longer than the other side by 5 cm, find the lengths of its parallel sides.
Answer:
Area of trapezium = 279 sq.cm
Distance between two parallel lines (h) = 18 cm
∴ Sum of parallel sides = (Area×2)/Height
= (279×2)/18 =31 m
Let shorter side, CD = x
Then longer side = x + 5
∴ x + x + 5 = 31
⇒ 2x = 31 – 5 = 26
⇒ x = 26/2
=13
∴ Shorter side = 13 cm
and longer side = 13 + 5 = 18 cm
Question 13.
The area of a rhombus is equal to the area of a triangle. If base of ∆ is 24 cm, its corresponding altitude is 16 cm and one of the diagonals of the rhombus is 19.2 cm. Find its other diagonal.
Answer:
Area of a rhombus = Area of a triangle Base of triangle = 24 cm and altitude = 16 cm
∴ Area = 1/2 base×altitude
= 1/2 × 24×16=192 cm2
∴ Area of rhombus = 192 cm2
one diagonal = 19.2 cm

Question 14.
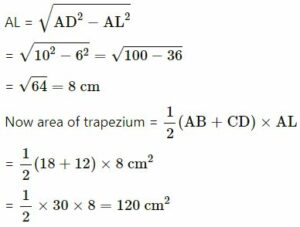
Find the area of the trapezium ABCD in which AB//DC, AB = 18 cm, ∠B = ∠C = 90°, CD = 12 cm and AD = 10 cm.
Answer:
In trapezium ABCD,
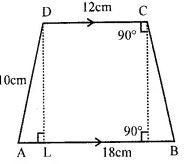
AB || DC, AB = 18 cm
∠B = ∠C = 90°, CD = 12 cm and AD = 10 cm.
Area of trapezium ABCD
Draw DL ⊥ AB
∴ AL = 18 – 12 = 6 cm
AL = BC
Exe – 20 D ICSE Class-8 Mathematics Area of Trapezium and a Polygon
Question 1.
Find the radius and area of a circle, whose circumference is :
(i) 132 cm
(ii) 22 m
Answer:
(i)
Circumference of circle = 132 cm
2πr = 132
2×(22/7)×r=132
r=(132×7(/(2×22)
r=21cm
∴ Area of circle = πr²
= (22/7)×21×21
= 1386 cm2
(ii)
circumference of circle = 22 m
∴ 2πr=22
2×(22/7)×r=22
r=(22×7)/(2×22)
r = 7/2
r = 3.5 m
Area of circle = πr²
= (22/7)×3.5×3.5
= 38.5 m2
Question 2.
Find the radius and circumference of a circle, whose area is :
(i) 154 cm2
(ii) 6.16 m2
Answer:
(i)
Area of circle = 154 cm2
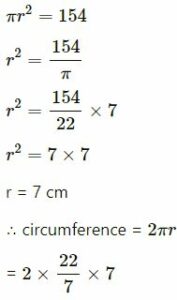
= 44 cm
Hence 7 cm ; 44 cm
(ii)
Area of circle = 6.16 m2
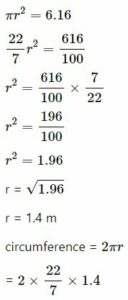
= 8.8 m
∴ 1.4 m; 8.8 m
Question 3.
The circumference of a circular table is 88 m. Find its area.
Answer:
Circumference of circle = 88 m
2πr = 88 m
2×(22/7)×r=88
r=(88×7)/(2×22)
r = 14 m
Area of circle = πr²
= (22/7)×14×14
= 616 m2
Question 4.
The area of a circle is 1386 sq.cm ; find its circumference.
Answer:
Area of circle = 1386 cm2
πr2 = 1386

= 132 m
Question 5.
Find the area of a flat circular ring formed by two concentric circles (circles with same centre) whose radii are 9 cm and 5 cm.
Answer:
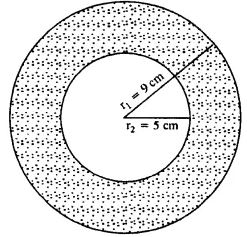
External radius, r1 = 9 cm
Internal radius, r2 = 5 cm

Question 6.
Find the area of the shaded portion in each of the following diagrams :

Answer:
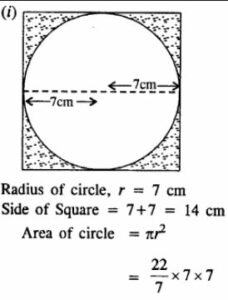
= 154 cm2
Area of square = 14×14
= 196 cm2
∴ Area of shaded portion = 196 – 154
= 42 cm2
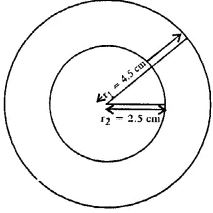
(ii)
Radii of concentric circles are
r1=4.5m
r2=2.5m
Question 7.
The radii of the inner and outer circumferences of a circular running track are 63 m and 70 m respectively. Find :
(i) the area of the track ;
(it) the difference between the lengths of the two circumferences of the track.
Answer:
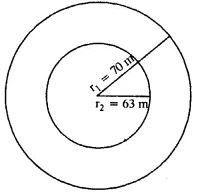
Outer radius, r1 = 70 m
Inner radius, r2 = 63 m

= 2926 m2
Length of outer edge i.e. circumference

Difference between lengths of two circumferences
= 440 – 396
= 44 m
Hence (i) 2926 m2
(ii) 44 m
Question 8.
A circular field cf radius 105 m has a circular path of uniform width of 5 m along and inside its boundary. Find the area of the path.
Answer:
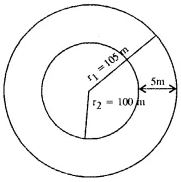
he radius of circular field, r1=105m
Width of path = 50 m
∴ The radius of the inner circle, r2=105-5=100m
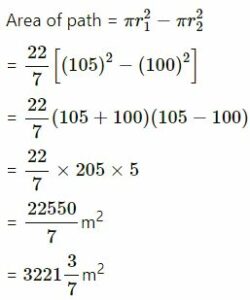
Question 9.
There is a path of uniform width 7 m round and outside a circular garden of diameter 210 m. Find the area of the path.
Answer:
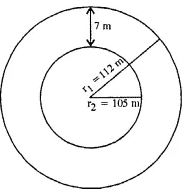
Diameter = 210 m
Radius of inner circle r2 = 105 m
Width = 7m
Radius of outer circle r1 = 105 + 7 = 112 m
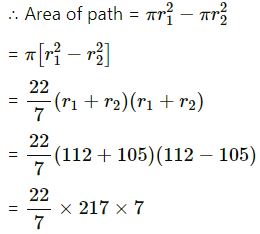
= 4774 m2
Question 10.
A wire, when bent in the form of a square encloses an area of 484 cm2. Find :
(i) one side of the square ;
(ii) length of the wire ;
(iii) the largest area enclosed; if the same wire is bent to form a circle.
Answer:
(i)
Area of Square = 484 cm2
Side of Square = √Area = √484 = 22 cm
(ii)
Perimeter, i.e. length of wire = 4 x 22 = 88 cm
(iii)
Circumference of circle = 88 cm
2πr = 88
2×(22/7)×r=88
r=(88×7)/(2×22)
r = 14 cm
∴ The largest area enclosed = πr²
= (22/7)×14×14
= 616 cm2
Hence (i) 22 cm
(ii) 88 cm
(iii) 616 cm2
Question 11.
A wire, when bent in the form of a square; encloses an area of 196 cm2. If the same wire is bent to form a circle; find the area of the circle.
Answer:
Area of Square = 196 cm2
Side of Square = √Area = √196 = 14 cm
Perimeter of Square = 4 x 14 cm
i.e. length of wire = 56 cm
Circumference of circle = 56 cm
2πr = 56
2×(22/7)×r=56
r=(56×7)/(2×22)
r= (98/11) cm
∴Area of circle enclosed=πr²
= (22/7)×(98/11)×(98/11)
= 2744/11
= 249.45 cm2
Question 12.
The radius of a circular wheel is 42 cm. Find the distance travelled by it in :
(i) 1 revolution ;
(ii) 50 revolutions ;
(iii) 200 revolutions ;
Answer:
(i)
Radius of wheel, r = 42 cm
Circumference i.e. distance travelled in 1 revolution = 2πr = 2 x 22/7 x 42 = 264 cm
(ii)
Distance travelled in 50 revolutions = 264 x 50 = 13200 cm = 132 m
(iii)
Distance travelled in 200 revolutions = 264 x 200 = 52800 cm = 528 m
Hence (i) 264 cm
(ii) 132 m
(iii) 528 m
Question 13.
The diameter of a wheel is 0.70 m. Find the distance covered by it in 500 revolutions. If the wheel takes 5 minutes to make 500 revolutions; find its speed in :
(i) m/s
(ii) km/hr.
Answer:
Diameter = 0.70 m
Radius, r = 0.35 m
Distance covered in 1 revolution, i.e. circumference = 2πr = 2×(22/7)×0.35 = 2.20 m
Distance covered in 500 revolutions = 2.20 x 500 = 1100 m
Time taken = 5 minutes = 5 x 60 = 300 sec.
∴ Speed in m/s = 1100/300
= 11/3=3 (2/3) m/s
Again, Distance = 1100 m
= 1100/1000
= 11/10 km
Time = 5 minutes
= 5/60 hr.

Hence 1100 m, (i) 3 (2/3) m/s
(ii) 13.2 km/hr
Question 14.
A bicycle wheel, diameter 56 cm, is making 45 revolutions in every 10 seconds. At what speed in kilometer per hour is the bicycle travelling ?
Answer:
Diameter = 56 cm
∴ Radius, r = 28 cm
∴ Distance travelled in 1 revolution
i.e. circumference = 2πr=(2×22/7)×28=176 cm
∴ Distance travelled in 45 revolution

= 28.512 km/hr
Question 15.
A roller has a diameter of 1.4 m. Find :
(i) its circumference ;
(ii) the number of revolutions it makes while travelling 61.6 m.
Answer:
Diameter = 1.4 m
r = 1.4/2=0.7 m
∴ Circumference of roller = 2πr
= 2×(22/7)×0.7=4.4 m
Revolutions made in 4.4 m distance = 1
Revolutions made in 1 m distance = 1/4.4
Revolutions made in 61.6 m distance
= (1/4.4)×61.6= (616/44)=14
Hence (i) 4.4 m
(ii) 14
Question 16.
Find the area of the circle, length of whose circumference is equal to the sum of the lengths of the circumferences with radii 15 cm and 13 cm.
Answer:
In a circle
Circumference = Sum of circumferences of two circle of radii 15 cm and 13 cm
Now circumference of first smaller circle = 2πr

Question 17.
A piece of wire of length 108 cm is bent to form a semicircular arc bounded by its diameter. Find its radius and area enclosed.
Answer:
Length of wire = 108 cm
Let r be the radius of the semicircle
πr+ 2r = 108
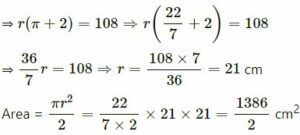
= 693 cm2
Question 18.
In the following figure, a rectangle ABCD enclosed three circles. If BC = 14 cm, find the area of the shaded portion (Take π = 22/7)

Answer
In rectangle ABCD, BC = 14 cm

∴ Radius of each circle = 14/2=7 cm
∴ Length of rectangle = (7+7+7)×2
= 42 cm
Now area of rectangle = l×b=42×14
= 588 cm2
and area of 3 circles = 3×πr²
= 3×(22/7)×7×7=462 cm2
∴ Area of shaded portion
= 588 – 462 = 126 cm2
— End of Area Trapezium and Polygon Solutions :–
Return to – Concise Selina Maths Solutions for ICSE Class -8