Mathematics Formula ICSE 10th Chapter-Wise

Mathematics Formula ICSE 10th Chapter-Wise. Applicable in All publications of ICSE Class-10 Mathematics. Formula of ICSE Mathematics Class 10th is very important to solve the problems to increase self confidence. Visit official Website CISCE for detail information about ICSE Board Class-10 Mathematics.

Mathematics Formula ICSE 10th Chapter-Wise

Important formula for ICSE class 10th mathematics is given below topic wise, we advice to you also view our ICSE class -10 physics formula Chapter Wise

Goods and Service tax (GST)

Goods and Services Tax (abbreviated GST) is an indirect tax levied on the supply of goods

 GST = output GST-  input GST

Before calculation find true selling price.  Market Price/ tag price/ catalogue price are only true selling price if discount is not given.

When discount is given then first of all find out discount value by following formula

discount value = ( MP x Dis % ) / 100

Now Selling Price = MP- Discount value

Banking

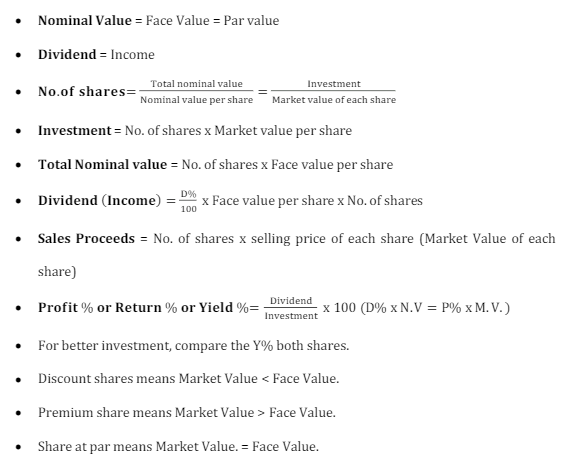
Shares and Dividends

Linear Inequations

we know that equality means = Ex if A = B it is equality

but there are other conditions in which  A is Not equal B then it is called Inequations

There are four Condition of inequation

•  a<b
•  a>b
•  a is less than or equal to b
• a is greater or equal to b

 Law of Inequality

•  add (or subtract) the same number or expression to both sides. no sign change
• any number (or expression) can be transposed from one side of an in equation to the other side with the sign of the transposed number (or expression) changed (-ve
  to -ve or -ve to +ve).
• multiply (or divide) both sides by the same positive number.no sign change
•  However, when you multiply (or divide) by the same negative number, then the symbol of in equation is reversed

How to Solve Inequation

Procedure to solve a linear in equation in one variable
(1) Simplify both sides by removing group symbols and collecting like terms.
(ii) Remove fractions (or decimals) by multiplying both sides by an appropriate fact
(L.C.M. of denominators or a power of 10 in case of decimals).
(1) Isolate all variable terms on one side and all constants on the other side. Collect h
terms when possible.
(17) Make the coefficient of the variable 1.
(v) Choose the solution set from the replacement set.

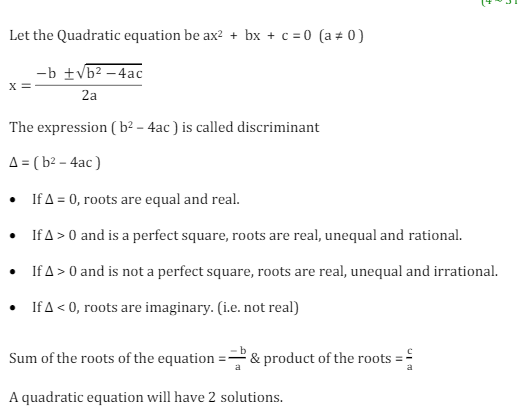
Quadratic Equations 

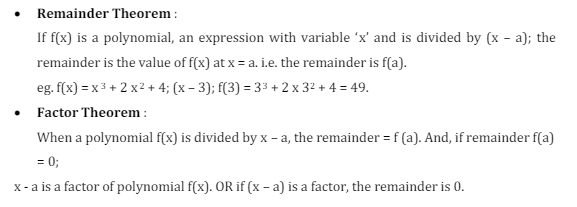
Reminder and Factor Theorem

Ratio and Proportion

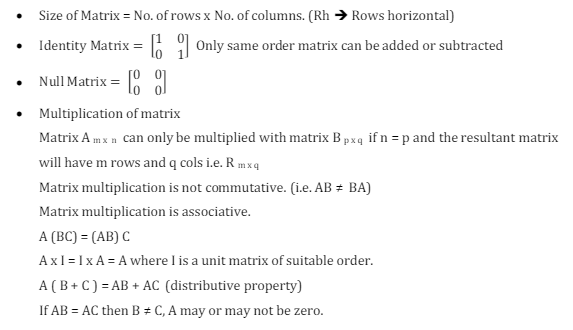
Matrices

 

Arithmetic Progression

• An arithmetic progression is a sequence of numbers in which each term is derived from the preceding term by adding or subtracting a fixed number called the common difference “d”
  For example, the sequence 9, 6, 3, 0,-3, …. is an arithmetic progression with -3 as the common difference. The progression -3, 0, 3, 6, 9 is an Arithmetic Progression (AP) with 3 as the common difference.
• The general form of an Arithmetic Progression is a, a + d, a + 2d, a + 3d and so on. Thus nth term of an AP series is T_n = a + (n – 1) d, where T_n = n^th term and a = first term. Here d = common difference = T_n – T_n-1.
• Sum of first n terms of an AP: S =(n/2)[2a + (n- 1)d]
• 
• The sum of n terms is also equal to the formula where l is the last term.
• T_n = S_n – S_n-1 , where T_n = n^th term
• When three quantities are in AP, the middle one is called as the arithmetic mean of the other two. If a, b and c are three terms in AP then b = (a+c)/2

Geometric Progression

• A geometric progression is a sequence in which each term is derived by multiplying or dividing the preceding term by a fixed number called the common ratio. For example, the sequence 4, -2, 1, – 1/2,…. is a Geometric Progression (GP) for which – 1/2 is the common ratio.
• The general form of a GP is a, ar, ar², ar³ and so on.
• The nth term of a GP series is T_n = ar^n-1, where a = first term and r = common ratio = T_n/T_n-1) .
• The formula applied to calculate sum of first n terms of a GP:
• When three quantities are in GP, the middle one is called as the geometric mean of the other two. If a, b and c are three quantities in GP and b is the geometric mean of a and c i.e. b =√ac
• The sum of infinite terms of a GP series S_∞= a/(1-r) where 0< r<1.
• If a is the first term, r is the common ratio of a finite G.P. consisting of m terms, then the nth term from the end will be = ar^m-n.
• The nth term from the end of the G.P. with the last term l and common ratio r is l/(r^(n-1)) .

Co ordinate Geometry ,Section formula,

 Equation of Straight Line

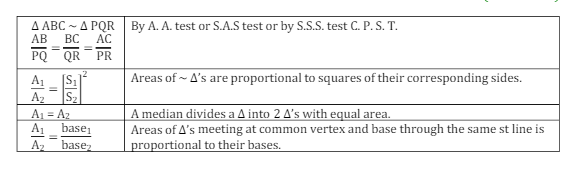
Similarity

(must be focus on map, model word problems)

Locus

Circles

Mensuration

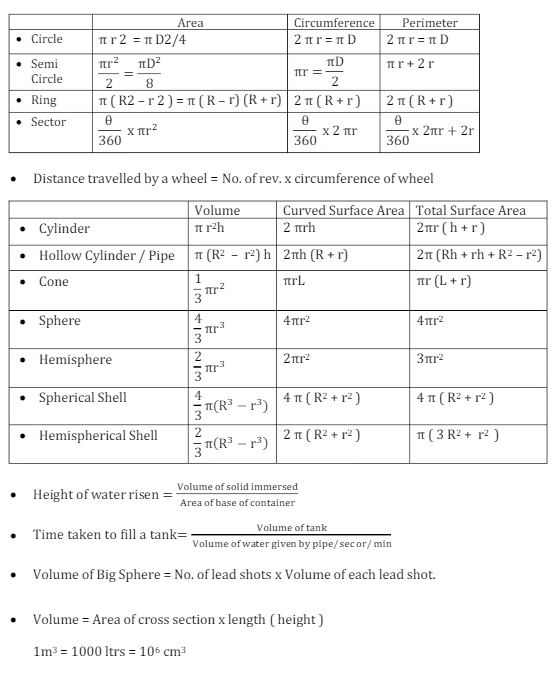
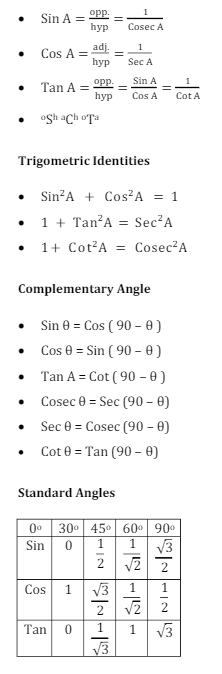
Trigonometry 

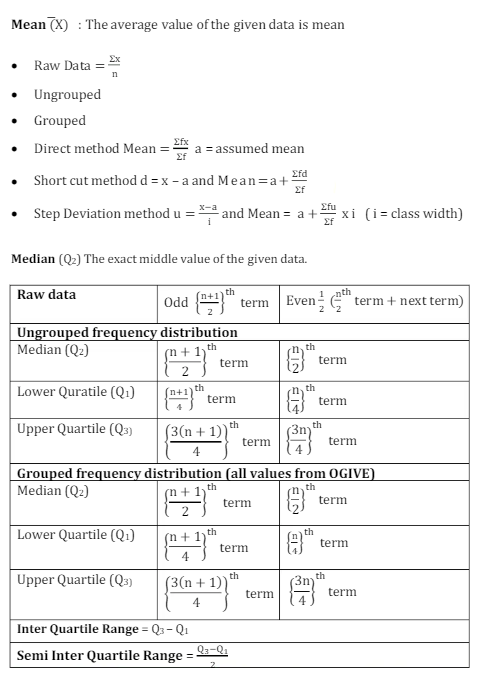
Measures of Central Tendency/ Statistics

Probability

Probability of an Event= Possible out come / Total  Outcome

— : End of ICSE Class 10 Mathematic Formula Chapter Wise :–

Thanks

Return to – ICSE Class-10 Textbook Solution, Syllabus, Notes and Paper

Please Share with your ICSE friends if it is helpful