ML Aggarwal Triangles MCQs Class 9 ICSE Maths Solutions Ch-10. Step by Step Answer of MCQs on Triangles of ML Aggarwal for ICSE Class 9th Mathematics Questions. Visit official website CISCE for detail information about ICSE Board Class-9.
ML Aggarwal Triangles MCQs Class 9 ICSE Maths Solutions Ch-10
| Board | ICSE |
| Subject | Maths |
| Class | 9th |
| Chapter-10 | Triangles |
| Topics | Solution of MCQs Questions |
| Academic Session | 2024-2025 |
Solution of MCQs Questions on Triangles
ML Aggarwal Class 9 ICSE Maths Solutions Ch-10
Question 1. Which of the following is not a criterion for congruency of triangles?
(a) SAS
(b) ASA
(c) SSA
(d) SSS
Answer : Criteria of congruency of two triangles ‘SSA’ is not the criterion. Option (c) is correct
Question 2. In the adjoining figure, AB = FC, EF=BD and ∠AFE = ∠CBD. Then the rule by which ∆AFE = ∆CBD is
(a) SAS
(b) ASA
(c) SSS
(d) AAS
Answer : Option (b) ASA is correct
Question 3. In the adjoining figure, AB ⊥ BE and FE ⊥ BE. If AB = FE and BC = DE, then
(a) ∆ABD ≅ ∆EFC
(b) ∆ABD ≅ ∆FEC
(c) ∆ABD ≅ ∆ECF
(d) ∆ABD ≅ ∆CEF
Answer 3 In ΔABD and ΔFEC,
AB = FE (Given)
∠B = ∠E (Each 90°)
BC = DE (Given)
Add CD both sides, we get
BD = EC
Therefore, by S.A.S. theorem, ΔABD ≅ ΔFEC
Option (b) ∆ABD ≅ ∆FEC ASA is correct
Question 4. In the adjoining figure, AB=AC and AD is median of ∆ABC, then AADC is equal to
(a) 60°
(b) 120°
(c) 90°
(d) 75°
Ans:wer: In the adjoining figure, AB=AC
AD is median of ∆ABC
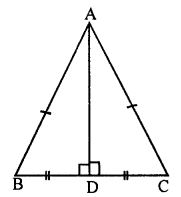
D is mid point
BD = DC
AD ⊥ BC
∠ ADC = 90 degree
Question 5. In the adjoining figure, O is mid point of AB. If ∠ACO = ∠BDO, then ∠OAC is equal to
(a) ∠OCA
(b) ∠ODB
(c) ∠OBD
(d) ∠BOD
Answer : In the adjoining figure, O is mid point of AB
∠ACO = ∠BDO
∠AOC = ∠BDO
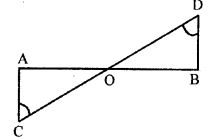
∠OCA = ∠OBD
Option (c) ∠OBD is correct
Question 6. In the adjoining figure, AC = BD. If ∠CAB = ∠DBA, then ∠ACB is equal to
(a) ∠BAD
(b) ∠ABC
(c) ∠ABD
(d) ∠BDA

Answer :
In the adjoining figure, AC = BD
∠CAB = ∠DBA
AB = Ab
Triangle ABC ≅ Triangle ABD
∠ACB = ∠BDA
(d) ∠BDA is correct
Question 7. In the adjoining figure, ABCD is a quadrilateral in which BN and DM are drawn perpendiculars to AC such that BN = DM. If OB = 4 cm, then BD is
(a) 6 cm
(b) 8 cm
(c) 10 cm
(d) 12 cm
Answer : (b) 8 cm is correct
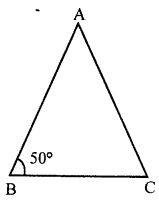
Question 8. In ∆ABC, AB = AC and ∠B = 50°. Then ∠C is equal to
(a) 40°
(b) 50°
(c) 80°
(d) 130°
Answer : ΔABC such that AB = AC and ∠B = 50°
In ΔABC, AB = AC
∠C = ∠B [angles opposite to equal sides are equal]
∠C = 50° [∠B = 50°]
Question 9. In ∆ABC, BC = AB and ∠B = 80°. Then ∠A is equal to
(a) 80°
(b) 40°
(c) 50°
(d) 100°
Answer : Opposite angles of equal sides are equal.
∠A = ∠C = x (Let)

∠x + ∠x + ∠B = 180°
∠x + ∠x + 80° = 180°
2∠x = 180° – 80° = 100°
⇒ x = ∠A = 50°
Answer: (c) 50° is correct
Question 10. In ∆PQR, ∠R = ∠P, QR = 4 cm and PR = 5 cm. Then the length of PQ is
(a) 4 cm
(b) 5 cm
(c) 2 cm
(d) 2.5 cm
Answer : In ∆PQR, ∠R = ∠P, QR = 4 PR = 5 cm
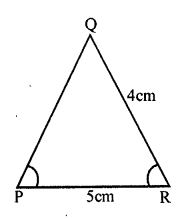
∠P = ∠R
∴ QR = PQ
⇒ PQ = 4 cm
So Option (a) 4 cm is correct
Question 11. In ∆ABC and APQR, AB = AC, ∠C = ∠P and ∠B = ∠Q. The two triangles are
(a) isosceles but not congruent
(b) isosceles and congruent
(c) congruent but isosceles
(d) neither congruent nor isosceles
Answer :
In △ABC and △PQR
∠C=∠P (Given)
∠B=∠Q (Given)
∠A=∠R (Third angle of the triangle)
Thus, △ABC∼△PQR
Also, given, AB=AC
Thus, ∠B=∠C (Isosceles triangle Property)
But, ∠B=∠Q and ∠C=∠P
Hence, ∠Q=∠P
or PR=QR
Thus, both the triangles are Isosceles but not congruent.
Question 12. Two sides of a triangle are of lengths 5 cm and 1.5 cm. The length of the third side of the triangle can not be
(a) 3.6 cm
(b) 4.1 cm
(c) 3.8 cm
(d) 3.4 cm
Answer 12 (d) 3.4 cm is correct answer
Question 13. If a, b, c are the lengths of the sides of a triangle, then
(a) a – b > c
(b) c > a + b
(c) c = a + b
(d) c < A + B
Answer 13 a, b, c are the lengths of the sides of a triangle than a + b> c or c < a + b
(Sum of any two sides is greater than its third side) (d)
(d) c < A + B is correct answer
Question 14. It is not possible to construct a triangle when the lengths of its sides are
(a) 6 cm, 7 cm, 8 cm
(b) 4 cm, 6 cm, 6 cm
(c) 5.3 cm, 2.2 cm, 3.1 cm
(d) 9.3 cm, 5.2 cm, 7.4 cm
Answer : (c) 5.3 cm, 2.2 cm, 3.1 cm is correct answer
Hint We know that sum of any two sides of a triangle is greater than its third side 2.2 + 3.1 = 5.3 ⇒ 5.3 = 5.3 is not possible (c)
Question 15. In ∆PQR, if ∠R> ∠Q, then
(a) QR > PR
(b) PQ > PR
(c) PQ < PR
(d) QR < PR
Answer : (b) PQ > PR is correct answer
Hint In ∆PQR, ∠R> ∠Q
∴ PQ > PR (b)
Question 16. If triangle PQR is right angled at Q, then
(a) PR = PQ
(b) PR < PQ
(c) PR < QR
(d) PR > PQ
Answer : (d) PR > PQ is correct answer
Question 17. If triangle ABC is obtuse angled and ∠C is obtuse, then
(a) AB > BC
(b) AB = BC
(c) AB < BC
(d) AC > AB
Answer 17 (a) AB > BC is correct answer
Question 18. If the lengths of two sides of an isosceles are 4 cm and 10 cm, then the length of the third side is
(a) 4 cm
(b) 10 cm
(c) 7 cm
(d) 14 cm
Answer : (b) 10 cm is correct
Lengths of two sides of an isosceles triangle are 4 cm and 10 cm, then length of the third side is 10 cm
(Sum of any two sides of a triangle is greater than its third side and 4 cm is not possible as 4 + 4 > 10 cm.
— : End of ML Aggarwal Triangles MCQs Class 9 ICSE Maths Solutions :–
Return to :- ML Aggarawal Maths Solutions for ICSE Class-9
Thanks
Please Share with Your Friends