ML Aggarwal Arithmetic and Geometric Progression Exe-9.2 Class 10 ICSE Maths Solutions . We Provide Step by Step Answer of Exe-9.2 Questions for Arithmetic and Geometric Progression(AP GP) as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-10.
ML Aggarwal Arithmetic and Geometric Progression Exe-9.2 Class 10 ICSE Maths Solutions
| Board | ICSE |
| Subject | Maths |
| Class | 10th |
| Chapter-9 | Arithmetic and Geometric Progression |
| Writer / Book | Understanding |
| Topics | Solutions of Exe-9.2 |
| Academic Session | 2024-2025 |
Arithmetic and Geometric Progression Exe-9.2
(ML Aggarwal AP GP Class 10 ICSE Maths Solutions)
Page-173
Question-1 Find the A.P. whose nth term is 7 – 3n Also find the 20th term.
Answer -1
Tn = 7 – 3n
Giving values 1, 2, 3, 4, … to n, we get
T1 = 7 – 3 x 1 = 7 – 3 = 4
T2 = 7 – 3 x 2 = 7 – 6 = 1
T3 = 7 – 3 x 3 = 7 – 9 = -2
T4 = 7 – 3 x 4 = 7 – 12 = -5
T20 = 7 – 3 x 20 = 7 – 60 = -53
A.P. is 4, 1, -2, -5, …
20th term = -53
Question- 2 Find the indicated terms in each of following A.P.s:
(i) 1, 6, 11, 16, …; a20
(ii) – 4, – 7, – 10, – 13, …, a25, an
Answer -2
(i) 1, 6, 11, 16, …
Here, a = 1, d = 6 – 1 – 5
a20 = a + (n – 1 )d
= 1 + (20 – 1) x 5
= 1 + 19 x 5
= 1 + 95
= 96
(ii) – 4, – 7, – 10, – 13, …, a25, an
The first term a = -4
Then, difference d = -7 – (-4) = – 7 + 4 = -3
-10 – (-7) = -10 + 7 = -3
-13 – (-10) = -13 + 10 = -3
Therefore, common difference d = -3
From the formula, an = a + (n – 1)d
So, a25 = a + (25 – 1)d
= -4 + (25 – 1)(-3)
or -4 + (24)-3
= – 4 – 72
= -76
Therefore, a25 = -76
Now, an = a + (n – 1)d
an = -4 + (n – 1)-3
= -4 – 3n + 3
= -1 – 3n
Question -3 Find the nth term and the 12th term of the list of numbers: 5, 2, – 1, – 4, …
Answer -3
5, 2, -1, -4, …
Here, a = 5 d = 2 – 5 = -3
nth term
Tn = a + (n – 1)d
= 5 + (n – 1) (-3)
= 5 – 3n + 3
= 8 – 3n
and 12th term
T12 = a + 11d
= 5 + 11(-3)
= 5 – 33
= -28
Question -4
(i) If the common difference of an A.P. is – 3 and the 18th term is – 5, then find its first term.
(ii) If the first term of an A.P. is – 18 and its 10th term is zero, then find its common difference.
Answer-4
(i) Common difference (d) = -3
T18 = -5
Tn = a + (n – 1)d
So, T18 = a + (18 – 1)d
-5 = a + (18 – 1)(-3)
-5 = a + (17)(-3)
-5 = a – 51
a = 51 – 5
a = 46
Therefore, first term a = 46
(ii) The 10th term = 0
Then, first term a = -18
Tn = a + (n – 1)d
So, T10 = a + (10 – 1)d
0 = -18 + (10 – 1)d
0 = -18 + 9d
9d = 18
d = 18/9
d = 2
Therefore, common difference d = 2
Question -5 Which term of the A.P.
(i) 3, 8, 13, 18, … is 78?
(ii) 18, 15½, 13, … is – 47?
Answer -5
(i) 3, 8, 13, 18, … is 78
Let 78 is nth term
Here, a = 3, d = 8 – 3 = 5
78 = a + (n-1) d
78 = 3 + (n-1) 5
78 – 3 = (n-1) 5
75/5 = n-1
15+1 = n
16 = n
(ii) 18, 15½, 13, … is – 47?
15½ = 31/2
Let -47 as nth term.
The first term a = 18
Then, difference d = 31/2 – 18 = (31 – 36)/2 = -5/2
13 – 31/2 = (26 – 31)/2 = -5/2
Therefore, common difference d = -5/2
Tn = a + (n – 1)d
So, -47 = a + (n – 1)d
-47 = 18 + (n – 1) (-5/2)
-47 = 18 – 5/2n + 5/2
-47 – 18 = -5/2n + 5/2
-65 = -5/2n + 5/2
-65 – 5/2 = – 5/2n
(-130 – 5)/2 = -5/2n
-135/2 = -5/2n
n = (-135/2) × (-2/5)
n = -135/-5
n = 27
AP GP, Exe-9.2,
ML Aggarwal Arithmetic and Geometric Progression Class 10 ICSE Maths Solutions
Question -6
(i) Check whether – 150 is a term of the A.P. 11, 8, 5, 2, …
(ii) Find whether 55 is a term of the A.P. 7, 10, 13, … or not. If yes, find which term is it.
Answer-6
(i) A.P. is 11, 8, 5, 2, …
Here, a = 11, d = 8 – 11 = -3
Let -150 = nth term, then
Tn = a + (n – 1)d
So, -150 = 11 + (n – 1) (-3)
-150 = 11 – 3n + 3
-150 = 14 – 3n
3n = 150 + 14
3n = 164
n = 164/3
n is not a natural number Therefore, – 150 is not a term of the A.P. of 11, 8, 5, 2,
(ii) The first term a = 7
Then, difference d = 10 – 7 = 3
13 – 10 = 3
Then, common difference d = 3
Let us assume 55 as nth term,
Tn = a + (n – 1)d
So, 55 = 7 + (n – 1)3
55 = 7 + 3n – 3
55 = 4 + 3n
3n = 55 – 4
3n = 51
n = 51/3
n = 17
Therefore, 55 is 17th term of the A.P. 7, 10, 13
Question-7
(i) Find the 20th term from the last term of the A.P. 3, 8, 13, …, 253.
(ii) Find the 12th from the end of the A.P. – 2, – 4, – 6, …; – 100.
Answer-7
(i) A.P. is 3, 8, 13, …, 253
12th term from the end
Last term = 253
Here, a = 3, d = 8 – 3 = 5
Therefore, common difference d = 5
Tn = a + (n – 1)d
So, 253 = a + (n – 1)d
253 = 3 + (n – 1)5
253 = 3 + 5n – 5
253 = -2 + 5n
5n = 253 + 2
5n = 255
n = 255/5
n = 51
Therefore, 253 is 51th term.
Now, assume ‘P’ be the 20th term from the last.
Then, P = L – (n – 1)d
= 253 – (20 – 1) 5
= 253 – (19) 5
= 253 – 95
P = 158
Therefore, 158 is the 20th term from the last.
(ii) Let -100 as nth term.
The first term a = -2
Then, difference d = – 4 – (-2) = – 4 + 2 = -2
-6 – (-4) = -6 + 4 = – 2
common difference d = -2
Tn = a + (n – 1)d
So, – 100 = a + (n – 1)d
– 100 = -2 + (n – 1)(-2)
– 100 = -2 – 2n + 2
– 100 = -2n
n = -100/-2
n = 50
Therefore, -100 is 50th term.
let ‘P’ be the 12th term from the last.
Then, P = L – (n – 1)d
= -100 – (12 – 1) (-2)
= -100 – (11) (-2)
= -100 + 22
P = – 78
Hence, -78 is the 12th term from the last of the A.P. – 2, – 4, – 6, …
Question-8 Find the sum of the two middle most terms of the A.P.
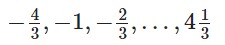
Answer -8
Last term (nth) = 13/3
First term a = -4/3
Then, difference d = -1 – (-4/3) = -1 + 4/3 = (-3 + 4)/3 = 1/3
= -2/3 – (-1) = -2/3 + 1 = (-2 + 3)/3 = 1/3
common difference d = 1/3
Tn = a + (n – 1)d
So, 13/3 = -4/3 + (n – 1)(1/3)
13/3 + 4/3 = 1/3n – 1/3
13/3 + 4/3 + 1/3 = 1/3n
(13 + 4 + 1)/3 = 1/3n
18/3 = 1/3n
6 = 1/3n
n = 6 × 3
n = 18
So, middle term is 18/2 and (18/2) + 1
= 9th and 10th term
Then, a9 + a10 = a + 8d + a + 9d
= 2a + 17d
Now substitute the value of ‘a’ and ‘d’.
= 2(-4/3) + 17(1/3)
= -8/3 + 17/3
= (-8 + 17)/3
= 9/3
= 3
Hence, the sum of the two middle most terms of the A.P is 3.
Question-9 Which term of the A.P. 53, 48, 43,… is the first negative term ?
Answer -9
Let nth term is the first negative term of the A.P. 53, 48, 43, …
Here, a = 53, d = 48 – 53 = -5
.’. Tn = a + (n – 1 )d
= 53 + (n – 1) x (-5)
= 53 – 5n + 5
= 58 – 5n
5n = 58
n= 58/5
n = 11.6 = 12th
Hence, 12th term is the first negative term of the A.P. 53, 48, 43,…
Question-10 Determine the A.P. whose third term is 16 and the 7th term exceeds the 5th term by 12
Answer -10
T3 = 16
T7 – T5 = 12
Let a be the first term and d be the common difference
Tn = a + (n – 1)d
So, T3 = a + 2d = 16 … equation (i)
T7 – T5 = (a + 6d) – (a + 4d) = 12 … equation (ii)
12 = a + 6d – a – 4d
12 = 2d
d = 12/2
d = 6
substitute value of d in equation (i)
Then, T3 = a + 2d
16 = a + 2(6)
a = 16 – 12
a = 4
So, A.P. is 4 + 6 = 10,
10 + 6 = 16,
16 + 6 = 22
Hence, the four term of A.P. are 4, 10, 16, 22, ….
Arithmetic and Geometric Progression Exe-9.2
(ML Aggarwal AP-GP Class 10 ICSE Maths Solutions)
Question -11 Find the 20th term of the A.P. whose 7th term is 24 less than the 11th term, first term being 12.
Answer -11
a= 12 given, and 7th term is 24 less than the 11th term = T11 – T7 = 24
T11 – T7 = (a + 10d) – (a + 6d) = 24
24 = a + 10d – a – 6d
24 = 4d
d = 24/4
d = 6
Now, T20 = a + 19d
Substitute the values of a and d,
T20 = 12 + 19(6)
T20 = 12 + 114
T20 = 126
Question-12 Find the 31st term of an A.P. whose 11th term is 38 and 6th term is 73.
Answer-12
T11 = 38, T6 = 73
Let a be the first term and d be the common difference, then
a + 10d = 38..(i)
a + 5d = 73…(ii)
on Subtracting
(a + 10d) – (a + 5d) = 73 – 38
a + 10d – a – 5d = 35
5d = 35
d = 35/5
d = 7
now, substitute the value of d in equation (i) to find out a, we get
a + 10d = 38
a + 10(7) = 38
a + 70 = 38
a = 38 – 70
a = -32
Therefore, T31 = a + 30d
= -32 + 30(7)
= -32 + 210
= 178
Question-13 If the seventh term of an A.P. is 1/9 and its ninth term is 1/7, find its 63rd term.
Answer -13
T9 = 1/7
T7 = 1/9
Let us assume ‘a’ be the first term and ‘d’ be the common difference,
So, T9 = a + 8d = 1/7 equation (i)
T7 = a + 6d = 1/9 equation (ii)
Subtracting both equation (i) and equation (i),
(a + 6d) – (a + 8d) = 1/9 – 1/7
a + 6d – a – 8d = (7 – 9)/63
-2d = -2/63
d = (-2/63) × (-1/2)
d = 1/63
now, substitute the value of d in equation (ii) to find out a, we get
a + 6(1/63) = 1/9
a = 1/9 – 6/63
a = (7 – 6)/63
a = 1/63
Therefore, T63 = a + 62d
= 1/63 + 62(1/63)
= 1/63 + 62/63
= (1 + 62)/63
= 63/63 = 1
Question-14
(i) The 15th term of an A.P. is 3 more than twice its 7th term. If the 10th term of the A.P. is 41, find its nth term.
(ii) The sum of 5th and 7th terms of an A.P. is 52 and the 10th term is 46. Find the A.P.
Answer -14
(i) T10 = 41
T10 = a + 9d = 41 … equation (i)
T15 = a + 14d = 2T7 + 3
= a + 14d = 2(a + 6d) + 3
= a + 14d = 2a + 12 d + 3
-3 = 2a – a + 12d – 14d
a – 2d = -3 … equation (ii)
, subtracting equation (ii) from (i), we get,
(a + 9d) – (a – 2d) = 41 – (-3)
a + 9d – a + 2d = 41 + 3
11d = 44, d = 44/11, d = 4
substitute the value of d is equation (i) to find a,
a + 9(4) = 41
a + 36 = 41
a = 41 – 36, a = 5
Hence, nth term = Tn = a + (n – 1)d
= 5 + (n – 1)4
= 5 + 4n – 4
= 1 + 4n
(ii) a5 + a7 = 52
(a + 4d) + (a + 6d) = 52
a + 4d + a + 6d = 52
2a + 10d = 52
Divide both the side by 2
a + 5d = 26 … equation (i)
Given, a10 = a + 9d = 46
a + 9d = 46 … equation (ii)
Now subtracting equation (i) from equation (ii),
(a + 9d) – (a + 5d) = 46 – 26
a + 9d – a – 5d = 20
4d = 20 , d = 20/4 , d = 5
Substitute the value of d in equation (i) to find out a,
a + 5d = 26
a + 5(5) = 26
a + 25 = 26
a = 26 – 25, a = 1
Then, a2 = a + d
= 1 + 5 = 6
a3 = a2 + d
= 6 + 5
= 11
a4 = a3 + d
= 11 + 5
= 16
Therefore, 1, 6, 11, 16,… are A.P.
Question -15 If 8th term of an A.P. is zero, prove that its 38th term is triple of its 18th term.
Answer -15
T8 = 0
To prove that T38 = 3 x T18
Let a be the first term and d be the common difference
T8 = a + 7d = 0
T8 = a = -7d
T38 = a + 37d
= -7d + 37d
= 30d
Take, T18 = a + 17d
Substitute the value of a and d,
T18 = -7d + 17d
T18 = 10d
on comparing …… T38 and T18, it is clear that 38th term is triple of its 18th term.
Arithmetic and Geometric Progression (AP GP) Exe-9.2 ML Aggarwal Class 10 ICSE Maths Solutions
Question -16 Which term of the A.P. 3, 10, 17,… will be 84 more than its 13th term?
Answer -16
A.P. is 3, 10, 17, …
Here, a = 3, d – 10 – 3 = 7
T13 = a + 12d
= 3 + 12 x 7
= 3 + 84
= 87
Let , nth term is 84 more than its 13th term
So, Tn = 84 + 87
= 171
Tn = a + (n – 1)d = 171
3 + (n – 1)7 = 171
3 + 7n – 7 = 171
7n – 4 = 171
7n = 171 + 4
7n = 175
n = 175/7
n = 25
Question -17
(i) How many two digit numbers are divisible by 3 ?
(ii) Find the number of natural numbers between 101 and 999 which are divisible by both 2 and 5.
Answer -17
(i) The two digits numbers which is divisible by 3 are
= 12, 15, 18, 21, 24,…..,99.
first number a = 12
Common difference d = 15 – 12 = 3
last number is 99
Tn (last number) = a + (n – 1)d
99 = 12 + (n – 1)3
99 = 12 + 3n – 3
99 = 9 + 3n
99 – 9 = 3n
3n = 90
n = 90/3 , n = 30
(ii) The natural numbers which are divisible by both 2 and 5 are
= 110, 120, 130, 140, ….,990
The above numbers are A.P.
Here a = 110, d = 120 – 110 = 0
an = 990
⇒ a + (n – 1)d = 990
⇒ 110 + (n – 1)(10) = 990
⇒ (n – 1)(10)
= 990 – 110
= 880
⇒ (n – 1) = 880/10 = 88
∴ n = 88 + 1
= 89
Hence, number between 101 and 999 which are divisible by both 2 and 5 are 89.
Question -18 If the numbers n – 2, 4n – 1 and 5n + 2 are in A.P., find the value of n.
Answer-18
given that,
n – 2, 4n – 1 and 5n + 2 are in A.P.
If AP then common deference should be same
(4n – 1 )-(n-2) =(5n + 2)- (4n – 1 )
on solving
4n -1 -n+2 = 5n+2 -4n+1
3n+1 = n+3
3n-n= 3-1
2n = 2, n= 2/2 , n= 1
Question-19 The sum of three numbers in A.P. is 3 and their product is – 35. Find the numbers.
Answer -19
Sum of three numbers which are in A.P. = 3
Their product = -35
Let three numbers which are in A.P.
a – d, a, a + d
a – d + a + a + d = 3
⇒ 3a = 3 , a = 1
product of 3 numbers is – 35
So, (a – d) × (a) × (a + d) = – 35
(1 – d) × (1) × (1 + d) = – 35
12 – d2 = – 35
d2 = 35 + 1
d2 = 36
d = √36
d = ±6
the numbers are (a – d) = 1 – 6 = – 5
a = 1
(a + d) = 1 + 6 = 7
If d = – 6
The numbers are (a – d) = 1 – (-6) = 1 + 6 = 7
a = 1
(a + d) = 1 + (-6) = 1 – 6 = -5
Hence, the numbers -5, 1, 7,… and 7, 1, -5,… are in A.P.
AP GP Exe-9.2 ML Aggarwal Solutions
Page-174
Question-20 The sum of three numbers in A.P. is 30 and the ratio of first number to the third number is 3 : 7. Find the numbers.
Answer -20
Sum of three numbers in A.P. = 30
Ratio between first and the third number = 3 : 7
Let numbers be
a – d, a, a + d, then
a – d + a + a + d = 30
3a = 30
a = 30/3
a = 10
Given ratio 3 : 7 = a – d : a + d
3/7 = (a – d)/(a + d)
(a – d)7 = 3(a + d)
7a – 7d = 3a + 3d
7a – 3a = 7d + 3d
4a = 10d
4(10) = 10d
40 = 10d
d = 40/10
d = 4
the numbers are a – d = 10 – 4 = 6
a = 10
a + d = 10 + 4 = 14
So, 6, 10, 14, … are in A.P.
Question -21 The sum of the first three terms of an A.P.is 33. If the product of the first and the third terms exceeds the second term by 29, find the A.P.
Answer -21
Let the three numbers in A.P. are
a – d, a, a + d
Now, a – d + a + a + d = 33
⇒ 3a = 33
a = 11
It is given that
the product of the first and the third terms exceeds the second term by 29.
(a – d) (a + d) = a + 29
a2 – d2 = 11 + 29
112 – d2 = 40
121 – 40 = d2
d2 = 81
d =√81
d = ±9
If d = 9
Therefore, the numbers are (a – d) = 11 – 9 = 2
a = 11
(a + d) = 11 + 9 = 20
If d = – 9
The numbers are (a – d) = 1 – (-9) = 11 + 9 = 20
a = 11
(a + d) = 11 + (-9) = 11 – 9 = 2
Therefore, the numbers 2, 11, 20,… and 20, 11, 2,… are in A.P.
— : End of ML Aggarwal Arithmetic and Geometric Progression Exe-9.2 Class 10 ICSE Maths Solutions : –
Return to :- ML Aggarwal Solutions for ICSE Class-10
Thanks
Please Share with Your Friends
