ML Aggarwal Data Handling Exe-19.3 Class 8 ICSE Ch-19 Maths Solutions. We Provide Step by Step Answer of Exe-19.3 Questions for Data Handling as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-8.
ML Aggarwal Data Handling Exe-19.3 Class 8 ICSE Maths Solutions
| Board | ICSE |
| Publications | Avichal Publishig Company (APC) |
| Subject | Maths |
| Class | 8th |
| Chapter-19 | Data Handling |
| Writer | ML Aggarwal |
| Book Name | Understanding |
| Topics | Solution of Exe-19.3 Questions |
| Edition | 2023-2024 |
Data Handling Exe-19.3
ML Aggarwal Class 8 ICSE Maths Solutions
Page-338
Question 1. List the outcomes you can see in these experiments.
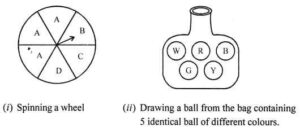
Answer:
(i) Outcomes in spinning wheel = A, A, A, B, C, D.
(ii) Outcomes in drawing a ball from a bag containing 5 identical balls
of different colours, says Red, black, green, blue, yellow.
Question 2. A die is rolled once. Find the probability of getting
(i) an even number
(ii) a multiple of 3
(iii) not a multiple of 3
Answer:
Total outcomes of a die when rolled once:
1, 2, 3, 4, 5, 6 = 6
(i) An even number: 2, 4, 6
i.e, Favourable outcomes = 3
Probability P(E) = 3 / 6
= 1 / 2
(ii) Multiple of 3 = 3, 6
i.e, Favourable outcomes = 2
Probability P(E) = 2 / 6
= 1 / 3
(iii) Not a multiple of 3 = 1, 2, 4, 5
i.e Favourable outcomes = 4
Probability P(E) = 4 / 6
= 2 / 3
Question 3. Two coins are tossed together. Find the probability of getting
(i) two tails
(ii) at least one tail
(iii) no tail
Answer:
When two coins are tossed together, then
Total outcomes = 2 × 2 = 4
i.e. HH, HT, TH, TT
(i) Favourable outcomes of getting two tails = 1
∴ Probability P(E) = 1/4
(ii) Favourable outcomes of getting at least one tail
TH, HT, TT = 3
∴ Probability P(E) = 3/4
(iii) Favourable outcomes of getting No tail: HH = 1
∴ Probability P(E) = 1/4
Question 4. Three coins are tossed together. Find the probability of getting
(i) at least two heads
(ii) at least one tail
(iii) at most one tail.
Answer:
Three coins are tossed together.
Total outcomes = 8
= HHH, HHT, HTH, THH, HTT, TTH, TTT, THT
(i) Favourable outcomes of getting atleast two heads = HHH, HHT, HTH, THH
= 4 in numbers
Probability P(E) = (Number of favourable outcomes) / (Number of possible outcomes)
= 4 / 8
= 1 / 2
(ii) Favourable outcomes of getting atleast one tail = HHT, HTH, HTT, TTT, THH, THT, TTH
= 7 in numbers
Probability P(E) = (Number of favourable outcomes) / (Number of possible outcomes)
= 7 / 8
(iii) Favorable outcomes of getting atmost one tail = HHH, HHT, HTH, THH
= 4 in numbers
Probability P(E) = (Number of favourable outcomes) / (Number of possible outcomes)
= 4 / 8
= 1 / 2
Question 5. A box contains 600 screws, one tenth are rusted. One screw is taken out at random from the box. Find the probability that it is
(i) a rusted screw
(ii) not a rusted screw
Answer:
Rusted screw = (1 / 10) of 600
= (1 / 10) × 600
= 60 seconds
(i) Favourable outcomes of picking rusted screw = 60
Probability P(E) = 60 / 600
= 1 / 10
(ii) Probability (of not rusted screw) = 1 – Probability (of rusted screw)
= 1 – 1 / 10
= (10 – 1) / 10
= 9 / 10
Question 6. A letter is chosen from the word ‘TRIANGLE’. What is the probability that it is a vowel?
Answer:
‘TRIANGLE’
Total number of outcomes = 8
Vowels : I, A, E = 3
∴ Probability P(E) = 3/8
Question 7. A bag contains 5 red, 6 black and 4 white balls. A ball is drawn at random from the bag, find the probability the ball is drawn is
(i) white
(ii) not black
(iii) red or black
(iv) neither red nor black
Answer:
In a bag, there are 5 red, 6 black and 4 white balls.
Total number of outcomes = 5 + 6 + 4=15
(i) Probability of white ball = 4/15
(ii) Probability of not black (5 + 4 = 9) balls = (9/15) = (3/5)
(iii) Probability of red or black ball (5 + 6 = 11)= 11/15
(iv) Probability of ball which is neither red nor black, white ball (4) P(E) = 4/15
Question 8. A box contains 17 cards numbered 1, 2, 3, ……….,17 and are mixed thoroughly. A card is drawn at random from the box. Find the probability that the number on the card is
(i) odd
(ii) even
(iii) prime
(iv) divisible by 3
(v) divisible by 2 and 3 both
Answer:
A box contains 17 cards numbered 1 to 17
Total number of outcomes = 17
(i) Card bearing odd number (1, 3, 5, 7, 9, 11, 13, 15, 17) = 9
∴Probability P(E) = 9/17
(ii) Even number (2, 4, 6, 8, 10, 12, 14, 16) = 8
∴Probability P(E) = 8/17
(iii) Prime numbers {2, 3, 5, 7, 11, 13, 17) = 7
∴Probability P(E) = 7/17
(iv) Numbers divisible by 3 = 3, 6, 9, 12, 15 = 5
∴Probability P(E) = 5/17
(v) Number divisible by 2 and 3 both 6, 12 = 2
∴Probability P(E) = 2/17
Question 9. A card is drawn from a well-shuffled pack of 52 cards. Find the probability that the card drawn is:
(i) an ace
(ii) a red card
(iii) neither a king nor a queen
(iv) a red face card or an ace
(v) a card of spade
(vi) non-face card of red colour.
Answer:
Total number of playing cards = 52
One card is drawn
(i) An ace = 4
Probability P(E) = 4 / 52
= 1 / 13
(ii) A red card = 13 + 13 = 26
Probability P(E) = 26 / 52
= 1 / 2
(iii) Neither a king nor a queen
Number of cards = 52 – (4 + 4)
= 52 – 8
= 44
Probability P(E) = 44 / 52
= 11 / 13
(iv) A red face card = 6
Probability P(E) = 6 / 52
= 3 / 26
(v) A card of spade or an ace = 13 + 3
= 16
Probability P(E) = 16 / 52
= 4 / 13
(vi) Non-face card of red colour = 26 – 6
= 20
Probability P(E) = 20 / 52
= 5 / 13
Data Handling Exe-19.3
ML Aggarwal Class 8 ICSE Maths Solutions
Page-339
Question 10. In a lottery, there are 5 prized tickets and 995 blank tickets. A person buys a lottery ticket. Find the probability of his winning a prize.
Answer:
Number of prized tickets = 5
Number of blank tickets = 995
Total number of tickets = 5 + 995 = 1000
Probability of prized ticket
Probability of prized ticket P(E) = (Number of favourable outcomes) / (Number of possible outcomes)
= 5 / 1000
= 1 / 200
The probability of his winning the prize is 1 / 200.
— End of Data Handling Exe-19.3 Class 8 ICSE Maths Solutions :–
Return to : – ML Aggarwal Maths Solutions for ICSE Class -8
Thanks


