ML Aggarwal Probability Ch-Test Class 10 ICSE Maths Solutions Ch-22. We Provide Step by Step Answer of Ch-Test Questions for Probability as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-10.
ML Aggarwal Probability Ch-Test Class 10 ICSE Maths Solutions Ch-22
| Board | ICSE |
| Subject | Maths |
| Class | 10th |
| Chapter-22 | Probability |
| Writer / Book | Understanding |
| Topics | Solutions of Ch-Test |
| Academic Session | 2024-2025 |
Probability Ch-Test
ML Aggarwal Class 10 ICSE Maths Solutions Ch-22
Page 545
Question 1 In a single throw of a die, find the probability of getting
(i) a number greater than 5
(ii) an odd prime number
(iii) a number which is multiple of 3 or 4.
Answer 1
In a single throw of a die
Number of total outcomes = 6 (1, 2, 3, 4, 5, 6)
(i) Numbers greater than 5 = 6 i.e., one number
Probability = 1/6
(ii) An odd prime number 2 i.e., one number
Probability = 1/6
(iii) A number which is a multiple of 3 or 4 which are 3, 6, 4 = 3 numbers
Probability = 3/6 = 1/2
Question 2 A lot consists of 48 mobile phones of which 42 are good, 3 have only minor defects and 3 have major defects. Varnika will buy a phone if it is good but the trader will only buy a mobile if it has no major defect. One phone is selected at random from the lot. What is the probability that it is
(i) acceptable to Varnika?
(ii) acceptable to the trader?
Answer 4
Number of total mobiles = 48,Number of good mobiles = 42,Number having minor defect = 3
and Number having major defect = 3
(i) Acceptable to Varnika = 42
Probability = 42/48 = 7/8
(ii) Acceptable to trader = 42 + 3 = 45
Probability = 45/48 = 15/16
Question 3 A bag contains 5 red, 8 white and 7 black balls. A ball is drawn from the bag at random. Find the probability that the drawn ball is:
(i) red or white
(ii) not black
(iii) neither white nor black
Answer 3
Total number of balls in a bag = 5 + 8 + 7 = 20
(i) Number of red or white balls = 5 + 8 = 13
Probability of red or white ball will be
P(E) = number of favourable outcome/number of possible outcome = 13/20
(ii) Number of ball which are not black = 20 – 7 = 13
Probability of not black ball will be
P(E) =number of favourable outcome/number of possible outcome = 13/20
(iii) Number of ball which are neither white nor black
= Number of ball which are only red = 5
Probability of neither white nor black ball will be
P(E) = number of favourable outcome/number of possible outcome
= 5/20
= 1/4
Question 4 A bag contains 5 white balls, 7 red balls, 4 black balls and 2 blue balls. One ball is drawn at random from the bag. What is the probability that the ball drawn is :
(i) white or blue
(ii) red or black
(iii) not white
(iv) neither white nor black ?
Answer 4
Number of total balls = 5 + 7 + 4 + 2 = 18
Total Number of white balls = 5, number of red balls = 7,number of black balls = 4
and number of blue balls = 2.
(i) Number of white and blue balls = 5 + 2 = 7
Probability of white or blue balls will be
P(E) =number of favourable outcome/number of possible outcome = 7/18
(ii) Number of red and black balls = 7 + 4 = 11
Probability of red or black balls will be
P(E) = number of favourable outcome/number of possible outcome = 11/18
(iii) Number of ball which are not white = 7 + 4 + 2 = 13
Probability of not white balls will be
P(E) = number of favourable outcome/number of possible outcome = 13/18
(iv) Number of balls which are neither white nor black = 18 – (5 + 4) = 18 – 9 = 9
Probability of ball which is neither white nor black will be
P(E) = number of favourable outcome/number of possible outcome = 9/18 = 1/2
Question 5 A box contains 20 balls bearing numbers 1, 2, 3, 4,……, 20. A ball is drawn at random from the box. What is the probability that the number on the ball is
(i) an odd number
(ii) divisible by 2 or 3
(iii) prime number
(iv) not divisible by 10?
Answer 5
In a box, there are 20 balls containing 1 to 20 number
Number of possible outcomes = 20
(i) Numbers which are odd will be,
1, 3, 5, 7, 9, 11, 13, 15, 17, 19 = 10 balls.
Probability of odd ball will be
P(E) =number of favourable outcome/number of possible outcome = 10/20 = 1/2
(ii) Numbers which are divisible by 2 or 3 will be
2, 3, 4, 6, 8, 9, 10, 12, 14, 15, 16, 18, 20 = 13 balls
Probability of ball which is divisible by 2 or 3 will be
P(E) =number of favourable outcome/number of possible outcome = 13/20
(iii) Prime numbers will be 2, 3, 5, 7, 11, 13, 17, 19 = 8
Probability of prime number will be
P(E) = number of favourable outcome/number of possible outcome = 8/20 = 2/5
(iv) Numbers not divisible by 10 will be
1, 2, 3, 4, 5, 6, 7, 8, 9, 11, 12, 13, 14, 15, 16, 17, 18, 19 = 18
Probability of prime number will be
P(E) = number of favourable outcome/number of possible outcome = 18/20 = 9/10
Question 6 Find the probability that a number selected at random from the numbers 1, 2, 3,……35 is a
(i) prime number
(ii) multiple of 7
(iii) multiple of 3 or 5.
Answer 6
Numbers are 1, 2, 3, 4, 5,…..30, 31, 32, 33, 34, 35
Total = 35
(i) Prime numbers are 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31
which are 11
Probability of prime number will be P(E) = number of favourable outcome / number of possible outcome = 11/35
(ii) Multiple of 7 are 7, 14, 21, 28, 35 which are 5
Probability of multiple of 7 will be
P(E) =number of favourable outcome/number of possible outcome = 5/35 = 1/7
(iii) Multiple of 3 or 5 are 3, 5, 6, 9, 10, 12 ,15, 18, 20, 21, 24, 25, 27, 30, 33, 35.
Which are 16 in numbers
Probability of multiple of 3 or 5 will be
P(E) = number of favourable outcome/number of possible outcome= 16/35
Question 7 Cards marked with numbers 13, 14, 15,…..60 are placed in a box and mixed thoroughly. One card is drawn at random from the box. Find the probability that the number on the card is
(i) divisible by 5
(ii) a number which is a perfect square.
Answer 7
Number of cards which are marked with numbers
13, 14, 15, 16, 17,….to 59, 60 are = 48
(i) Numbers which are divisible by 5 will be
15, 20, 25, 30, 35, 40, 45, 50, 55, 60 = 10
Probability of number divisible by 5 will be
P(E) = number of favourable outcome/number of possible outcome = 10/48 = 5/24
(ii) Numbers which is a perfect square are 16, 25, 36, 49 which are 4 in numbers.
Probability of number which is a perfect square will be
P(E) = number of favourable outcome/number of possible outcome = 4/48 = 1/12
Question 8 A box has cards numbered 14 to 99. Cards are mixed thoroughly and a card is drawn at random from the box. Find the probability that the card drawn from the box has
(i) an odd number
(ii) a perfect square number.
Answer 8
Cards in a box are from 14 to 99 = 86
No. of total cards = 86
One card is drawn at random
Cards bearing odd numbers are 15, 17, 19, 21, …, 97, 99
Which are 43
(i) P(E) = number of favourable outcome/number of possible outcome
= 43/86
= 1/2
(ii) Cards bearing number which are a perfect square
= 16, 25, 36, 49, 64, 81
Which are 6
P(E) = number of favourable outcome/number of possible outcome
= 6/86
= 3/43
ML Aggarwal Probability Ch-Test Class 10 ICSE Maths Solutions Ch-22
Question 9 A bag contains 5 red balls and some blue balls. If the probability of drawing a blue ball is four times that of a red ball, find the number of balls in the bags.
Answer 9
Number of red balls = 5
and let number of blue balls = x
Total balls in the bag = 5 + x
and that of red balls = 5/(5+x)
According to the condition,
x/(5+x)=4 x 5/(5+x)=x/(5+x)=20/(5+x)
x ≠ – 5
x = 20
Hence, number of blue balls = 20
and number of balls in the bag = 20 + 5 = 25
Question 10 A bag contains 18 balls out of which x balls are white.
(i) If one ball is drawn at random from the bag, what is the probability that it is white ball?
Answer 10
Total numbers of balls in a bag = 18
No. of white balls = x
(i) One ball is drawn a random
Probability of being a white ball = x/18
(ii) If 2 more white balls an put, then number of white balls = x + 2
and probability is 9/8 times
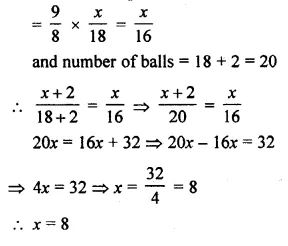
Question 11 A card is drawn from a well-shuffled pack of 52 cards. Find the probability that the card drawn is :
(i) a red face card
(ii) neither a club nor a spade
(iii) neither an ace nor a king of red colour
(iv) neither a red card nor a queen
(v) neither a red card nor a black king.
Answer 11
Number of cards in a pack of well-shuffled cards = 52
(i) Number of a red face card = 3 + 3 = 6
Probability of red face card will be
P(E) =
number of favourable outcome/number of possible outcome
= 6/52 = 3/26
(ii) Number of cards which is neither a club nor a spade = 52 – 26 = 26
Probability of card which’ is neither a club nor a spade will be
P(E) =
number of favourable outcome/number of possible outcome
= 26/52 = 1/2
(iii) Number of cards which is neither an ace nor a king of red colour
= 52 – (4 + 2) = 52 – 6 = 46
Probability of card which is neither ace nor a king of red colour will be
P(E) =
number of favourable outcome/number of possible outcome
= 46/52 = 23/26
(iv) Number of cards which are neither a red card nor a queen are
= 52 – (26 + 2) = 52 – 28 = 24
Probability of card which is neither red nor a queen will be
P(E) =
number of favourable outcome/number of possible outcome
= 24/52 = 6/13
(v) Number of cards which are neither red card nor a black king
= 52 – (26 + 2) = 52 – 28 = 24
Probability of cards which is neither red nor a black king will be
P(E) =number of favourable outcome/number of possible outcome
= 24/52 = 6/13
Question 12 From pack of 52 playing cards, blackjacks, black kings and black aces are removed and then the remaining pack is well-shuffled. A card is drawn at random from the remaining pack. Find the probability of getting
(i) a red card
(ii) a face card
(iii) a diamond or a club
(iv) a queen or a spade.
Answer 12
Total number of cards = 52
Black jacks, black kings and black aces are removed
Now number of cards = 52 – (2 + 2 + 2) = 52 – 6 = 46
One card is drawn
(i) No. of red cards = 13 + 13 = 26
∴Probability = 26/46 = 13/23
(ii) Face cards = 4 queens, 2 red jacks, 2 kings = 8
∴Probability = 8/46 = 4/23
(iii) a diamond on a club = 13 + 10 = 23
∴Probability = 23/46 = 1/2
(iv) A queen or a spade = 4 + 10 = 14
∴Probability = 14/46 = 7/23
Question 13 Two different dice are thrown simultaneously. Find the probability of getting:
(i) sum 7
(ii) sum ≤ 3
(iii) sum ≤ 10
Answer 13
(i) Numbers whose sum is 7 will be (1, 6), (2, 5), (4, 3), (5, 2), (6, 1), (3, 4) = 6
P(E) =number of favourable outcome/number of possible outcome
= 6/36 = 1/6
(ii) Sum ≤ 3
Then numbers can be (1, 2), (2, 1), (1, 1) which are 3 in numbers
∴Probability will be
P(E) =number of favourable outcome/number of possible outcome
= 3/36 = 1/12
(iii) Sum ≤ 10
The numbers can be,
(1, 1), (1, 2), (1, 3), (1, 4), (1, 5), (1, 6),
(2, 1), (2, 2), (2, 3), (2, 4), (2, 5), (2, .6),
(3, 1), (3, 2), (3, 3), (3, 4), (3, 5), (3, 6),
(4, 1), (4, 2), (4, 3), (4, 4), (4, 5), (4, 6),
(5, 1), (5, 2), (5, 3), (5, 4), (5, 5),
(6, 1), (6, 2), (6, 3), (6, 4) = 33
Probability will be
P(E) =number of favourable outcome/number of possible outcome
= 33/36 = 11/12
Question 14 Two dice are thrown together. Find the probability that the product of the numbers on the top of two dice is
(i) 6
(ii) 12
(iii) 7
Answer 14
Two dice are thrown together
Total number of events = 6 × 6 = 36
(i) Product 6 = (1, 6), (2, 3), (3, 2). (6, 1) = 4
Probability = 4/36 = 1/9
(ii) Product 12 = (2, 6), (3, 4), (4, 3), (6, 2) = 4
Probability = 4/36 = 1/9
(iii) Product 7 = 0 (no outcomes)
Probability = 0/36 = 0
— : End ML Aggarwal Probability Ch-Test Class 10 ICSE Maths Solutions Ch-22 :–
Return to :- ML Aggarwal Solutions for ICSE Class-10
Thanks
Please Share with Your Friends


