ML Aggarwal Ratio and Proportion Exe-7.2 Class 10 ICSE Maths Solutions . We Provide Step by Step Answer of Exe-7.2 Questions for Ratio and Proportion as council prescribe guideline for upcoming board exam. Visit official Website CISCE for detail information about ICSE Board Class-10.
ML Aggarwal Ratio and Proportion Exe-7.2 Class 10 ICSE Maths Solutions
| Board | ICSE |
| Subject | Maths |
| Class | 10th |
| Chapter-7 | Ratio and Proportion |
| Writer / Book | Understanding |
| Topics | Solutions of Exe-7.2 |
| Academic Session | 2024-2025 |
Ch-7, Ratio and Proportion Exe-7.2
ML Aggarwal Class 10 ICSE Maths Solutions
Question -1 Find the value of x in the following proportions :
(i) 10 : 35 = x : 42
(ii) 3 : x = 24 : 2
(iii) 2.5 : 1.5 = x : 3
(iv) x : 50 :: 3 : 2
Answer -1
(i) 10 : 35 = x : 42
⇒ 35 × x = 10 × 42
x = (10 × 42) / 35
x = 2 × 6, x = 12
(ii) 3: x = 24: 2
x × 24 = 3 × 2
x = (3 × 2)/ 24
x = ¼
(iii) 2.5: 1.5 = x: 3
1.5 × x = 2.5 × 3
x = (2.5 × 3)/ 1.5
x = 5.0
(iv) x: 50 :: 3: 2
x × 2 = 50 × 3
x = (50 × 3)/ 2
x = 75
Question- 2 Find the fourth proportional to
(i) 3, 12, 15
(ii) 1/3,1/4,1/5
(iii) 1.5, 2.5, 4.5
(iv) 9.6 kg, 7.2 kg, 28.8 kg
Answer -2
(i) Let fourth proportional to
3, 12, 15 be x.
then 3 : 12 :: 15 : x
3 × x = 12 × 15
x = (12 × 15)/ 3
x = 60
(ii) 1/3, 1/4, 1/5
let x is fourth proportional
1/3: 1/4:: 1/5: x
1/3 × x = 1/4 × 1/5
So we get
x = 1/4 × 1/5 × 3/1
x = 3/20
(iii) 1.5, 2.5, 4.5
let x is fourth proportional
1.5: 2.5 :: 4.5: x
1.5 × x = 2.5 × 4.5
x = (2.5 × 4.5)/ 1.5
x = 7.5
(iv) 9.6 kg, 7.2 kg, 28.8 kg
let x be fourth proportional
9.6: 7.2 :: 28.8: x
9.6 × x = 7.2 × 28.8
x = (7.2 × 28.8)/ 9.6
x = 21.6
Question -3 Find the third proportional to
(i) 5, 10
(ii) 0.24, 0.6
(iii) Rs. 3, Rs. 12
(iv) 21/4 and 7.
Answer -3
(i) Let x be the third proportional to 5, 10,
then 5 : 10 :: 10 : x
5 × x = 10 × 10
x = (10 × 10)/ 5 = 20
the third proportional to 5, 10 is 20.
(ii) let x be third proportional to 0.24, 0.6
0.24: 0.6 :: 0.6: x
0.24 × x = 0.6 × 0.6
x = (0.6 × 0.6)/ 0.24 = 1.5
the third proportional to 0.24, 0.6 is 1.5.
(iii) let x be third proportional to Rs. 3 and Rs. 12
3: 12 :: 12: x
3 × x = 12 × 12
x = (12 × 12)/ 3 = 48
the third proportional to Rs. 3 and Rs. 12 is Rs. 48
(iv) let x as the third proportional to 5 ¼ and 7
5 ¼: 7 :: 7: x
21/4 × x = 7 × 7
x = (7 × 7 × 4)/ 21 = 28/3 = 9 1/3
Question- 4 Find the mean proportion of:
(i) 5 and 80
(ii) 1/12 and 1/75
(iii) 8.1 and 2.5
(iv) (a – b) and (a3 – a2b), a ˃ b
Answer -4
(i) let x as the mean proportion of 5 and 80
5: x :: x: 80
x2 = 5 × 80 = 400
x = √400 = 20
so mean proportion of 5 and 80 is 20.
(ii) let x is mean proportion of 1/12 and 1/75
1/12: x :: x: 1/75
x2 = 1/12 × 1/75 = 1/900
x = √1/900 = 1/30
So, mean proportion of 1/12 and 1/75 is 1/30.
(iii) let x is mean proportion of 8.1 and 2.5
8.1: x :: x: 2.5
x2 = 8.1 × 2.5 = 20.25
x = √20.25 = 4.5
So mean proportion of 8.1 and 2.5 is 4.5.
(iv) let x as the mean proportion of (a – b) and (a3 – a2b), a ˃ b
(a – b): x :: x:(a3 – a2b)
x2 = (a – b) (a3 – a2b)
x2 = (a – b) a2 (a – b)
x2 = a2 (a – b)2
x = a (a – b)
Hence, mean proportion is a (a – b).
Question -5 If a, 12, 16 and b are in continued proportion find a and b.
Answer- 5 ∵ a, 12, 16, b are in continued proportion, then
a/12 = 12/16 = 16/b
a/12 = 12/16
16a = 144 (By cross multiplication)
a = 144/16 = 9
and 12/16 = 16/b
12b = 16 × 16 = 256 (By cross multiplication)
b = 256/12 = 64/3 = 21 1/3
Hence, a = 9 and b = 64/3 or 21 1/3.
Ch-7, Ratio and Proportion Exe-7.2
ML Aggarwal Class 10 ICSE Maths Solutions
Page-126
Question- 6 What number must be added to each of the numbers 5, 11, 19 and 37 so that they are in proportion ? (2009)
Answer- 6
Let x be added to 5, 11, 19 and 37 to make them in proportion.
5 + x : 11 + x : : 19 + x : 37 + x
(5 + x) (37 + x) = (11 + x) (19 + x)
185 + 5x + 37x + x2 = 209 + 11x + 19x + x2
185 + 42x + x2 = 209 + 30x + x2
42x – 30x + x2 – x2 = 209 – 185
12x = 24
x = 2
Question- 7 What number should be subtracted from each of the numbers 23, 30, 57 and 78 so that the remainders are in proportion ? (2004)
Answer- 7
Let x be subtracted from each term, then
23 – x, 30 – x, 57 – x and 78 – x are proportional
23 – x : 30 – x : : 57 – x : 78 – x
(23 – x)/ (30 – x) = (57 – x)/ (78 – x)
(23 – x) (78 – x) = (30 – x) (57 – x)
1794 – 23x – 78x + x2 = 1710 – 30x – 57x + x2
x2 – 101x + 1794 – x2 + 87x – 1710 = 0
– 14x + 84 = 0
14x = 84
x = 84/14 = 6
Question-8 The following numbers, K + 3, K + 2, 3K – 7 and 2K – 3 are in proportion. Find k (2019)
Answer-8
(K + 3) / (K + 2) = (3K – 7) / (2K – 3 )
⇒ (K + 3) (2K – 3) = (K + 2) (3K – 7).
⇒ 2K2 – 3K + 6K – 9 = 3K2 – 7K + 6K – 14
⇒ K2 – 4K – 5 = 0
⇒ (K – 5) (K + 1) = 0
⇒ K = 5 or K = – 1
Question- 9 If x + 5 is the mean proportion between x + 2 and x + 9, find the value of x.
Answer -9
∵ x + 5 is the mean proportion between x + 2 and x + 9, then
(x + 5)² = (x + 2) (x + 9)
⇒ x² + 10x + 25 = x² + 11x + 18,
and ⇒ x² + 10x – x² – 11x = 18 – 25
so ⇒ – x = – 7
∵ x = 7 Ans.
Question- 10 What number must be added to each of the numbers 16, 26 and 40 so that the resulting numbers may be in continued proportion?
Answer -10
Let x be added to each number then
16 + x, 26 + x and 40 + x
are in continued proportion.
(16 + x)/ (26 + x) = (26 + x)/ (40 + x)
(16 + x) (40 + x) = (26 + x) (26 + x)
640 + 16x + 40x + x2 = 676 + 26x + 26x + x2
640 + 56x + x2 = 676 + 52x + x2
56x + x2 – 52x – x2 = 676 – 640
4x = 36
x = 36/4 = 9
Question -11 Find two numbers such that the mean proportional between them is 28 and the third proportional to them is 224.
Answer- 11
Let the two numbers are a and b.
∵ 28 is the mean proportional
∵ a : 28 : : 28 : b
ab = 282 = 784
Here a = 784/b …… (1)
224 is the third proportional
a: b :: b: 224
b2 = 224a ….. (2)
substituting the value of a in equation (2)
b2 = 224 × 784/b
b3 = 224 × 784
b3 = 175616 = 563
b = 56
substituting the value of b in equation (1)
a = 784/56 = 14
Hence 14 and 56 are the two numbers.
Question- 12 If b is the mean proportional between a and c, prove that a, c, a² + b², and b² + c² are proportional.
Answer- 12
∵ b is the mean proportional between a and c, then,
b² = a × c ⇒ b² = ac …(i)
a, c, a2 + b2 and b2 + c2 are in proportion
a/c = (a2 + b2)/ (b2 + c2)
a (b2 + c2) = c (a2 + b2)
Using equation (1)
a (ac + c2) = c (a2 + ac)
ac (a + c) = a2c + ac2
Here ac (a + c) = ac (a + c)
Question -13 If b is the mean proportional between a and c, prove that (ab + bc) is the mean proportional between (a² + b²) and (b² + c²).
Answer -13
b is the mean proportional between a and c then
b² = ac …(i)
Now if (ab + bc) is the mean proportional
(ab + bc)2 = (a2 + b2) (b2 + c2)
Consider LHS = (ab + bc)2
on Expanding
= a2b2 + b2c2 + 2ab2c
equation (1)
= a2 (ac) + ac (c)2 + 2a. ac. c
= a3c + ac3 + 2a2c2
by Taking ac as common
= ac (a2 + c2 + 2ac)
= ac (a + c)2
RHS = (a2 + b2) (b2 + c2)
Using equation (1)
= (a2 + ac) (ac + c2)
Taking common
= a (a + c) c (a + c)
= ac (a + c)2
Hence, LHS = RHS
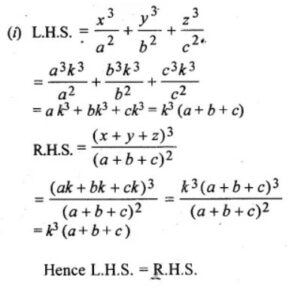
Question -14 If y is mean proportional between x and z, prove that xyz (x + y + z)³ = (xy + yz + zx)³.
Answer- 14
∵ y is the mean proportional between
x and z, then
y² = xz …(i)
LHS = xyz (x + y + z)3
= xz. y (x + y + z)3
Using equation (1)
= y2 y (x + y + z)3
= y3 (x + y + z)3
= [y (x + y + z)]3
= (xy + y2 + yz)3
Using equation (1)
= (xy + yz + zx)3
= RHS
Question -15 If a + c = mb and 1/b + 1/d = m/c, prove that a, b, c and d are in proportion.
Answer -15
a + c = mb and 1/b + 1/d = m/c
a + c = mb
Dividing the equation by b
a/b + c/d = m ……. (1)
1/b + 1/d = m/c
Multiplying the equation by c
c/b + c/d = m …… (2)
Using equation (1) and (2)
a/b + c/b = c/b + c/d
So we get
a/b = c/d
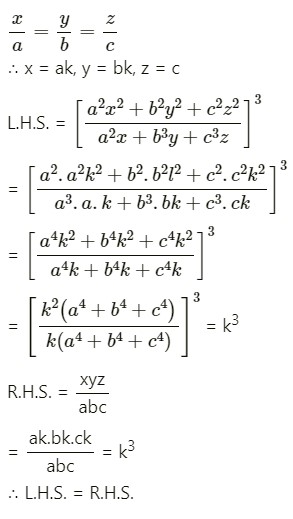
Question- 16 If x/a = y/b = z/c, prove that
(i) x³/a² +y³/b² +z³/c² =(x+y+z)³/(a+b+c)²
(ii)
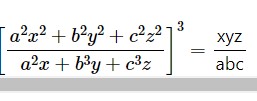
(iii)
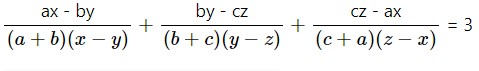
Answer -16
(i) let x/a = y/b = z/c = k ∴ x = ak, y = bk, z = ck
(ii)
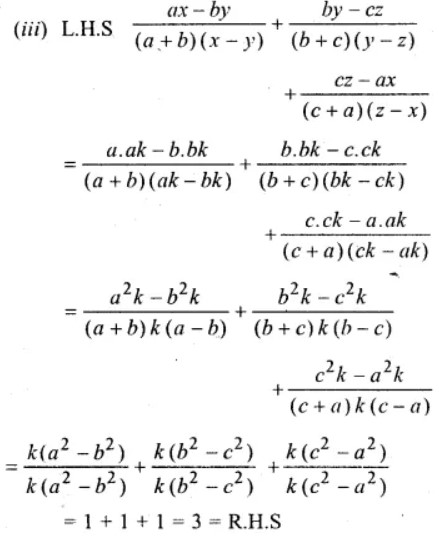
Question- 17 If a/c=c/d=c/f prove that :
(i) (b² + d² + f²) (a² + c² + e²) = (ab + cd + ef)²
(ii) (a³+c³)² /(b³+d³)² =(e³)² / (f³)²
(iii) a²/b² +c²/d² +e²/f² =ac/bd +ce/df +ae/df
(iv) bdf[(a+b)/b +(c+d)/d +(c+f)/f]³ =27(a+b)(c+d)(e+f)
Answer -17
a/b = c/d = e/f = k
a = bk, c = dk, e = fk
(i) LHS = (b2 + d2 + f2) (a2 + c2 + e2)
= (b2 + d2 + f2) (b2k2 + d2k2 + f2k2)
Taking out the common terms
= (b2 + d2 + f2) k2 (b2 + d2 + f2)
= k2 (b2 + d2 + f2)
RHS = (ab + cd + ef)2
= (b. kb + dk. d + fk. f)2
So we get
= (kb2 + kd2 + kf2)
Taking out common terms
= k2 (b2 + d2 + f2)2
Therefore, LHS = RHS.
(ii)
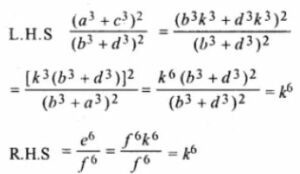
(iii)
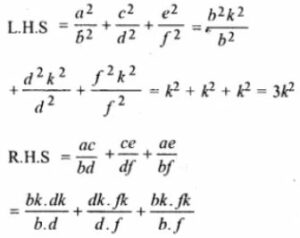
= k²+ k²+ k²
=3k²
(iv)

= bdf(k + 1 + k + 1 + k + 1)3
= bdf(3k + 3)3 = 27bdf(k + 1)3
R.H.S. = 27(a + b)(c + d)(e + f)
= 27(bk + b)(dk + d)(fk + f)
= 27b(k + 1)d(k + 1)f(k + 1)
= 27bdf(k + 1)3
∴ L.H.S. = R.H.S.
Question -18 If ax = by = cz; prove that
x² / yz + y² / zx + z² / xy =bc / a² + ca / b² + ab / c²
Answer -18
Let ax = by = cz = k
x = k/a, y = k/b, z = k/c

Question- 19 If a, b, c and d are in proportion, prove that:
(i) (5a + 7b) (2c – 3d) = (5c + 7d) (2a – 3b)
(ii) (ma + nb) : b = (mc + nd) : d
(iii) (a4 + c4) : (b4 + d4) = a2 c2 : b2 d2.
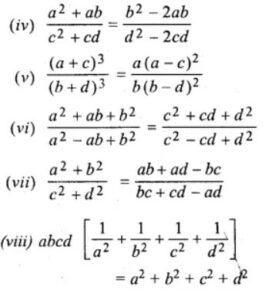
Answer -19
a, b, c, d are in proportion
Consider a/b = c/d = k
a = b, c = dk
(i) LHS = (5a + 7b) (2c – 3d)
Substituting the values
= (5bk + 7b) (2dk – 3d)
= k (5b + 7b) k (2d – 3d)
= k2 (12b) (-d)
= – 12 bd k2
RHS = (5c + 7d) (2a – 3b)
Substituting the values
= (5dk + 7d) (2kb – 3b)
= k (5d + 7d) k (2b – 3b)
= k2 (12d) (-b)
= – 12 bd k2
Therefore, LHS = RHS.
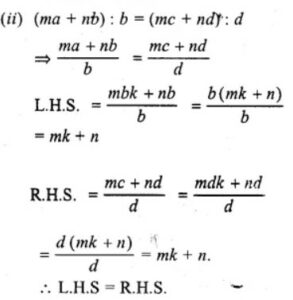
Therefore, LHS = RHS.
(iii)( a4 + c4): (b4 + d4) = a2c2: b2d2
L.H.S.= (a4 + c4)/ (b4 + d4)
= (k4b4 + k4d4) / (b4 + d4)
= k4 (b4 + d4) / (b4 + d4)
= k4
R.H.S. =a2c2/ b2d2
= k2b2 . k2d2 / b2d2
= k4
Hence L.H.S. = R.H.S.
(iv)
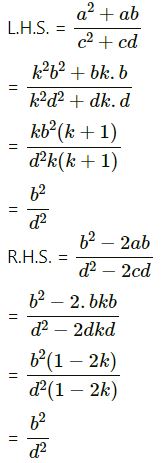
Hence L.H.S. = R.H.S.
(v) ∵ a, b, c, d are in proportion
ab=cd = k(say)
a = bk, c = dk.
L.H.S. = (a+c)³/(b+d)³
= (bk+dk)³ /(b+d)³
= k³(b+d)³ /(b+d)²
= k3
R.H.S. = a(a-c)² /b(b-d)²
= bk(bk-dk)²/ b(b-d)²
= bk.k²(b-d)²/b(b-d)²
= k3
∴ L.H.S. = R.H.S.
(vi)
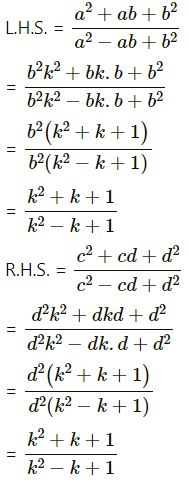
∴ L.H.S. = R.H.S.
(vii)
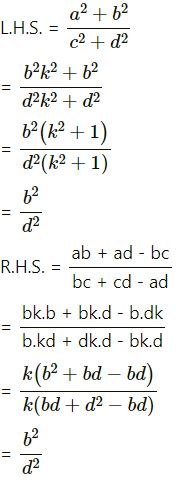
∴ L.H.S. = R.H.S.
(viii)
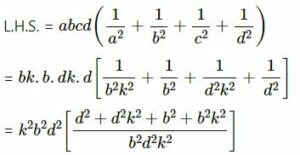
= d2(1 + k2) + b2(1 + k2)
= (1 + k2)(b2 + d2)
R.H.S. = a2 + b2 + c2 + d2
= b2k2 + b2 + d2k2 + d2
= b2(k2 + 1) + d2(k2 + 1)
= (k2 + 1)(b2 + d2)
∴ L.H.S. = R.H.S.
Ch-7, Ratio and Proportion Exe-7.2
ML Aggarwal Class 10 ICSE Maths Solutions
Page-127
Question- 20 If x, y, z are in continued proportion, prove that:(x+y)²/(y+z)² =x/z. (2010)
Answer -20
x, y, z are in continued proportion
Let x/y = y/z = k
Then y = kz
x = yk = kz × k
= k2z
Now L.H.S = (x + y)2/(y + z)2
= (k2z + kz)2/(kz + z)2
= {kz(k + 1)}2/{z(k + 1)}2
= {k2z2(k + 1)2}/{z2(k + 1)2}
= k2
R.H.S. = x/z = k2z/z
= k3
∴ L.H.S. = R.H.S.
Question- 21 If a, b, c are in continued proportion, prove that:
(pa² + qab +rb²)/(pb² + qbc + rc²)=a/c
Answer -21
Given a, b, c are in continued proportion (pa2 + qab + rb2)/(pb2 + qbc + rc2) = a/c
Let a/b = b/c = k
⇒ a = bk and b = ck …(i)
⇒ a = (ck)k = ck2 [Using (i)]
and, b = ck
L.H.S = a/c = ck2/c = k2
R.H.S. = {p(ck2)2 + q(ck2)ck + r(ck)2}/(p(ck)2 + q(ck)c + rc2)
= (pc2k4 + qc2k3 + rc2k2)/(pc2k2 + qc2k + rc2)
= (c2k2)/c2[(pk2 + qk + r)/(pk2 + qk + r)]
= k2 …(iii)
From (ii) and (iii), L.H.S. = R.H.S
∴ b = ck, a = bk, ckk = ck2
(i) L.H.S = (a + b)/(b + c)
= (a + b)/(b + c)
= (ck2 + ck)/(ck + c)
= {ck(k + 1)}/{c(k + 1)}
= k
R.H.S = {a2(b – c)}/{b2(a – b)}
= (ck2)2(ck – c)}/{(ck)2(ck2 – ck)}
= {c2k4c(k – 1)}/{c2k2 ck(k – 1)}
Question- 22 If a, b, c are in continued proportion, prove that:
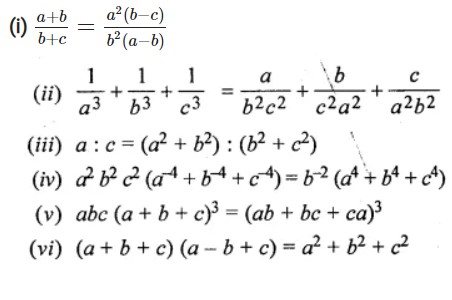
Answer -22
(i) As a, b, c are in continued
Let a/b = b/c = k
= {c3k4(k – 1)}/{c3k3(k – 1)}
= k
∴ L.H.S. = R.H.S
(ii) L.H.S = 1/a3 + 1/b3 + 1/c3
= 1/(ck2)3 + 1/(ck)3 + 1/c3
= 1/(c3k6) + 1/(c3k3) + 1/c3
= 1/c3[1/k6 + 1/k3 + 1/1]
R.H.S = a/(b2c2) + b/(c2a2)+ c/(a2b2)
= (ck2)/(ck2)c2 + ck/{(c2(ck2)2} + c/(ck2)2 (ck)2
= {ck2/(ck2)c2} + {ck/c2(ck2)2} + {c/(ck2)2 (ck)2}
= ck2/(c4k2) + ck/(c4k4) + c/(c4k6)
= 1/c3 + 1/(c3k3) + 1/(c3k6)
= 1/c3[1 + 1/k3 + 1/k6]
= 1/c3[1/k6 + 1/k3 + 1]
∴ L.H.S. = R.H.S.
(iii) a : c = (a2 + b2) : (b2 + c2)
⇒ a/c = (a2 + b2)/(b2 + c2)
L.H.S = a/c = (ck2)/c = k2
R.H.S. = {(ck2)2 + (ck)2}/(c2k2 + c2)
= (c2k4 + c2k2)/(c2k2 + c2)
= {c2k2(k2 + 1)}/{c2(k2 + 1)}
= k2
∴ L.H.S. = R.H.S.
(iv) L.H.S.= a2b2c2 (a-4 + b-4 + c-4)
= a2b2c2[1/a4 + 1/b4 + 1/c4]
= (a2b2c2)/a4 + (a2b2c2)/b4 + (a2b2c2)/c4
= (b2c2)/a2 + (c2a2)/b2 + (a2b2)/c2
= {(ck)2.c2}/(ck2)2 + {c2(ck2)2}/(ck)2 + {(ck2)2(ck)2}/c2
= (c2k2.c2)/(c2k4) + (c2.c2k4)/(c2k2) + (c2k4.c2k2)/c2
= c2/k2 + (c2k2)/1 + (c2k6)/1
= c2[1/k2 + k2 + k6]
= c2\k2[1 + k4 + k8]
R.H.S = b-2[a4 + b4 + c4]
= 1/b2[a4 + b4 + c4]
= 1/(ck)2 [(ck2)4 + (ck)4 + c4]
= 1/(c2k2)[c4k8 + c4k4 + c4]
= c4/(c2k2)[k8 + k4 + 1]
= c2/k2 [1 + k4 + k8]
∴ L.H.S. = R.H.S.
(v) L.H.S. = abc (a + b + c)3
= ck2.ck.c[ck2 + ck + c]3
= c3k3[c(k2 + k + 1)]3
= c2k3.c3.(k2 + k + 1)3
= c6k3(k2 + k + 1)3
R.H.S = (ab + bc + ca)3
= (ck2.ck + ck.c + c.ck2)3
= (c2k3 + c2k + c2k2)3
= (c2k3 + c2k2 + c2k)3
= [c2k(k2 + k + 1)]3
= c6k3(k2 + k + 1)3
∴ L.H.S. = R.H.S.
(vi) L.H.S. = (a + b + c) (a – b + c)
= (ck2 + ck + c)(ck2 – ck + c)
= c(k2 + k + 1)c(k2 – k + 1)
= c2(k2 + k + 1)(k2 – k + 1)
= c2(k4 + k2 + 1)
R.H.S = a2 + b2 + c2
= (ck2)2 + (ck)2 + (c)2
= c2k4 + c2k2 + c2
= c2(k4 + k2 + 1)
∴ L.H.S. = R.H.S.
Question- 23 If a, b, c, d are in continued proportion, prove that:
Answer- 23 a, b, c, d are in continued proportion
∴ a/b = b/c + c/d = k(say)
∴ c = dk, b = ck = dk.k = dk2,
a = bk = dk2.k = dk3
(i) L.H.S. = (a3 + b3 + c3) / (b3 + c3 + d3)
= {(dk3)3 + (dk2)3 + (dk)3}/{(dk)2)3 + (dk)3 + d3}
= (d3k9 + d3k6 + d3k3)/(d3k6 + d3k3 + d3)
= {d3k3(k6 + k3 + 1)}/{d3(k6 + k3 + 1)}
= k3
R.H.S. = a/d = (dk3)/d = k3
∴ L.H.S. = R.H.S.
(ii) L.H.S = (a2 – b2)(c2 – d2)
= [(dk3)2 – (dk2)2][(dk)2 – d2]
= (d2k6 – d2k4)(d2k2 – d2)
= d2k4(k2 – 1)d2(k2 – 1)
= d4k4(k2 – 1)2
R.H.S. = (b2 – c2)2
= [(dk2)2 – (dk)2]2
= [d2k4 – d2k2]2
= [d2k2(k2 – 1)]2
= d4k4(k2 – 1)1
∴ L.H.S. = R.H.S.
(iii) L.H.S = (a + d)(b + c) – (a + c)(b + d)
= (dk3 + d)(dk2 + dk) – (dk3 + dk)(dk2 + d)
= d(k3 + 1)dk(k + 1) – dk(k2 + 1)d
= d2k(k + 1)(k3 + 1) – d2k(k2 + 1)(k2 + 1)
= d2k[k4 + k3 + k + 1 – k4 – 2k2 – 1]
= d2k [k3 – 2k2 + k]
= d2k2 [k2 – 2k + 1]
= d2k2(k – 1)2
R.H.S. = (b – c)2
= (dk2 – dk)2
= d2k2(k – 1)2
∴ L.H.S. = R.H.S.
(iv) a : d = triplicate ratio of (a – b) : (b – c)
= (a – b)3 : (b – c)3
L.H.S. = a : d = a/d = dk3/d = k3
R.H.S = (a – b)3/(b – c)3
= {(dk3 – dk2)3}/(dk2 – dk)3
= d3k6(k – 1)3/d3k3(k – 1)3
= k3
∴ L.H.S. = R.H.S.
(v) L.H.S. = {(a – b) / c + (a – c)/b}2 – {(d – b)/c + (d – c)/b}2
= {(dk3 – dk2)/dk} + {(dk3 – dk)/dk2}2 – {(d – dk2)/dk + (d – dk)/dk2}2
= {(dk2(k – 1)/dk + dk(k2 – 1)/dk2)}2 – {d (1 – k2)/dk + d(1 – k)/dk2}2
= {k(k – 1) + (k2 – 1)/k}2 – {(1 – k2)/k + (1 – k)/k2}2
= {k2(k – 1) + (k2 – 1)/k}2 – {(k – k2 + 1 – k)2}/k2
= {(k3 – k2 + k2 – 1)/k}2 – {(k – k3 + 1 – k)/k2}2
= {(k3 – 1)2}/k2 – {(- k3 + 1)2}/k4
= {(k3 – 1)2}/k2 – {(1 – k3)2}/k4
= {(k3 – 1)/k2}2 (1 – 1/k2)
= {(k3 – 1)/k2}2 {(1 – 1/k2)}
= {(k3 – 1)2(k2 – 1)}/k4
= {(k3 – 1)2(k2 – 1)}/k4
= {(k3 – 1)2(k2 – 1)}/k4
R.H.S. = (a – d)2(1/c2 – 1/b2)
= (dk3 – d)2 {(1/(d2k2) – 1/(d2k4)}
= d2(k3 – 1)2 (k2 – 1)/(d2k4)
= {(k3 – 1)2(k2 – 1)}/k4
∴ L.H.S. = R.H.S.
— : End of ML Aggarwal Ratio and Proportion Exe-7.2 Class 10 ICSE Maths Solutions: –
Return to :- ML Aggarwal Solutions for ICSE Class-10
Thanks
Please Share with Your Friends
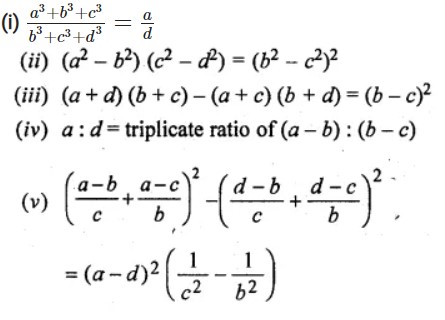



In question number 20, there’s an error in the end part of the proving. Where it should have been k^2, it’s k^3. Plus can u also do it using y^2=xz