Newton Laws of Motion Numerical on Impulse and Conservation of Momentum Class-11 Nootan ISC Physics Solutions Ch-6. Step by step solutions of Kumar and Mittal Physics of Nageen Prakashan as council latest prescribe guideline for upcoming exam. Visit official Website CISCE for detail information about ISC Board Class-11 Physics.

Numericals on Impulse and Law of Conservation of Momentum
Ch-6 Newton Laws of Motion Class-11ISC Nootan Solutions of Kumar and Mittal Physics of Nageen Prakashan
| Board | ISC |
| Class | 11 |
| Subject | Physics |
| Writer | Kumar and Mittal |
| Publication | Nageen Prakashan |
| Chapter-6 | Newton Laws of Motion |
| Topics | Numericals on Impulse and Law of Conservation of Momentum |
| Academic Session | 2024-2025 |
Imp Practice Problems on Impulse and Law of Conservation of Momentum
Ch-6 Newton Laws of Motion Class-11 ISC Nootan Solutions of Kumar and Mittal Physics of Nageen Prakashan
Question-16: A machine gun of mass 5 kg fires 30 bullets, each of mass 50, g, per minute at a speed of 400 m/s. What force must be exerted to keep the machine gun in position?
Answer- F = ΔP / Δt = n( Δmv) / Δt
where n = number of bullet per sec = 30 / 60 = 0.5
=> n x m Δv / Δt
=> 0.5 x 0.05 x 400 / 1
=> 10 N
Question-17: A proton (mass 1.67 × 10^-27 kg) on striking a neutron (mass nearly equal to the proton) forms a deutron. What would be the velocity of the deutron if it is formed by a proton moving left with a velocity of 7.0 x 10^6 m/s and a neutron moving right with a velocity of 4.0 × 10^6 m/s?
Answer- Given – mp = 1.67×10^-27 kg , mn = 1.67×10^-27 kg
up = 7×10^6 m/s , un = -4×10^6 m/s
Let M be mass of deuteron and v be its velocity.
By law of conservation of momentum,
=>pi = pf
=>mp.up + mn.un = M.v
=>mp.up + mn.un = (mp+mn).v
=>(1.67×10^-27 × 7×10^6) + (1.67×10^-27 × -4×10^6) = (1.67×10^-27 +1.67×10^-27) × V
=>v = (7×10^6 – 4×10^6) / 2
=>v = 1.5×10^6 m/s.
Question-18: A 10 g moving body is acted upon by a force of 10 N for 3 µs. Compute the impulse and the change in velocity of the body.
Answer- Impulse = F x Δt
=> 10 x 3 x 10^-6 = 3 x 10^-5 Ns
Impulse = change in momentum = m Δv
=> Δv = 3 x 10^-5 / 10 x 10^-3 = 3 x 10^-3 m/s
Question-19: A hammer weighing 1.5 kg and moving at a speed of 10 m/s strikes a nail, driving it into a wooden block. The duration of the impact is 0.005 s. Find the average impact force and the distance of penetration of the nail into the block.
Answer- F = Δp / Δt = m Δv / Δt
=> 1.5 x (10 – 0) / 0.005 = 3000 N
Distance = average velocity x time
=> [(10 + 0) / 2 ] x 0.005 = 0.025 = 2.5 cm
Question-20: A ball moving with a momentum of 15 kg m/s strikes against a wall at 60° angle and is reflected at the same angle with its initial speed. Find the impulse.
Answer- If we assume it reflects back with same angle then,
Impulse = Change of momentum
=> p – (-p)
=>2p
=> 2 x 15 x 1/2
=> 15 kg m/s
Question-21 A 20 g ball hits a wall at 45° angle with a speed of 15 m/s and rebounds perpendicular to the direction of incidence with same speed. Find the impulse received by the ball.
Answer-
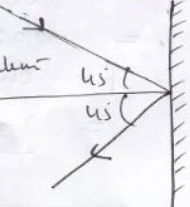
Impulse = change in momentum
Δp = 2 p cos 45
=> 2 x 0.02 x 15 x 1/√2
=> 0.3 √2 kg m/s
Question-22: A 150 g cricket ball moving at a speed of 12 m/s is hit by a bat and turned back at a speed of 20 m/s. The duration of impact is 0.01 s. What is the average force exerted on the ball by the bat?
Answer- v1 = -12 m/s
v2 = +20 m/s [because direction is reversed]
m = 150g = 0.15 kg
t = 0.01 s
Force exerted by the bat on the ball
F = m[v2 – v2]/t = 0.15 [20 – (-12)] / 0.01 = 480 N.
Question-23: A ball of mass 0.5 kg moving with a speed of 2.0 m/s strikes a rigid wall in a direction perpendicular to the wall and is reflected back after a perfectly elastic collision. If the ball has remained in contact with the wall for 0.5 s, calculate the average force exerted on the ball by the wall.
Answer- p1 = 0.5 x 2 = 1 kg m/s
p2 = – 1 kg m/s as direction of motion is reversed.
∴ Δp = Ι-1 -1Ι = 2 kg m/s
∴ F = Δp / Δt = 2 /0.5 = 4 N.
Question-24: A child suspends a steel disc in a vertical plane by means of a thread. Then he shoots bullets on the disc by a toy-gun. If the mass of each bullet be 2.0 g and the bullet striking the disc with a velocity of 11 m/s is returning with the same velocity, then what average force is being exerted on the disc? The child is firing 3 bullets per second.
Answer- initial velocity of the bullet ,u = 11 m/s
Final velocity of the bullet ,v = -11 m/s
mass of the bullet , m = 2 g
mass of the bullet per sec , m = 2 x 3/ 1000 = 0.006 kg
t= 1s
average force of exerted on disc = (m’v – m’u) / t
F = (-11 x 0.006 – 11 x 0.006 ) /1
F = 0.132 N
Question-25: A shell of mass 0.02 kg is fired by a gun of mass 100 kg. The muzzle speed of the shell is 80 m/s. Find the recoil speed of the gun.
Answer- By law of conservation of momentum
=> m1 v1 = m2 v2
or 0.02 x 80 = 100 x v2
=> v2 = 0.02 x 80 / 100 = 0.016 m/s
Question-26: A uniform shell explodes into three equal parts. Two of the parts fly off perpendicular to each other with velocities 9 m/s and 12 m/s. Find the velocity of the third part.
Answer- (1) Let, Total mass of shell = 3 M
Initial velocity of shell before explosion = 0
Mass of first, second and third parts after explosion = M (Given)
Velocity of first parts after explosion = 9j m/s
⇒ …… second parts after explosion = 12j m/s
and velocity of third parts after explosion = →V
(2) Here momentum is conserved before and after explosion.
Initial Momentum = Final Momentum ……….(1)
3M x 0 = M x 9i + M × 12j + M x →v
→v=-9î – 12j ……….(2)
(3) Magnitude of velocity of third parts
|→v| = √9² +12²
On solving
|→v| = 15 m/s.
Question-27: A body of mass 1 kg initially at rest, explodes and breaks into three fragments of masses in the ratio 1 : 1 : 3. The two fragments of equal masses ray off perpendicular to each other with a speed of 30 m/s each. What is the velocity of the heavier fragment?
Answer- Resultant of two perpendicular momentum
=> √(mv)² + (mv)²
=> mv √2
it will be equal to third part momentum
i.e. 3 mv’ = mv√2
=> v’ = v√2/3 = 30 x √2 / 3 = 10 √2
=> 14.1 m/s at angle (90 + 45) = 135°
Question-28: A projectile of mass 50 kg is shot vertically upwards with a velocity of 100 m/s. After 5 seconds the projectile explodes into two fragments, one of which (mass 20 kg) travels vertically up with a velocity of 150 m/s. What will be the velocity of the other fragments at this moment? (g = 9.8 m/s²)
Answer- velocity of projectile after 5 s
=> 100 – 9.8 x 5 = 51 m/s
∴ momentum = 50 x 51 = 2550 kg m/s ↑ (+ve)
Let the 30 kg fragment has speed v, then initial momentum = final momentum
=> 2550 = 20 x 150 + 30 x v
or 2550 – 3000 = 30 v
=> v = -450 / 30 = -15 m/s
negative sign shows that 20 kg fragment will move vertically downward.
Question-29: Determine the magnitude and the direction of the combined momentum of the moving spheres shown in the figure by drawing a vector-diagram. m1 = 2 kg, m2 = 3 kg, v1 = 6 m/s, v2 = 4 m/s.
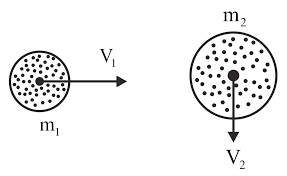
Answer- p1 = m1v1 = 2 x 6 = 12 kg m/s
p2 = m2v2 = 3 x 4 = 12 kg m/s
both are perpendicular to each other
∴ resultant momentum = √ 12² + 12² = 12√2 = 17 kg m/s
again p1 =p2
∴ resultant momentum will be bisector of p1 and p2 i.e. 45°
Question-30: The velocities of two bodies (masses 5 kg and 10 kg in the + x and the + y directions are 4 m/s and 2 m = respectively. They collide and stick together. What the final velocity?
Answer- let there are two bodies move in x+ and y+ directions respectively.
given, mass of body A = 5kg
velocity of body A = 4 i m/s [ along x+ direction]
mass of body B = 10kg
velocity of body B = 2j m/s [along y+ direction]
here, external force = 0
so, from law of conservation of linear momentum,
initial momentum = final momentum
or, 5kg × 4i m/s + 10kg × 2j m/s = (5kg + 10kg) × v
⇒, 20i + 20 j=15 x v
or, v = 4/3(i + j) m/s
hence, magnitude of final velocity = √{(4/3)2 + (4/3)2}
=> 4√2/3 m/s = 1.88 m/s.
—: end of Newton Laws of Motion Numerical on Impulse and Conservation of Momentum Class-11 Nootan Solutions :—
Return to : – Nootan Solutions for ISC Physics Class-11 Nageen Prakashan
Thanks
Please Share with your friends if helpful