Nootan Solutions Gravitation Planets and Satellites ISC Class-11 Physics Nageen Prakashan Chapter-12 Numericals of latest edition. Step by step Solutions of Kumar and Mittal ISC Physics Part-1 Class-11 Nageen Prakashan Numericals Questions. Visit official Website CISCE for detail information about ISC Board Class-11 Physics.
Nootan Solutions Gravitation Planets and Satellites ISC Class-11 Physics Nageen Prakashan
| Class: | 11 |
| Subject: | Physics Part-1 |
| Chapter | Ch:12 Gravitation Planets and Satellites |
| Board | ISC |
| Writer / Publications | Nootan / Nageen Prakashan / Kumar and Mittal |
| Topics | Solved Numericals of page 535, 536 & 537 |
Nootan Solutions Gravitation Planets and Satellites ISC Class-11 Physics Nageen Prakashan
What is Gravitation?
When we talk about gravitation or gravity it is a naturally occurring phenomenon or a force which exists among all material objects in the universe.
What is Kepler’s Law of Planetary Motion?
Kepler’s laws of planetary motion state that:
(a) All planets move in elliptical orbits with the Sun at one of the focal points
(b) The radius vector drawn from the Sun to a planet sweeps out equal areas in equal time intervals. This follows from the fact that the force of gravitation on the planet is central and hence angular momentum is conserved.
(c) The square of the orbital period of a planet is proportional to the cube of the semi-major axis of the elliptical orbit of the planet
UNIVERSAL LAW OF GRAVITATION
Forces of mutual attraction acting between two point particles are directly proportional to the masses of these particles and inversely proportional to the square of the distance between them. The magnitude of the gravitational force is determined by the expression
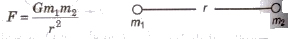
where m1 and m2 are masses of the interacting particles, r = distance between them.
The proportionality constant G is defined as the universal gravitational constant and its value is G = 6.6732*10-11 N m2/kg2.
Forces of gravity are directed along the line joining the interacting particles and are, therefore, called central forces, which is conservative.
The law of universal gravitation in the above form holds not only for two particles but also for
(a) bodies of an arbitrary shape whose dimensions are only a small fraction of the distance between the centers of mass of the bodies.
(b) bodies having a spherically symmetrical distribution of their mass.
The gravitational force is a real force and it is always of attractive nature.
Gravitation is one of the four classes of interactions found in nature.
These are
(i) the gravitational force
(ii) the electromagnetic force
(iii) the strong nuclear force (also called the hadronic force).
(iv) the weak nuclear forces.
Although, of negligible importance in the interactions of elementary particles, gravity is of primary importance in the interactions of objects. It is gravity that holds the universe together.
Newton’s Law of Gravitation (Nootan Solutions Gravitation Planets)
Gravitational force is a attractive force between two masses m1 and m2 separated by a distance r.
The gravitational force acting between two point objects is proportional to the product of their masses and inversely proportional to the square of the distance between them.
Gravitational force. 
where G is universal gravitational constant.
The value of G is 6.67 X 10-11 Nm2 kg-2 and is same throughout the universe.
The value of G is independent of the nature and size of the bodies well as the nature of the medium between them.
Dimensional formula of Gis [M-1L3T-2].
Important Points about Gravitation Force
(i) Gravitational force is a central as well as conservative force.
(ii) It is the weakest force in nature.
(iii) It is 1036 times smaller than electrostatic force and 10’l8times smaller than nuclear force.
(iv) The law of gravitational is applicable for all bodies, irrespective of their size, shape and position.
(v) Gravitational force acting between sun and planet provide it centripetal force for orbital motion.
(vi) Gravitational pull of the earth is called gravity.
(vii) Newton’s third law of motion holds good for the force of gravitation. It means the gravitation forces between two bodies are action-reaction pairs.
Following three points are important regarding the gravitational force
(i) Unlike the electrostatic force, it is independent of the medium between the particles.
(ii) It is conservative in nature.
(iii) It expresses the force between two point masses (of negligible volume). However, for external points of spherical bodies the whole mass can be assumed to be concentrated at its centre of mass.
Note Newton’s law of gravitation holde goods for object lying at uery large distances and also at very short distances. It fails when the distance between the objects is less than 10-9 m i.e., of the order of intermolecular distances.
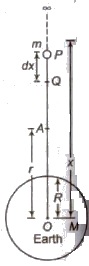
Acceleration Due to Gravity
The uniform acceleration produced in a freely falling object due to the gravitational pull of the earth is known as acceleration due to gravity.
It is denoted by g and its unit is m/s2. It is a vector quantity and its direction is towards the centre of the earth.
The value of g is independent of the mass of the object which is falling freely under gravity.
The value of g changes slightly from place to place. The value of g is taken to be 9.8 m/s2 for all practical purposes.
The value of acceleration due to gravity on the moon is about. one sixth of that On the earth and on the sun is about 27 times of that on the earth.
Among the planets, the acceleration due to gravity is minimum on the mercury.
Relation between g and a is given by
g = Gm / R2
where M = mass of the earth = 6.0 * 1024 kg and R = radius of the earth = 6.38 * 106 m.
Acceleration due to gravity at a height h above the surface of the earth is given by
gh = Gm / (R+h)2 = g (1 – 2h / R)
Gravitational Potential
Gravitational potential at any point in gravitational field is equal the work done per unit mass in bringing a very light body from infinity to that point.
It is denoted by Vg.
Gravitational potential, Vg = W / m = – GM / r
Its SI unit is J / kg and it is a scalar quantity. Its dimensional formula is [L3r-2].
Since work W is obtained, that is, it is negative, the gravitational potential is always negative.
Gravitational Potential Energy
Gravitational potential energy of any object at any point in gravitational field is equal to the work done in bringing it from infinity to that point. It is denoted by U.
Gravitational potential energy U = – GMm / r
The negative sign shows that the gravitational potential energy decreases with increase in distance.
Gravitational potential energy at height h from surface of earth
Uh = – GMm / R + h = mgR / 1 + h/R
Satellite
A heavenly object which revolves around a planet is called a satellite. Natural satellites are those heavenly objects which are not man made and revolve around the earth. Artificial satellites are those neaven objects which are man made and launched for some purposes revolve around the earth.
Time period of satellite
T = 2π √r3 / GM
= 2π √(R + h)3 / g [ g = GM / R2
Near the earth surface, time period of the satellite
T = 2π √R3 / GM = √3π / Gp
T = 2π √R / g = 5.08 * 103 s = 84 min.
where p is the average density of earth.
Artificial satellites are of two types :
1. Geostationary or Parking Satellites
A satellite which appears to be at a fixed position at a definite height to an observer on earth is called geostationary or parking satellite.
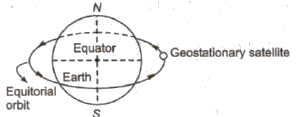
Height from earth’s surface = 36000 km
Radius of orbit = 42400 km
Time period = 24 h
Orbital velocity = 3.1 km/s
Angular velocity = 2π / 24 = π / 12 rad / h
There satellites revolve around the earth in equatorial orbits.
The angular velocity of the satellite is same in magnitude and direction as that of angular velocity of the earth about its own axis.
These satellites are used in communication purpose.
INSAT 2B and INSAT 2C are geostationary satellites of India.
2. Polar Satellites
These are those satellites which revolve in polar orbits around earth. A polar orbit is that orbit whose angle of inclination with equatorial plane of earth is 90°.
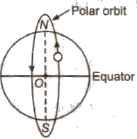
Height from earth’s surface = 880 km
Time period = 84 min
Orbital velocity = 8 km / s
Angular velocity = 2π / 84 = π / 42 rad / min.
There satellites revolve around the earth in polar orbits.
These satellites are used in forecasting weather, studying the upper region of the atmosphere, in mapping, etc.
PSLV series satellites are polar satellites of India.
Orbital Velocity
Orbital velocity of a satellite is the minimum velocity required to the satellite into a given orbit around earth.
Orbital velocity of a satellite is given by
vo = √GM / r = R √g / R + h
where, M = mass of the planet, R = radius of the planet and h = height of the satellite from planet’s surface.
If satellite is revolving near the earth’s surface, then r = (R + h) =- R
Now orbital velocity,
vo = √gR
= 7.92km / h
if v is the speed of a satellite in its orbit and vo is the required orbital velocity to move in the orbit, then
(i) If v < vo, then satellite will move on a parabolic path and satellite falls back to earth.
(ii) If V = vo then satellite revolves in circular path/orbit around earth.
(iii) If vo < V < ve then satellite shall revolve around earth in elliptical orbit.
Chapter-12 Gravitation Planets and Satellites
ISC Physics Class-11 Nootan Solutions Nageen Prakashan Numericals of Kumar and Mittal
Page No 535,536,537
CONTACT FOR LIVE CLASSES- 9335725646
-: End of Gravitation Planets and Satellites Nootan Solutions :-
Return to – Nootan Solutions for ISC Physics Class-11 Nageen Prakashan



Question no. 21(c) is wrong
latest and updated version publishing soon