OP Malhotra Mean Value Theorems S.Chand ISC Class-12 Maths Solutions Ch-10. Step by step Solutions of OP Malhotra SK Gupta, Anubhuti Gangal S.Chand ISC Class-12 Mathematics with Exe-10(a), Exe-10(b), and Chapter Test Questions. Visit official Website CISCE for detail information about ISC Board Class-12 Mathematics.
OP Malhotra Mean Value Theorems S.Chand ISC Class-12 Maths Solutions Ch-10
| Class: | 12th |
| Subject: | Mathematics |
| Chapter : | Ch-10 Mean Value Theorems of Section -A |
| Board | ISC |
| Writer | OP Malhotra, SK Gupta, Anubhuti Gangal |
| Publications | S.Chand Publications 2020-21 |
-: Included Topics :-
Exe-10(a)
Exe-10(b)
Self Revision
Chapter Test
OP Malhotra Mean Value Theorems S. Chand ISC Class-12 Maths Solutions Ch-10
Lagrange’s Mean Value Theorem :-
If a function f is defined on the closed interval [a, b] satisfying the following conditions –
i) The function f is continuous on the closed interval [a, b]
ii)The function f is differentiable on the open interval (a, b)
Then there exists a value x = c in such a way that
f'(c) = [f(b) – f(a)]/(b-a)
This theorem is also known as the first mean value theorem or Lagrange’s mean value theorem.
Mean Value Theorem for Integrals :-
The mean value theorem for integrals is the direct consequence of the first fundamental theorem of calculus and the mean value theorem. This theorem states that if “f” is continuous on the closed bounded interval, say [a, b], then there exists at least one number in c in (a, b), such that
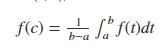
Mean Value Theorem for Derivatives :-
Suppose f is a continuous, real-valued function, described on an arbitrary interval (I) of the real line. If the derivative of function f at each interior point of I exists and is equal to zero, then f is constant in the interior.
Proof: Let (a, b) be an arbitrary open interval in I. By the mean value theorem, there exists a point c in (a,b) such that;
![]()
This indicates that f(a) = f(b). Thus, f is continuous on the interior of I.
Exe-10(a)
OP Malhotra Mean Value Theorems S.Chand ISC Class-12 Maths Solutions Ch-10
Question 1:
f(x) = x² – x – 6 on [-2, 3].
Question 2:
f(x) = x² – 6x + 5, in the interval [1,5].
Question 3:
…………………….
………………………
……………………..
Question 17:
Examine the applicability of Rolle’s theorem on the following function :
……………….
Rolle’s Theorem :-
A special case of Lagrange’s mean value theorem is Rolle’s Theorem which states that:
If a function f is defined in the closed interval [a,b] in such a way that it satisfies the following conditions.
(i) The function f is continuous on the closed interval [a, b]
(ii) Now if f (a) = f (b) , then there exists at least one value of x, let us assume this value to be c, which lies between a and b i.e. (a < c < b ) in such a way that f‘(c) = 0 .
(iii) The function f is differentiable on the open interval (a, b)
Exe-10(b)
OP Malhotra Mean Value Theorems S.Chand ISC Class-12 Maths Solutions Ch-10
Question 1:
(i) f(x) = x(x – 2) in [1, 2]
(ii) f(x) = x² – 2x + 4 in [1, 5]
Question 2:
…………………
………………….
…………………..
Question 12:
f(x) = x (1 – log x); x>0 , show that, (a – b) log c = b(1 – log b) – a (1 – log a); 0 < a < b.
Self Revision Exercise
Mean Value Theorems S.Chand ISC Class-12 Maths Solutions Ch-10
Question 1:
Find ‘c’ of the language’s Mean Value , Theorem,
…………………..
Question 2:
……………………
…………………….
……………………
Question 16:
Verify the condition of Rolle’s Theorem for the function ..
………………………..
Chapter Test
Mean Value Theorems S. Chand ISC Class-12 Maths Solutions Ch-10
Verify of Rolle’s Theorem for the function :
Question 1:
f(x) = x² – 5x + 4 on [1, 4]
Question 2:
…………………..
……………………..
………………………
Question 8:
Using Lagrange’s Mean Value Theorem, find a point on the parabola y = (x + 3)², where the tangent is parallel to the chord joining (-3), 0] in (-4, 1).
-: End of Mean Value Theorems OP Malhotra S. Chand ISC Class-12 Maths Solution :-
Return to :- OP Malhotra S. Chand ISC Class-12 Maths Solutions
Thanks
Please share with your friends


