Geometric Progression Class 10 OP Malhotra Exe-9C ICSE Maths Solutions of Ch-9 questions as latest prescribe guideline for upcoming exam. In this article you would learn about n term of an GP. Visit official Website CISCE for detail information about ICSE Board Class-10 Mathematics.
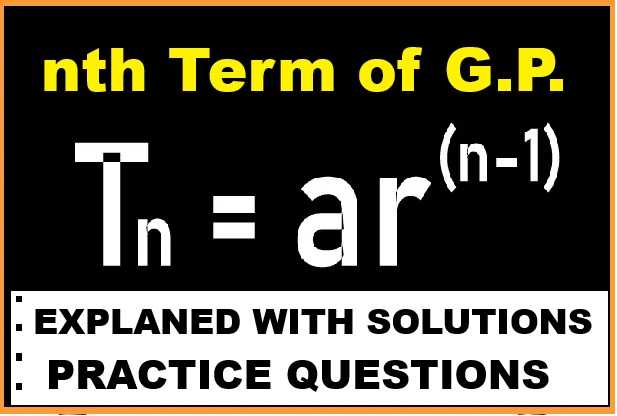
Geometric Progression Class 10 OP Malhotra Exe-9C ICSE Maths Solutions of Ch-9
| Board | ICSE |
| Publications | S Chand |
| Subject | Maths |
| Class | 10th |
| Chapter-9 | Arithmetic and Geometric Progression |
| Writer | OP Malhotra |
| Exe-9C | nth Term of an GP |
| Edition | 2024-2025 |
What is G.P.
Geometric Progression (G.P.) is a geometric sequence where each successive term is the result of multiplying a constant number to its preceding term
nth Term of an GP
The nth term of a GP series is Tn = arn–1, where a = first term and r = common ratio .
Exercise- 9C
( Geometric Progression Class 10 OP Malhotra Exe-9C ICSE Maths Solutions of Ch-9 )
Que-1: Determine whether the following sequences are geometric progressions or not? If yes then find the common ratio.
(i) 27, 9, 3, 1, …
(ii) -1, 2, 4, 8, …
(iii) 2, (1/2), (1/8), (1/32), …
(iv) -12, -6, 0, 6, …
Sol: (i) 27, 9, 3, 1, …
Here, a = 27, r = 9/27 = 1/3, 3/9 = 1/3, ….
∴ It is a G.P. and r = 1/3
(ii) -1, 2, 4, 8, …
Here, a = – 1, r = 2/−1 = – 2, 4/2 = 2, 8/4 = 2
∵ differs
∴ It is not a G.P.
(iii) 2, 12, 18, 132, …
Here, a = 2
r = (1/2)÷2, = (1/2)×(1/2) = 1/4
(1/8)÷(1/2) = (1/8)×(2/1) = 1/4
(1/32)÷(1/8) = (1/32)×(8/1) = 1/4 …
∴ It is G.P. and r = 1/4
(iv) – 12, – 6, 0, 6, …
a = – 12, r = −6/−12 = 1/2
= 0/−6 = 0
∴ It is not G.P.
Que-2: Write the next three terms in each of the GPs given below:
(i) 2, 6, …
(ii) 1/16, – 1/8, …
(iii) 0.3, 0.06, …
Sol: (i) Next 3 terms of G.P.
(i) 2, 6, … (r = 6/2 = 3)
2, 6, 18, 54, 162
(ii) 1/16, – 1/8, ….
(r = (−1/8)÷(1/16) = (−1/8)×(16/1) = – 2)
(1/16), (−1/8), (1/4), (−1/2), 1
(iii) 0.3, 0.06, …
r = 0.06/0.3 = 0.06/0.30 = 1/5 = -.2
0.3, 0.03, 0.012, 0.0024, 0.00048
Que-3: Find the:
(i) 6th term of the G.P. 2, 10, 50 …
(ii) 11th term of the GP. 4, 12, 36 …
Sol: (i) 6th term of the G.P. 2, 10, 50 …
Here, a = 2, r = 10/2 = 5
∴ T6 = ar^n-1 = 2 x 5^(6 – 1)
= 2 x 5^5 = 2 x 3125
= 6250
(ii) 11th term of the G.P. 4, 12, 36 …
Here, a = 4, r = 12/4 = 3
T11 = ar^n-1 = 4 x (3)^(11-1) = 4 x 3^10
= 4 x 243 x 243
= 4 x 59049
= 236196
Que-4: Write the first five terms of the G.P. where nth term is given as:
(i) 4.3^(n-1)
(ii) {5^(n−1)}/{2^(n+1)}
Sol: (i) Tn = 4.3^(n-1).
∴ T1 = 4.3¹ = 4.3° = 4 x l = 4
T2= 4.3² = 4.31 = 4 x 3 = 12
T3 = 4.3³ = 4.3² = 4 x 9 = 36
T4 = 4.3^(4-1) = 4.3³ = 4 x 27 = 108
T5 = 4.3^(5-1) = 4.3^4 = 4 x 81 = 324
∴ Terms are 4, 12, 36, 108, 324
(ii) {5^(n−1)}/{2^(n+1)}
T1 = {5^(1-1)}/{2^(1+1)} = 5^0/2^2 = 1/4
T2 = {5^(2-1)}/{2^(2+1)} = 5^1/2^3 = 5/8
T3 = {5^(3-1)}/{2^(3+1)} = 5^2/2^4 = 25/16
T4 = {5^(4-1)}/{2^(4+1)} = 5^3/2^5 = 125/32
T5 = {5^(5-1)}/{2^(5+1)} = 5^4/2^6 = 625/64
∴ Terms are 1/4, 5/8, 25/16, 125/32, 625/64
Que-5: Write down the nth term of each of the following GPs whose first two terms are given as follows. Also find the term stated besides each G.P.
(i) 12, -36, … sixth term
(ii) 3, (–1/3), …, 8th term
(iii) b²c³, b³c², …, 5th term
Sol: (i) 12, -36, … sixth term -36
Here, a = 12, r = −36/12 = – 3
∴ T6 = ar^(n-1) = 12 x (- 3)^(6-1)
= 12(- 3)^5 = 12 x (- 243) = – 2916
(ii) 3, (–1/3), …, 8th term
Here, a = 3, r =(–1/3) ÷ 3 = (–1/3) x (1/3) = –1/9
∴ T8 = ar^(n-1) = 3(-1/9)^(8-1) = 3(-1/9)^7
(iii) b²c³, b³c², …, 5th term
Here, a = b²c³, r = b³c²/b²c³ = b/c
T5 = ar^(n-1) = (b²c³)(b/c)^(5-1)
T5 = (b²c³)(b/c)⁴
T5 = (b²c³) × (b⁴/c⁴)
T5 = b^6/c
Que-6: Which term of the G.P. 27, -18,12, -8, … is 1024/2187.
Sol: G.P. is 27,-18, 12, -8, … is 1/2
Here, a = 27, r = −18/27 = −2/3
Let 1027/2187 be the nth term, then
an = a.r^(n-1)
1027/2187 = 27(-2/3)^(n-1)
1027/(2187×27) = (-2/3)^(n-1)
{2^10}/{3^10} = (-2/3)^(n-1)
(2/3)^10 = (-2/3)^(n-1)
n-1 = 10
n = 10+1
n = 11.
Que-7: Write the GP. whose 4th term is 54 and the 7th term is 1458.
Sol: In a G.P.
T4 = 54, T7 = 1458
Let a be the first term and r be the common ratio.
∴ T4 = ar^(n-1) = ar³ = 54 … (i)
and T7 = ar^(7-1) = ar^6 = 1458 … (ii)
Dividing, we get
ar^6/ar³ = 1458/54 ⇒ r³ = 27 = (3)³
∴ r = 3
Now, T4 = ar^(4-1)
54 = a(3)³
∴ r = 3
54 = 27a
∴ a = 54/27 = 2
∴ a = 2, r = 3
∴ G.P. will be 2, 6, 18, 54, …
Que-8: The last term of the G.P. : 3, 3√3 , 9,… is 2187. How many terms in all there in the GP.?
Sol: In a G.P. 3, 3√3, 9, …
Last term (l) = 2187
Here, a = 3, r = 3√3/3 = √3
L = arⁿ¯¹
2187 = 3 × ( √3 )ⁿ¯¹
729 = (√3)ⁿ¯¹
3^6 = (√3)ⁿ¯¹ { ∴ 3^6 = 729 }
3^6 = {3}^ (n-1)/2
Now, Compare powers of 3 we have
6 = (n – 1 )/2
12 = ( n -1 )
n = 12 + 1
n = 13
Que-9: Find the value of x + y + z if 1, x, y, z, 16 are in GP.
Sol: 1, x, y, z, 16 are in G.P.
∴ a (first term) = 1
Common ratio (r) = x/1
Ts = 16
T5 = ar^(n – 1)
= ar^(5-1)
16 = ar^4
16 = 1(r)^4
16 = (2)^4
r^4 = (2)^4
By comparing,
r = 2
i.e. the common ratio = 2
Now, r = x/1 = 2/1
i.e. x = 2 … (i)
Also, common ratio
16/z = 2
z = 16/2
z = 8 … (ii)
Also, common ratio
z/y = 2
8/y = 2
y = 8/2
y = 4 … (iii)
As per condition,
From (i), (ii) and (iii)
⇒ x + y + z = 2 + 8 + 4 = 14
Que-10: The third term of a G.P. is 18 and its seventh term is 3*(5/9). Find the tenth term of the G.P.
Sol: In a G.P.
T3 = 18, T7 = 3*(5/9) = 32/9
Let a be the first term and r be the common ratio, then
Tn = ar^(n-1)
T3 = ar^(3-1) = ar² = 18 … (i)
T7 = ar^(7-1) = ar^6 = 50/9 … (ii)
Dividing, we get
ar^6/ar² = 32/(9×18) = 16/81 = (2/3)^4
r^4 = (2/3)^4
r = 2/3
and ar² = 18
a(2/3)² = 18
a(4/9) = 18
a = 18×(9/4) = 81/2
T10 = ar^9
= (81/2)(2/3)^9
= 256/243.
Que-11: The 5th, 8th and 11th terms of a G.P. are P, Q and S respectively. Show that Q² = PS.
Sol: In a G.P.
T5 = P, T8 = Q, T11 = S
To prove : Q² = PS
Let a be the first term and r be the common ratio
∴ T5 = ar^(5-1) = ar^4 = P
T8 = ar^(8-1) = ar^7 = Q
T11 = ar^(11-1)= ar^10 = S
Q² = (ar^7)² = a²r^14
and P x S = ar^4 x ar^10 = a²r^(4+10) = a²r^14
Hence, Q² = P x 5.
— : Geometric Progression Class 10 OP Malhotra Exe-9C ICSE Maths Solutions of Ch-9 :–
Return to : OP Malhotra S Chand Solutions for ICSE Class-10 Maths
Thanks
Please Share with Your Friends


