Representing 3D in 2D ICSE Class-8th Concise Mathematics Selina Solutions Chapter-19. We provide step by step Solutions of Exercise / lesson-19 Representing 3D in 2D for ICSE Class-8 Concise Selina Mathematics.
Our Solutions contain all type Questions with figure explanations of Exe-19 to develop skill and confidence . Visit official Website CISCE for detail information about ICSE Board Class-8 Mathematics.
Representing 3D in 2D ICSE Class-8th Concise Mathematics Selina Solutions Chapter-19
Exercise – 19
Question 1.
If a polyhedron has 8 faces and 8 vertices, find the number of edges in it.
Answer
Faces = 10
Vertices = 8
using Euler’s formula,
F + V – E = 2
10 + 8 – E = 2
-E = 2 – 18
E= 16
Question 2.
If a polyhedron has 10 vertices and 7 faces, find the number of edges in it.
Answer
Vertices = 10
Faces = 7
Using Euler’s formula,
F + V – E = 2
7 + 10 – E = 2
-E = -15
E = 15
Question 3.
(i) a pentagonal pyramid
(ii) a hexagonal prism
Answer
(i)
A pentagonal pyramid
Number of faces = 6
Number of vertices = 6
Number of edges = 10
(ii)
A hexagonal prism
Number of faces = 8
Number of vertices = 12
Number of edges = 18
Question 4.
Verily Euler’s formula for the following three dimensional figures:
Answer
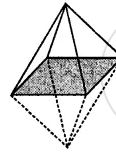
(i)
Number of vertices = 6
Number of faces = 8
Number of edges = 12
Using Euler formula,
F + V – E = 2
8 + 6 – 12 = 2
2 = 2 Hence proved.
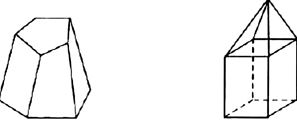
(ii)
Number of vertices = 9
Number of faces = 5
Number of edges = 12
Using, Euler’s formula,
F + V – E = 2
9 + 5 – 12 = 2
2 = 2 Hence proved.
(iii)
Number of vertices = 9
Number of faces = 8
Number of edges = 15
Using, Euler’s formula,
F + V – E = 2
9 + 8 – 15 = 2
2 = 2 Hence proved.
Question 5.
Answer
Number of faces = 8
Number of vertices = 16
Number of edges = 26
Using Euler’s formula
F + V – E
⇒ 8 + 16 – 26 ≠ -2
⇒ -2 ≠ 2
No, a polyhedron cannot have 8 faces, 26 edges, and 16 vertices.
Question 6.
Can a polyhedron have:
(i) 3 triangles only ?
(ii) 4 triangles only ?
(iii) a square and four triangles ?
Answer
(i) No.
(ii) Yes.
(iii) Yes.
Question 7.
Using Euler’s formula, find the values of x,y,z.
| Faces | Vertices | Edges | |
| (i) | x | 15 | 20 |
| (ii) | 6 | y | 8 |
| (iii) | 14 | 26 | z |
Answer
(i)
F + V – E = 2
⇒ x + 15 – 20 = 2
⇒ x – 5 = 2 ⇒ x = 2 + 5 = 7
(ii)
F + V – E = 2
⇒ 15 + y – 26 = 2
⇒ y – 11 = 2
⇒ y = 2 + 11 ⇒ y = 13
(iii)
F + V – E = 2
⇒ 14 + 26 – Z = 2
⇒ -Z = 2 – 40 ⇒ Z = 38
Question 8.
What is the least number of planes that can enclose a solid? What is the name of the solid.
Answer
The least number of planes that can enclose a solid is 4.
The name of the solid is Tetrahedron.
Question 9.
Is a square prism same as a cube?
Answer
Yes, a square prism is the same as a cube.
Question 10.
A cubical box is 6 cm x 4 cm x 2 cm. Draw two different nets of it.
Answer
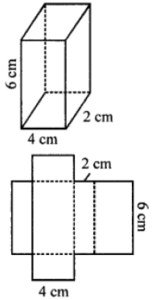
Question 11.
Dice are cubes where the sum of the numbers on the opposite faces is 7. Find the missing numbers a, b and c.
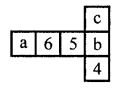
Answer
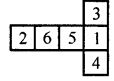
Question 12.
Name the polyhedron that can be made by folding each of the following nets:
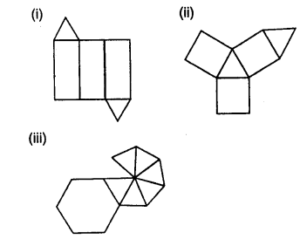
Answer
(i) Triangular prism. It has 3 rectangles and 2 triangles.
(ii) Triangular prism. It has 3 rectangles and 2 triangles.
(iii) Hexagonal pyramid as it has a hexagonal base and 6 triangles.
Question 13.
Draw nets for the following polyhedrons:
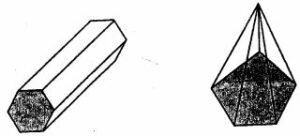
Answer
Net of hexagonal prism:

Net of the pentagonal pyramid:
— End of Representing 3D in 2D ICSE Solutions :–
Return to – Concise Selina Maths Solutions for ICSE Class -8