Simple Linear Equations ICSE Class-6th Concise Selina Maths Solutions Ch-22

Simple Linear Equations ICSE Class-6th Concise Selina Mathematics Solutions Chapter-22  (Including Word Problems) . We provide step by step Solutions of Exercise / lesson-22 Simple Linear Equations (Including Word Problems)  for ICSE Class-6 Concise Selina Mathematics.

Our Solutions contain all type Questions of Exe-22 A, Exe-22 B, Exe-22 C, Exe-22 D and Revision Exercise to develop skill and confidence. Visit official Website  CISCE for detail information about ICSE Board Class-6 .

Simple Linear Equations ICSE Class-6th Concise Selina Mathematics Solutions Chapter-22  (Including Word Problems)

---

–: Select Topics :–

Exe-22 A,

 Exe-22 B,

Exe-22 C,

Exe-22 D,

 Revision Exercise

---

Exercise – 22 A Simple Linear Equations  (Including Word Problems) for ICSE Class-6th Concise Selina Mathematics Solutions

Question- 1.

Solve:
(i) x + 2 = 6

(ii) x + 6 = 2

(iii) y + 8 = 5

(iv) x + 4 = – 3

(v)  y + 2 = – 8

(vi) b + 2.5 = 4.2

(vii)  p + 4.6 = 8.5

(viii) y + 3.2 = – 6.5

(ix) a + 8.9 = – 12.6

(x)…x +2.¹⁄₃..= 5……..

Answer-1

(i) x + 2 = 6

⇒ x = 6 – 2

⇒ x = 4

(ii) x + 6 = 2

⇒ x = 2 – 6

⇒ x = – 4

(iii) y + 8 = 5

⇒ y = 5 – 8

⇒ x = – 3

(iv) x + 4 = – 3

x + 4 = – 3

⇒ x = – 3 – 4

⇒ x = – 7

(v)  y + 2 = – 8

⇒ y = – 8 – 2

⇒ y = – 10

(vi) b + 2.5 = 4.2

⇒ b = 4.2 – 2.5

⇒ b = 1.7

(vii)  p + 4.6 = 8.5

⇒ p = 8.5 – 4.6

⇒ p = 3.9

(viii) y + 3.2 = – 6.5

⇒ y = – 6.5 – 3.2

⇒ y = – 9.7

(ix) a + 8.9 = – 12.6

⇒ a = – 12.6 – 8.9

⇒ a = – 21.5

(x)…x +2.¹⁄₃..= 5……..

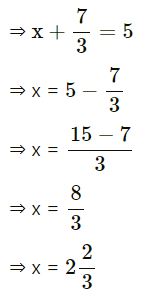
(xi)

(xii)

(xiii)

(xiv)

(xv)

Question -2.

Answer-2

(i) x – 3 = 2

⇒ x = 2 + 3

⇒ x = 5

(ii) m – 2 = – 5

⇒ m = – 5 + 2

⇒ m = – 3

(iii) m – 2 = – 5

⇒ m = – 5 + 2

⇒ m = – 3

(iv) a – 2.5 = – 4

⇒ a = – 4 + 2.5

⇒ a = – 1.5

(v)

(vi)

(vii) p – 5.4 = 2.7

⇒ p = 2.7 + 5.4

⇒ p = 8.1

(viii) x – 1.5 = – 4.9

⇒ x = – 4.9 + 1.5

⇒ x = – 3.4

(ix)

Question -3.

Solve:

(i) 3x = 12

(ii) 2y = 9

(iii) 5z = 8.5

(iv) 2.5 m = 7.5

(v) 3.2 p = 16

(vi) 2a = 4.6

Answer-3:

Question- 4.

Solve:

Answer-4:

Question- 5.

Solve:

Answer-5:

---

Selina Concise Mathematics solutions Simple Linear Equations (Including Word Problems) Exercise – 22 B for ICSE Class-6th 

Question- 1.

Solve:

(i) 2x + 5 = 17

(ii) 3y – 2 = 1

(iii) 5p + 4 = 29

(iv) 4a – 3 = – 27

(v) 2z + 3 = – 19

(vi)  7m – 1 = 20

(vii) 2.4x – 3 = 4.2

(viii) 4m + 9.4 = 5

(ix)  6y + 4 = – 4.4

Answer-1:

Question -2.

Solve:

Answer-2:

Question- 3.

Solve:

(i) 8m -2 = – 10

(ii) 4x + 2x = 3 + 5

(iii) 4x – x + 5 = 8

(iv) 6x + 2 = 2x + 10

(v) 18 – (2a – 12) = 8a

(vi) 3x + 5 + 2x + 6 + x = 4x + 21

(vii) 3.5x – 9 – 3 = x + 1

(viii) 8x + 6 + 2x – 4 = 4x + 8

(ix) m + (3m – 6m) = – 8 – 14

(x)…5x – 14 = x – (24 + 4x)……

Answer-3:

---

Exercise – 22 C  Simple Linear Equations (Including Word Problems) Selina Concise Mathematics solutions for ICSE Class-6

Question- 1.

5 – x = 3
Answer-1:

5 – x = 3

⇒ 5 – 3 = x

⇒ x = 2

Question -2.

2 – y = 8
Answer-2:

2 – y = 8

⇒ 2 – 8 = y

⇒ – 6 = y

⇒ y = – 6

Question -3.

8.4 – x = -2
Answer-3:

8.4 – x = -2

⇒ 8.4 + 2 = x

⇒ x = 10.4

Question -4.

Answer-4:

Question -5.

Answer-5:

Question- 6.

Answer-6:

Question -7.

1.6z = 8
Answer-7:

Question -8.

3a = – 2.1
Answer-8:

Question -9.

Answer-9:

Question -10.

Answer-10:

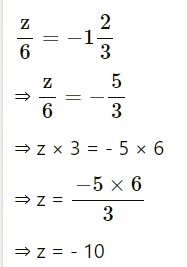
Question- 11.

– 5x = 10
Answer-11:

Question -12.

2.4z = -4.8
Answer-12:

Question- 13.

2y – 5 = -11
Answer-13:

2y – 5 = -11

⇒ 2y = – 11 + 5

⇒ 2y = – 6

⇒ y = –⁶⁄₂

⇒ y = – 3

Question -14.Simple Linear Equations ICSE Class-6th Concise  

2x + 4.6 = 8
Answer-14:

2x + 4.6 = 8

⇒ 2x = 8 – 4.6

⇒ 2x = 3.4

⇒ x = ^3.4⁄₂

⇒ x = ³⁴⁄_{2 x 10}

x = ¹⁷⁄₁₀

x = 1.7

Question- 15.

5y – 3.5 = 10
Answer-15:

5y – 3.5 = 10

⇒ 5y = 10 + 3.5

⇒ 5y = 13.5

⇒ y = ^13.5⁄₅

⇒ y = 2.7

Question- 16.

3x + 2 = -2.2
Answer-16:

3x + 2 = -2.2

⇒ 3x = – 2.2 – 2

⇒ 3x = – 4.2

⇒ x = -4.2/3

⇒ x = – 1.4

Question -17.

Answer-17:

^y⁄₂ – 5 = 1

⇒ ^y⁄₂ ×2-5×2=1×2

⇒ y – 10 = 2

⇒ y = 2 + 10

⇒ y = 12

Question- 18.

Answer-18:

^z⁄₃ -1=-5

⇒ ^z⁄₃×3-1×3

=-5×3

⇒ z – 3 = – 15

⇒ z = – 15 + 3

⇒ z = – 12

Question- 19.

Answer-19:

^x⁄₄+3.6=-1.1

⇒ ^x⁄₄=-1.1-3.6

⇒ ^x⁄₄–4.7

⇒ x = – 4.7 × 4

⇒ x = – 18.8

Question -20.

-3y – 2 = 10
Answer-20:

-3y – 2 = 10

⇒ – 3y = 10 + 2

⇒ – 3y = 12

⇒ y = ¹²⁄_-3

⇒ y= – 4

Question- 21.

4z – 5 = 3 – z
Answer-21:

4z – 5 = 3 – z

⇒ 4z + z = 3 + 5

⇒ 5z = 8

⇒ z = ⁸⁄₅

= 1.6

Question -22.

7x – 3x + 2 =22
Answer-22:

7x – 3x + 2 = 22

⇒ 7x – 3x = 22 – 2

⇒ 4x = 20

⇒ x = ²⁰⁄₄

⇒ x = 5

Question- 23.

6y + 3 = 2y + 11
Answer-23:

6y + 3 = 2y + 11

⇒ 6y – 2y = 11 – 3

⇒ 4y = – 8

⇒ y = ⁸⁄₄

⇒ y = 2

Question -24.

3 (x + 5) = 18
Answer-24:

3(x + 5) = 18

⇒ 3x + 15 = 18

⇒ 3x = 18 – 15

⇒ 3x = 3

⇒ x = ³⁄₃=1

⇒ x = 1

Question- 25.

5 (x – 2) – 2 (x + 2) = 3
Answer-25:

5(x – 2)- 2(x + 2) = 3

⇒ 5x – 10 – 2x – 2 = 3

⇒ 5x – 2x – 10 – 2 = 3

⇒ 3x – 12 = 3

⇒ 3x = 3 + 12

⇒ x = ¹⁵⁄₃=5

∴ x = 5

Question- 26.

(5x – 3) 4 = 3
Answer-26:

(5x – 3) 4 = 3

⇒ 20x – 12 =3

⇒ 20x = 3+12

⇒ 20x = 15

⇒ x = ¹⁵⁄₂₀

⇒ x = ³⁄₄

Question- 27.

3(2x + 1) – 2(x – 5) -5 (5 – 2x) = 16
Answer-27:

3(2x + 1) -2(x – 5) -5(5 – 2x) = 16

⇒ 6x + 3 – 2x + 10 – 25 + 10x = 16

⇒ 6x – 2x + 10x + 3 + 10 – 25 = 16

⇒ 16x – 2x + 13 – 25 = 16

⇒ 14x – 12 = 16

⇒ 14x = 16 + 12 = 28

⇒ x = ²⁸⁄₁₄=2

∴ x = 2

---

Exercise 22 D  Simple Linear Equations (Including Word Problems) ICSE Class-6th Concise Selina Mathematics 

Question 1.

A number increased by 17 becomes 54. Find the number.
Answer-1:

Let the required number = x

∴ According to the sum:

x + 17 = 54

⇒ x = 54 – 17

⇒ x = 37

Required number = 37

Question–2.

A number decreased by 8 equals 26, find the number.
Answer-2:

Let required number = A

∴ According to the sum:

x – 8 = 26

⇒ A = 26 + 8

⇒ A = 34

∴ Required number = 34

One-fourth of a number added to two- seventh of it gives 135; find the number.
Answer-3:

Let required number = x

∴ According to the sum,

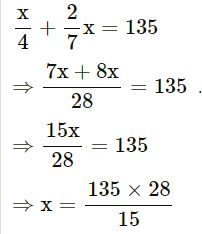
(LCM of 4, 7 = 28)

⇒ x = 9 × 28 = 252

∴ Required number = 252

Question -4.

Answer-4:

Let the required number = x

According to the sum,

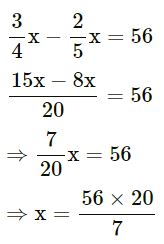
= 8×20 =160

∴ Required number = 160

Question- 5.Simple Linear Equations ICSE Class-6th Concise  

A number is increased by 12 and the new number obtained is multiplied by 5. If the resulting number is 95, find the original number.
Answer-5:

Let the original number = x

According to the sum,

(x + 12) × 5 = 95

⇒ 5x + 60 = 95

⇒ 5x = 95 – 60

⇒ 5x = 35

⇒ x = ³⁵⁄₅=7

∴ The original number = 7

Question- 6.

A number is increased by 26 and the new number obtained is divided by 3. If the resulting number is 18; find the original number.
Answer-6:

Let the original number = x

According to the sum,

(x + 26) ÷3 = 18

⇒ x+²⁶⁄₃=18

⇒ x + 26 = 18 × 3

⇒ x + 26 = 54

⇒ x = 54 – 26 = 28

Question- 7.

The age of a man is 27 years more than the age of his son. If the sum of their ages is 47 years, find the age of the son and his father.
Answer-7:

Let the age of son = x years

∴ Age of his father = x + 27

According to the sum:

x + x + 27 = 47

⇒ 2x + 27 = 47

⇒ 2x = 47 – 27 = 20

⇒ x = 202=10

∴ Age of son = 10 years

and age of his father = 10 + 27 = 37 years

Question -8.

The difference between the ages of Gopal and his father is 26 years. If the sum of their ages is 56 years, find the ages of Gopal and his father.
Answer-8:

Let age of Gopal = x years

∴ Age of his father = (x + 26) years

According to the sum,

x + x + 26 = 56

⇒ 2x + 26 = 56

⇒ 2x = 56 – 26 = 30

⇒ x = ³⁰⁄₂=15

∴ Age of Gopal = 15 years

and age of his father = 15 + 26 = 41 years

Question-9.

When two consecutive natural numbers are added, the sum is 31; find the numbers.
Answer-9:

 

Let first natural number = x

Then second natural number = x + 1

According to the sum,

x + x + 1 = 31

⇒ 2x + 1 = 31

⇒ 2x = 31 – 1 = 30

⇒ x = ³⁰⁄₂=15

∴ First natural number = 15

and second number = 15 + 1 = 16

Question- 10.

When three consecutive natural numbers are added, the sum is 66, find the numbers.
Answer-10:

Let first natural number = x

Then second natural number = x + 1

and third number = x + 2

According to the sum,

x + x + 1 + x + 2 = 66

⇒ 3x + 3 = 66

⇒ 3x = 66 – 3 = 63

⇒ x = ⁶³⁄₃=21

∴ First natural number = 21

second number = 21 + 1 = 22

and third number = 22 + 1 = 23

Hence numbers are 21, 22, 23

Question -11.

A natural number decreased by 7 is 12. Find the number.
Answer-11:

Let the required number = x

Then x – 7 = 12

⇒ x – 7 + 7 = 12 + 7  (Adding 7 to both sides)

x = 19

∴ Required number = 19

Question-12.

One fourth of a number added to one- sixth of itself is 15. Find the number.
Answer-12:

Let the required number = x

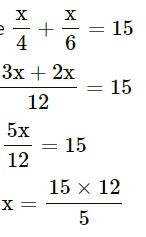
x= ¹⁸⁰⁄₅

⇒ x = 36

∴ Required number = 36

Question -13.Simple Linear Equations ICSE Class-6th Concise  

A whole number is increased by 7 and the new number so obtained is multiplied by 5; the result is 45. Find the number.
Answer-13:

Let the required whole number = x

Then (x + 7) × 5 = 45

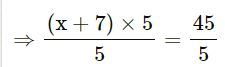
=   (Dividing by 5)

⇒ x + 7 = 9

⇒ x = 9 – 7

x = 2

∴ Required whole number = 2

Question- 14.

The age of a man and the age of his daughter differ by 23 years and the sum of their ages is 41 years. Find the age of the man.
Answer-14:

Let age of daughter = x years

Then age of man = (x + 23)

∴ x + (x + 23) = 41

x + x + 23 = 41

⇒ 2x + 23 = 41

⇒ 2x = 41 – 23 = 18

⇒ x = ¹⁸⁄₂=9

∴ Age of man = x + 23 = 9 + 23 = 32 years

Question -15.

The difference between the ages of a woman and her son is 19 years and the sum of their ages is 37 years; find the age of the son.
Answer-15:

Let age of son = x years

The age of woman = x + 19

∴ x + x + 19 = 37

⇒ 2x + 19 = 37

⇒ 2x = 37 – 19 = 18

⇒ x = ¹⁸⁄₂=9

∴ Age of son = 9 years

Question- 16. Simple Linear Equations ICSE Class-6th Concise  

Two natural numbers differ by 6 and sum of them is 36. Find the larger number.
Answer-16: 

∵ Difference between two numbers = 6

and their sum = 36

Let first natural number = x

The second number = x – 6

∴ x + x – 6 = 36

⇒ 2x = 36 + 6 = 42

x = ⁴²⁄₂=21

∴ Larger number = 21

Question -17.

The difference between two numbers is 15. Taking the smaller number as x; find:
(i) the expression for larger number.
(ii) the larger number, if the sum of these numbers is 71.
Answer-17:

Difference of two numbers = 15

Let smaller number = x

∴ Second number = x + 15

∴ Larger number = x + 15

If sum of two numbers = 71

Then x + x + 15 = 71

(i) 2x + 15 = 71

⇒ 2x = 71 – 15 = 56

x = ⁵⁶⁄₂=28

(ii) Larger number = x + 15 = 28 + 15 = 43

Question -18.

The difference between two numbers is 23. Taking the larger number as x, find:
(i) the expression for smaller number.
(ii) the smaller number, if the sum of these two numbers is 91.
Answer-18:

Difference of two numbers = 23

Let Larger number = x

(i) Then smaller number = x – 23

(ii) ∵ Sum of two numbers = 91

Then x + x – 23 = 91

⇒ 2x – 23 = 91

⇒ 2x = 91 + 23 = 114

⇒ x = ¹¹⁴⁄₂=57

∴ Smaller number = x – 23 = 57 – 23 = 34

Question- 19.

Find three consecutive integers such that their sum is 78.
Answer-19:

Sum of three consecutive numbers = 78

Let first number = x

Then second number = x + 1

and third number = x + 2

Then x + x+1+x + 2 = 78

⇒ 3x + 3 = 78

⇒ 3x = 78 – 3 = 75

⇒ x = ⁷⁵⁄₃=25

∴ First number=25

Second number = 25 + 1 = 26

and third number = 26 + 1 = 27

Then the three required numbers are 25, 26, 27

Question -20.

The sum of three consecutive numbers is 54. Taking the middle number as x, find:
(i) expression for the smallest number and the largest number.
(ii) the three numbers.
Answer-20:

Sum of three consecutive numbers = 54

Middle number = x

(i) The first number = x – 1

and third number = x + 1

(ii) ∴x + x-1+x+1 = 54

⇒ 3x = 54

⇒ x = ⁵⁴⁄₃=18

∴ First number =18 – 1 = 17

and third number =18 + 1 = 19

∴ Three required numbers are 17, 18,19

---

Revision Exercise Simple Linear Equations (Including Word Problems) for ICSE Class-6th Concise Selina Mathematics Solutions

Question- 1. Solve each of the following equations :

Question -i.

2x + 3 = 7
Answer-i:

2x + 3 = 7

⇒ 2x + 3 – 3 = 7 – 3  …(Subtracting 3 from both sides)

⇒ 2x = 4

⇒ ^2x⁄₂=⁴⁄₂ ….(Dividing by 2)

⇒ x = 2

∴ x = 2

Question -ii

2x – 3 = 7

Answer-ii:

2x – 3 = 7

⇒ 2x – 3 + 3 = 7 + 3  …(Adding 3 to both sides)

⇒ 2x = 10

⇒ ^2x⁄₂=^2x⁄₂ ….(Dividing by 2)

⇒ x = 2

∴ x = 5

Question iii.

2x ÷ 3 = 7
Answer-iii:

2x ÷ 3 = 7

⇒ ^2x⁄₃=7

⇒ ^2x⁄₃×3=7×3 …(Multiplying by 3)

⇒ 2x = 21

⇒ ^2x⁄₂=²¹⁄₂ ….(Dividing by 2)

⇒ x = ²¹⁄₂

= ..10.¹⁄₂………………..

Question- iv.

3x – 8 = 13
Answer-iv:

3y – 8 = 13

⇒ 3y – 8 + 8 = 13 + 8   …(Adding 8 to both sides)

⇒ 3y = 21

⇒ ^3y⁄₃=²¹⁄₃  ….(Dividing by 3)

∴ y = 7

Question -v.

3y + 8 = 13

Answer-v:

3y + 8 = 13

⇒ 3y + 8 – 8 = 13 – 8   …(Substracting 8 from both sides)

⇒ 3y = 5

⇒ ^3y⁄₃=⁵⁄₃  ….(Dividing by 3)

∴ y = ⁵⁄₃

⇒  y= .1.²⁄₃………

Question- vi.

3y ÷ 8 = 13
Answer-vi:

3y ÷ 8 = 13

⇒ ^3y⁄₈=13

⇒ ^3y⁄₈×8=13×8   …(Multiplying by 8)

⇒ 3y = 104  ….(Dividing by 3)

∴ y =  .¹⁰⁴⁄₃…= 34 ²⁄₃………………………………………..

Question -vii.

x – 3 = .5 ¹⁄₂..
Answer-vii:

x – 3 = .5 ¹⁄₂..

⇒ x – 3 + 3 = 5 ¹⁄₂.+3  ..(Adding 3 to both sides)

∴ x = 8 ¹⁄₂.

Question -viii.

^3x⁄₅.+4=13
Answer-viii:

^3x⁄₅.+4=13

⇒ ^3x⁄₅+4-4=13-4  ..(subtracting 4 from both sides)

⇒  ^3x⁄₅=9

⇒ ^3x⁄₅×⁵⁄₃=9× ⁵⁄₃  …(Multiplying by ⁵⁄₃)

∴ x = 15

Question- ix.

u + 3 ¹⁄₄.=4 ¹⁄₃.
Answer-9:

u + 3 ¹⁄₄.=4 ¹⁄₃.

⇒u+¹³⁄₄=¹³⁄₃

⇒u+¹³⁄₄–¹³⁄₄=¹³⁄₃–¹³⁄₄  …(Substracting ¹³⁄₄ from both sides)

⇒u=^52-39⁄₁₂= ¹³⁄₁₂

.⇒….1 ¹⁄₁₂

Question -x.

5x – 2.4 = 4.9
Answer-x:

5x – 2.4 = 4.9

⇒ 5x – 2.4 + 2.4 = 4.9 + 2.4   …(Adding 2.4 to both sides)

⇒ 5x = 7.3

⇒ ^5x⁄₅=^7.3⁄₅  ..(Dividing by 5)

∴ x = 1.46

Question- xi.

5y + 4.9 = 2.4
Answer-xi:

5y + 4.9 = 2.4

⇒ 5x + 4.9 – 4.9 = 2.4 – 4.9   …(Substracting 4.9 from both sides)

⇒ 5y = – 2.5

⇒ ^5y⁄₅ = –^2.5⁄₅  ..(Dividing by 5)

∴ x = – 0.5

Question- xii.

48 z + 3.6 = 1.2
Answer-xii:

48 z + 3.6 = 1.2

⇒ 4.8z + 3.6 – 3.6 = 1.2 – 3.6   …(Substracting 3.6 from both sides)

⇒ 4.8z = – 2.4

⇒ ^4.8z⁄_4.8=-^2.4⁄_4.8  ..(Dividing by 4.8)

∴ z = ^-1⁄₂

= – 0.5

Question -xiii.Simple Linear Equations ICSE Class-6th Concise  

^x⁄₂ – 3 = 5
Answer-xiii

^x⁄₂ – 3 = 5

⇒ ^x⁄₂-3+3=5+3   …(Adding 3 to both sides)

⇒ ^x⁄₂=8

⇒ ^x⁄₂×2=8×2   ..(Multiplying by 2)

∴ x = 16

Question- xiv.

Answer-xiv:

^y⁄₃+7=2

⇒ ^y⁄₃+7-7=2-7   …(Substracting 7 from both sides)

⇒ ^y⁄₃=-5

⇒ ^y⁄₃×3=-5×3   ..(Multiplying by 3)

∴ y = – 15

Question -xv.

Answer-xv:

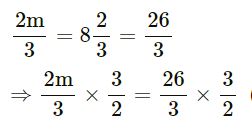
(Multiplying both side by ³⁄₂)

m= 13

Question- xvi.

-3x + 4 = 10
Answer-xvi:

– 3x + 4 = 10

⇒ – 3x + 4 – 4 = 10 – 4   (Substracting 4 from both sides)

⇒ – 3x = 6

⇒ – ^3x⁄_-3 =⁶⁄_-3 (Dividing by – 3)

∴ x = -2

Question -xvii.

5 = x – 3
Answer-xvii:

5 = x – 3

⇒ 5 + 3 = x – 3 + 3  (Adding 3 to both sides)

⇒ 8 = x

∴ x = 8

Question -xviii.

8y = 3- 3y
Answer:-xviii.

8y = 3- 3y

⇒ 18 – 3 = 3 – 3y – 3  (Substrating 3 from both sides)

⇒ 15 = – 3y

⇒ ¹⁵⁄_-3=-^3y⁄_-3   (Dividing by -3)

⇒ – 5 = y

∴ y = – 5

Question- xix.

4x = 4.9 = 6.5
Answer-xix:

4x + 4.9 = 6.5

⇒ 4x + 4.9 – 4.9 = 6.5 – 4.9

(Substracting 4.9 from both sides)

⇒ 4x = 1.6

⇒ ^4x⁄₄=^1.6⁄₄   (Dividing by 4)

⇒ x = 0.4

∴ x = 0.4

Question- xx.

3z + 2 = -4
Answer-xx:

3z + 2 = -4

⇒ 3z + 2 – 2 = – 4 – 2 (Substrating -2 from both sides)

⇒ 3z = – 6

⇒ ^3z⁄₃=-⁶⁄₃   (Dividing by 3)

∴ z = – 2

Question -xxi.

7y – 18 = 17
Answer-xxi:

7y -18 = 17

⇒ 7y – 18 + 18 = 17 + 18 (Adding 18 to both sides)

⇒ 7y = 35

(Dividing by 7)

∴ y = 5

Question- xxii.

^x⁄_1.2 -6=1
Answer-xxii:

^x⁄_1.2 -6=1

⇒ ^x⁄_1.2-6+6=1+6 (Adding 6 to both sides)

⇒ ^x⁄_1.2  = 7

⇒ ^x⁄_1.2=7×1.2    (Multiplying by 1.2)

∴ x = 8.4

Question- xxiii.

^z⁄_2.4+3.6=5.1

Answer-xxiii:

^z⁄_2.4+3.6=5.1

⇒^z⁄_2.4+3.6-3.6=5.1-3.6 (Substracting 3.6 from both sides)

⇒ ^z⁄_2.4  = 1.5

⇒ ^z⁄_2.4×2.4=1.5×2.4    (Multiplying by 2.4)

⇒ z = 3.60

∴ z = 3.6

Question- xxiv.

^y⁄_1.8-2.1=-2.8
Answer-xxiv:

^y⁄_1.8-2.1=-2.8

⇒ ^y⁄_1.8-2.1+2.1=-2.8+2.1 (Adding 2.1 to both sides)

⇒ ^y⁄_1.8  = – 0.7

⇒ ^y⁄_1.8×1.8=-0.7×1.8    (Multiplying by 1.8)

∴ y = – 1.26

Question- xxv.

7x – 2 = 4x + 7
Answer-xxv:

7x – 2 = 4x + 7

⇒ 7x – 2 + 2 = 4x + 7 + 2  ..(Adding 2 to both sides)

⇒ 7x = 4x + 9

⇒ 7x – 4x = 4x + 9 – 4x   …(Substracting 4x from both sides)

⇒ 3x = 9

(Dividing by 3)

∴ x = 3

Question- xxvi.

3y -(y + 2) = 4
Answer-xxvii:

3z – 18 = z – (12 – 4z)

⇒ 3z – 18 = z – 12 + 4z

⇒ 3z – 18 = 5z – 12

⇒ 3z – 18 + 18 = 5z – 12 + 18   …(Adding 18 to both sides)

⇒ 3z = 5z + 6

⇒ 3z – 5z = 5z + 6 – 5z  (Subtracting 5z from both sides)

⇒ – 2z = 6

∴ z = – 3

Question- xxvii.

3z – 18 = z – (12 – 4z)
Answer-xxvii.

3z – 18 = z – (12 – 4z)

⇒ 3z – 18 = z – 12 + 4z

⇒ 3z – 18 = 5z – 12

⇒ 3z – 18 + 18 = 5z – 12 + 18   …(Adding 18 to both sides)

⇒ 3z = 5z + 6

⇒ 3z – 5z = 5z + 6 – 5z  (Subtracting 5z from both sides)

⇒ – 2z = 6

…(Dividing by -2)

∴ z = – 3

Question -xxviii.

Answer-xxviii:

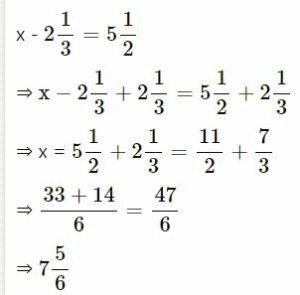
Question- xxix.

Answer- xxix.

Question- xxx.

Answer-xxx:

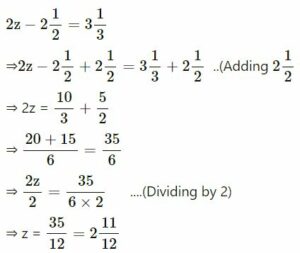
Question- xxxi.

5x – 2x + 15 = 27
Answer-xxxi:

5x – 2x +15 = 27

⇒ 3x + 15 = 27

⇒ 3x + 15 – 15 = 27 – 15  …(Subtracting 15 from both sides)

⇒  3x = 12

..(Dividing by 3)

⇒ x = 4

Question- xxxii.

5y – 15 = 27 -2y
Answer-xxxii:

5y – 15 = 27 -2y

⇒ 5y + 2y – 15 = 27 – 2y + 2y (Adding 2y to both sides)

⇒  7y – 15 = 27

⇒ 7y – 15 + 15 = 27 + 15  …(Adding 15 to both sides)

⇒ 7y = 42

…(Dividing by 7)

⇒ y = 6

Question- xxxiii.

7z + 15 = 3z – 13
Answer-xxxiii:

7z + 15 = 3z – 13

⇒ 7z + 15 – 3z = 3z – 13 – 3z (Subtracting 3z from both sides)

⇒ 4z + 15 = – 13

⇒ 4z + 15 – 15 = – 13 – 15  …(Subtracting 15 from both sides)

⇒ 4z = – 28

…(Dividing by 4)

⇒ z = – 7

Question -xxxiv.

2 (x -3) – 3 (x-4) =12
Answer-xxxiv:

2 (x – 3) – 3 (x – 4) =12

⇒ 2x – 6 – 3x + 12 = 12

⇒ – x + 6 = 12

⇒ – x + 6 – 6 = 12 – 6  …(Subtracting 6 from both sides)

⇒ – x = 6

⇒ x = – 6

Question- xxxv.

(7y + 8) + 7 = 8
Answer-xxxv:

^7y+8⁄₇= 8

⇒ ^7y+8⁄₇=8

⇒^7y+8⁄₇×7=8×7  …(Multiplying by 7)

⇒ 7y + 8 = 56

⇒ 7y + 8 – 8 – 56 – 8  …(Subtracting 8 from both sides)

⇒ 7y = 48

⇒ ^7y+8⁄₇=⁴⁸⁄₇  ..(Dividing by 7)

⇒ y = ⁴⁸⁄₇

⇒ y =…6.⁶⁄₇………….

Question -xxxvi.

2(z – 5) +3 (z + 2) -(3 – 5z) = 10
Answer-xxxvi:

2(z – 5) +3 (z + 2) – (3 – 5z) =10

⇒ 2z – 10 + 3z + 6 – 3 + 5z = 10

⇒ 10z – 7 = 10

⇒ 10z – 7 + 7 = 10 + 7    …(Adding 7 to both sides)

⇒  10z = 17

..(Dividing by 10)

⇒ z = .¹⁷⁄₁₀

⇒ z = .1⁷⁄₁₀

---

Simple Linear Equations ICSE Class-6th Concise  

Question -2.

A natural number decreased by 7 is 12. Find the number.
Answer-2:

Let the required number = x

Then x – 7 = 12

⇒ x – 7 + 7 = 12 + 7  (Adding 7 to both sides)

x = 19

∴ Required number = 19

Question- 3.

One-fourth of a number added to one-sixth of It is 15. Find the number.
Answer-3:

Let the required number = x

⇒ x = 36

∴ Required number = 36

Question- 4.

A whole number is increased by 7 and the number so obtained is multiplied by 5; the result is 45. Find the whole number.
Answer-4:

Let the required whole number = x

Then (x + 7) × 5 = 45

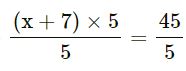
(Dividing by 5)

⇒ x + 7 = 9

⇒ x = 9 – 7

x = 2

∴ Required whole number = 2

Question -5.

The age of a man and the age of his daughter differ by 23 years and the sum of their ages is 41 years. Find the age of the man.
Answer-5:

Let age of daughter = x years

Then age of man = (x + 23)

∴ x + (x + 23) = 41

x + x + 23 = 41

⇒ 2x + 23 = 41

⇒ 2x = 41 – 23 = 18

⇒ x = 18/2 =9

∴ Age of man = x + 23 = 9 + 23 = 32 years

Question -6.

The difference between the ages of a woman and her son is 19 years and the sum of their ages is 37 years; find the age of the son.
Answer-6:

Let age of son = x years

The age of woman = x + 19

∴ x + x + 19 = 37

⇒ 2x + 19 = 37

⇒ 2x = 37 – 19 = 18

⇒ x = 18/2=9

∴ Age of son = 9 years

Question- 7.

Two natural numbers differ by 6 and their sum is 36. Find the larger number.
Answer-7:

∵ Difference between two numbers = 6

and their sum = 36

Let first natural number = x

The second number = x – 6

∴ x + x – 6 = 36

⇒ 2x = 36 + 6 = 42

x = 42/2=21

∴ Larger number = 21

Question -8.

The difference between two numbers is 15. Taking the smaller number as x; find :
(i) the expression for the larger number.
(ii) the larger number, if the sum of these numbers is 71.
Answer-8:

Difference of two numbers = 15

Let smaller number = x

∴ Second number = x + 15

∴ Larger number = x + 15

If sum of two numbers = 71

Then x + x + 15 = 71

(i) 2x + 15 = 71

⇒ 2x = 71 – 15 = 56

x = 56/2=28

(ii) Larger number = x + 15 = 28 + 15 = 43

Question -9.

The difference between two numbers is 23. Taking the larger number as x, find :
(i) the expression for smaller number.
(ii) the smaller number, if the sum of these two numbers is 91.
Answer-9:

Difference of two numbers = 23

Let Larger number = x

(i) Then smaller number = x – 23

(ii) ∵ Sum of two numbers = 91

Then x + x – 23 = 91

⇒ 2x – 23 = 91

⇒ 2x = 91 + 23 = 114

⇒ x = 114/2=57

∴ Smaller number = x – 23 = 57 – 23 = 34

Question- 10.

Find the three consecutive integers whose sum is 78.
Answer-10:

Sum of three consecutive numbers = 78

Let first number = x

Then second number = x + 1

and third number = x + 2

Then x + x+1+x + 2 = 78

⇒ 3x + 3 = 78

⇒ 3x = 78 – 3 = 75

⇒ x = 75/3=25

∴ First number=25

Second number = 25 + 1 = 26

and third number = 26 + 1 = 27

Then the three required numbers are 25, 26, 27

Question -11.

The sum of three consecutive numbers is 54. Taking the middle number as x, find :
(i) the expressions for the smallest number and the largest number.
(ii) the three numbers.
Answer-11

Sum of three consecutive numbers = 54

Middle number = x

(i) The first number = x – 1

and third number = x + 1

(ii) ∴x + x-1+x+1 = 54

⇒ 3x = 54

⇒ x = 54/3=18

∴ First number =18 – 1 = 17

and third number =18 + 1 = 19

∴ Three required numbers are 17, 18,19

 End of Simple Linear Equations ICSE Class-6th Solutions :–

Return to – Concise Selina Maths Solutions for ICSE Class -6

---

Thanks