Triangles Class-6 RS Aggarwal ICSE Maths Goyal Brothers Prakashan Chapter-16. We provide step by step Solutions of Exercise / lesson-16 Triangles for ICSE Class-6 RS Aggarwal Mathematics.
Our Solutions contain all type Questions with Exe-16 A and Exe-16 B, to develop skill and confidence. Visit official Website CISCE for detail information about ICSE Board Class-6 Mathematics.
| Board | ICSE |
| Publications | Goyal brothers Prakshan |
| Subject | Maths |
| Class | 6th |
| Chapter-16 | Triangles |
| Writer | RS Aggrawal |
| Book Name | Foundation |
| Topics | Solution of Exe-16 A and Exe-16 B |
| Academic Session | 2021-2022 |
Triangles Class-6 RS Aggarwal ICSE Maths Goyal Brothers Prakashan Chapter-16
–: Select Topics :–
a triangle is a type of polygon, which has three sides, and the two sides are joined end to end is called the vertex of the triangle.
Angles of Triangle
There are three angles in a triangle. These angles are formed by two sides of the triangle, which meets at a common point, known as the vertex. The sum of all three interior angles is equal to 180 degrees.
Properties of Triangle
Each and every shape in Maths has some properties which distinguish them from each other. Let us discuss here some of the properties of triangles.
- A triangle has three sides and three angles.
- The sum of the angles of a triangle is always 180 degrees.
- The exterior angles of a triangle always add up to 360 degrees.
- The sum of consecutive interior and exterior angle is supplementary.
- The sum of the lengths of any two sides of a triangle is greater than the length of the third side. Similarly, the difference between the lengths of any two sides of a triangle is less than the length of the third side.
- The shortest side is always opposite the smallest interior angle. Similarly, the longest side is always opposite the largest interior angle
Types of Triangles
On the basis of length of the sides, triangles are classified into three categories:
- Scalene Triangle :- A scalene triangle is a type of triangle, in which all the three sides have different side measures. Due to this, the three angles are also different from each other
- Isosceles Triangle :- In an isosceles triangle, two sides have equal length. The two angles opposite to the two equal sides are also equal to each other
- Equilateral Triangle :– An equilateral triangle has all three sides equal to each other. Due to this all the internal angles are of equal degrees, ie. each of the angles is 60°
On the basis of measurement of the angles, triangles are classified into three categories:
- Acute Angle Triangle :- An acute triangle has all of its angles less than 90°
- Right Angle Triangle :-In a right triangle, one of the angles is equal to 90° or right angle.
- Obtuse Angle Triangle :- An obtuse triangle has any of its one angles more than 90°.
Exe-16 A,
Triangles Class-6 RS Aggarwal ICSE Maths Goyal Brothers Prakashan Chapter-16
Page 211-212
Question 1:
Two angles of a triangle are 72° and 63°. Find the third angle.
Answer :
Two angles of a triangle are 72° and 63° But sum of three angles of a triangle = 180°
So, Third angle 180° (sum of two angles)
= 180° – (72°+ 63°) 180° – 135°
= 45°
Question 2:
The angles of a triangle are in the ratio 2:3:5. Find the measure of each angle of the triangle.
Answer :
The angles of a triangle be 2x, 3x and 5x
2x + 3x + 5x = 180°
=> 10x = 180°
=> x = (180°/10°)
= 18°
So, Angles of a triangle
2x = 2 x 18° = 36°
3x = 3 x 18° = 54°
5x = 5 x 18° = 90°
Question 3:
The acute angles of a right triangle are in the ratio 1:2. Find the angles of the triangle.
Answer :
The acute angles are x and 2x
x + 2x = 90°
=> 3x = 90°
=> x = 90°/3
= 30°
Acute angles x = 30°
2x = 2 x 30° = 60° and 3rd angle = 90°
Question 4:
One of the acute angles of a right triangle is 36°, Find the other acute angle.
Answer :
In right angled triangle, one of acute angles = 36°
So, Second acute angle 90° – 36° = 54°
Question 5:
One of the angles of a triangle is 106° and the other two angles are equal. Find each of the equal angles.
Answer :
Each equal angle be ‘x’
x + x + 106° = 180°
2x + 106° = 180°
2x = 180°- 106°
2x = 74°
=> x = 74°/2
= 37°
The each of equal angle be 37°.
Question 6:
Each of the two equal angles of an isosceles triangle is half the third angle. Find the angles of the triangle.
Answer :
Let the two equal angles are x/2 and x/2 and the third angle be ‘x’.
x +(x/2) + (x/2) = 180°
(2x + x + x)/2 = 180°
(4x/2) = 180°
2x = 180°
x = (180/2) = 90°
Third angle = 90°
The other angles are (x/2). (x/2) = (90°/2)
ex. 45° and 45°
Question 7:
In the given figure, find ∠A + ∠B + ∠C + ∠D+ ∠E + ∠F
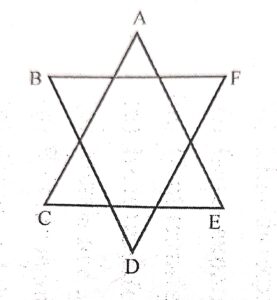
Answer :
In △ACE
∠A + ∠C + ∠E 180° …(i)
In △BDF
∠B + ∠D + ∠F= 180° ……….(ii)
Adding (i) and (ii), we get
∠A + ∠C+ ∠E + ∠B + ∠D + ∠F
180° + 180°= 360°
∠A + ∠B + ∠C + ∠D + ∠E + ∠F = 360°
Question 8:
In each of the figures given below state whether the given triangle is acute-angled, right-angled or obtuse-angled.
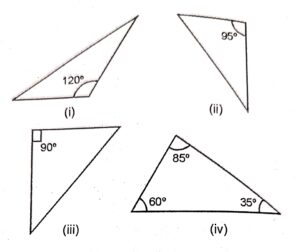
Answer :
(i) Obtuse-angled as one angle is 120°.
(ii) Obtuse-angled as one angle is 95°.
(iii) Right-angled as one angle is 90°
(iv) Acute angled as all angles are less than 90°
Question 9:
Can a triangle have:
(i) each angle less than 60°?
(ii) each angle greater than 60?
(iii) tw0 obtuse angles ?
(iv) two right angles ?
(v) two acute angles ?
Answer :
(i) Each angle less than 60°
No, it is also not possible as the sum of three angles of a triangle cannot be less than 180.
(ii) Each angle is greater than 60°
No, it is not possible, as in this triangle sum of angles is greater than 180°.
(iii) Two obtuse angles.
No, it is possible as a triangle has at the most one obtuse angle.
(iv) Two right angles.
No, it is not possible as a triangle has at the most one right angle.
(v) Two acute angles.
Yes, it is possible.
Question 10:
Which of the following can possibly be the angles of a triangle ?
(i) 50°, 30°, 100°
(ii) 90° 50°, 50°
(iii) 20°, 70°, 90°
(iv) 45, 90°, 45°
(v) 65, 65, 65°
(vi) 120°, 50°, 10°
Answer :
(i) Yes, Sum of angles = 50° + 30°+ 100°
= 180°
It is a triangle.
(ii) Sum of angles = 90° + 50° + 50°
= 190° ≠ 180°.
It is not a triangle.
(iii) Yes, Sum of angles = 20° + 70° + 90°
= 180°
It is a triangle.
(iv) Yes, Sum of angles = 45° +90° +45°
= 180°
It is a triangle.
(v) Sum of angles = 65° +65° + 65 195
195° ≠ 180°.
It is not a triangle
(vi) Sum of angles = 120° + 50° + 10°
= 180°
It is a triangle.
So, These angles of possible of a triangle.
Question 11:
In each of the figures given below state whether the given triangle is equilateral, isosceles or scalene :
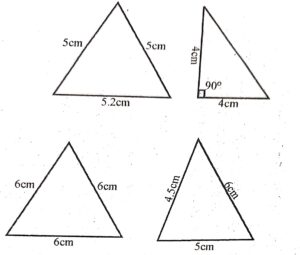
Answer :
(i) Isosceles
(ii) Isosceles
(iii) Equilateral
(iv) Scalene.
Question 12:
Which of the following lengths of three line segments can be the lengths of the sides of a triangle ?
(i) 5 cm, 4 cm, 9 cm
(ii) 8 cm, 6 cm, 15 cm
(iii) 5 cm, 5 cm, 5 cm
(iv) 3.5 cm, 4.5 cm, 6.5 cm
(v) 6 cm, 8 cm, 10 cm
(vi) 3 cm, 7 cm, 3 cm
Answer :
Sum of any two sides of a triangle is greater than its third side therefore
(i) 5 cm, 4 cm, 9 cm
5 cm + 4 cm = 9 cm
9 cm = 9 cm,
The triangle is possible.
(ii) 8 cm, 6 cm, 15 cm
8 cm + 6 cm = 14 cm
So, 14 cm 15 cm which is not possible to draw a triangle.
(iii) 5 cm, 5 cm, 5 cm
As three sides are equal
The triangle is possible.
(iv) 3.5 cm, 4.5 cm, 6.5 cm
3.5 cm + 4.5 cm 8 cm
and 8 cm> 6.5 cm
The triangle is possible.
(v) 6 cm, 8 cm, 10 cm
6 cm + 8 cm = 14 cm and 14 cm > 10 cm
The triangle is possible.
(vi) 3 cm, 7 cm, 3 cm
Sum of the small sides 3 + 3
=6 cm > 7 cm
The triangle is not possible.
Question 13:
(i) Can a right triangle be equilateral ?
(ii) Can an isosceles triangle be right-angled ?
(iii) Can a right triangle be scalene ?
(iv) Can aright triangle have an obtuse angle ?
Answer :
(i) No
(ii) No
(iii) Yes
(iv) No
Question 13:
Fill in the blanks :
(i) A triangle has …………… Vertices,. …….sides and angles.
(ii) The sum of the angles of a triangle is …….
(iii) Each angle of an equilateral triangle measures. ……
(iv) The sum of any two sides of a triangle is………..than the third side.
(v) Aright triangle cannot have an ………… angle.
Answer :
(i) A triangle has 3 Vertices, 3 sides and 3 angles.
(ii) The sum of the angles of a triangle is 180°.
(iii) Each angle of an equilateral triangle measures 60°.
(iv) The sum of any two sides of a triangle is greater than the third side.
(v) Aright triangle cannot have an Obtuse angle.
Exe-16 B,
Triangles Class-6 RS Aggarwal ICSE Maths Goyal Brothers Prakashan Chapter-16
Page 214
Question 1:
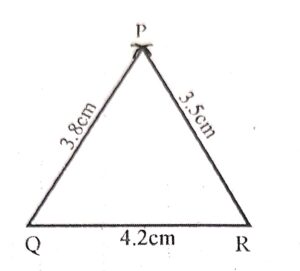
Construct a △PQR in which PQ = 3.8 cm, QR = 4.2 cm and PR = 3.5 cm.
Answer :
Construction :
(i) Draw a line segment QR = 4.2 cm
(ii) With centers Q and R radii 3.8 cm and 3.5cm respectively, draw arcs which intersect each other at P.
(iii) Join PQ and PR
△PQR is the required triangle.
Question 2:
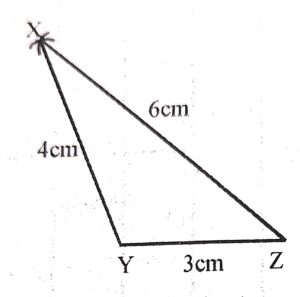
Construct a △XYZ in which XY = 4 cm YZ 3 cm and XZ = 6 cm. Draw its medians.
Answer :
Construction:
(i) Draw a line segment YZ = 3 cm.
(ii) With centers Y and Z and radii 4cm and 6cm respectively draw arcs which intersect each other at X.
(iii) Join XY and XZ
△XYZ is the required triangle.
Question 3:
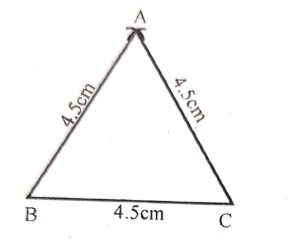
Construct a AABC in which AB = BC = CA = 4.5cm. Draw its medians Measure ∠A, ∠B, ∠C, What do you observe?
Answer :
Construction:
(i) Draw a line segment BC = 4.5 cm
(ii) With centers B and C, and radius 4.5 cm, each draw arcs which intersect each other at A.
(iii) Join AB at AC.
△ABC is the required equilateral triangle.
Question 4:
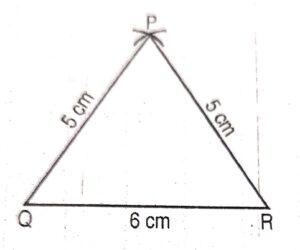
Construct a △PQR in which PQ= PR = 5 cm and QR= 6 cm. Measure ∠Q and ∠R. What do you observe ?
Answer :
Construction:
(i) Draw a line segment QR = 6 cm.
(ii) With Q as centre and radius 5 cm draw an arc.
(iii) With R as centre and radius= 5 cm draw another arc cutting the previous arc at P.
(iv) Join PQ and PR.
△PQR is required triangle. By measurement we observe that ∠Q = ∠R and it is a isosceles triangle.
Question 5:
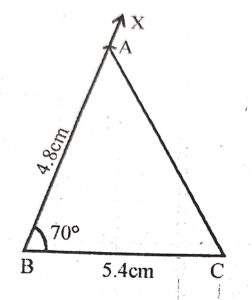
Construct a △ABC in which AB = 4.8 cm, BC = 5.4 cm and ∠B = 70°
Answer :
Construction :
(i) Draw line segment BC = 5.4 cm
(ii) At B, draw a ray BX making an angle of 70° and cut off BA = 4.8 cm.
(iii) Join AC.
△ABC Is the required triangle.
Question 6:
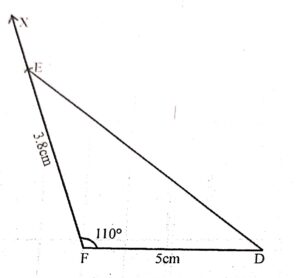
Construct a △DEF in which EF = 3.8 cm, ∠F = 110° and FD = 5 cm.
Answer :
Construction :
(i)Draw a line segment FD= 5 cm.
(ii) At F, draw a ray FX making an angle of 110° and cut off FE = 3.8 cm.
(ii) Join ED
So, EFD is required triangle.
Question 7:
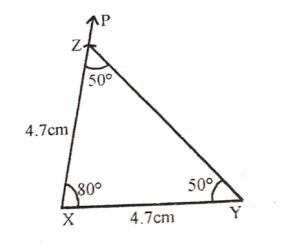
Construct a △XYZ in which XY = XZ = 4.7 cm and ∠X = 80°. Measure ∠Y and ∠Z.
Answer :
Construction :
(i) Draw a line segment XY = 4.7 cm.
(ii) At X draw a ray XP making an angle of 80° and cut off XZ = 4.7 cm.
(iii) Join ZY.
△XYZ is the required triangle.
∠Y = ∠Z = 50°
Question 8:
Construct a △ABC in which BC = 5 cm, ∠B 70° and ∠C 50°.
Answer :
Construction :
(i) Draw line segment BC-5 cm
(ii) At B, draw a ray BX making an angle of 70° an at C, draw another ray making an angle of 50° which intersects BX at A.
△ABC is the required triangle.

Question 9:
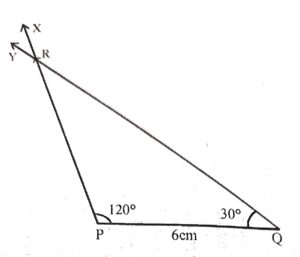
Construct a △PQR in which PQ = 6 cm, P = 120° and ∠Q 30°. Measure ∠R.
Answer :
Construction :
(i) Draw a line segment PQ = 6 cm
(ii) At P, draw a ray PX making an angle of 120° and at Q, draw another ray making an angle of 30°
Which intersects PX at R.
△PQR is the required triangle.
Question 10:
Construct a △XYZ in which XY = 5.3 cm, ∠Y = 60° and ∠Z = 75°
Answer :
Construction:
(i) Draw a line segment XY = 5.3 cm
(ii). Construct ∠YXP = 45°
(iii) Construct ∠XYO = 60°
Let the rays XP and YQ intersect at Z.
△XYZ is the required triangle.
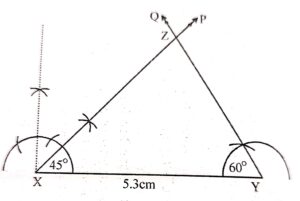
=> In △XYZ
∠X + ∠Y + ∠Z = 180°
∠X + 60°+ 75° = 180°
∠X + 135° = 180°
∠X = 180° – 135°
∠X = 45°
–: End of Triangles Class-6 RS Aggarwal Solutions :–
Return to- RS Aggarwal Solutions for ICSE Class-6 Goyal Brothers Prakashan
Thanks


