Arithmetic Progression Concise Solutions for ICSE Maths Chapter-10. Solutions of Arithmetic Progression with Exercise – 10 (A), Exercise – 10 (B), Exercise – 10 (C), Exercise – 10 (D), Exercise –10 (E) and Exercise – 10 (F) for Concise Selina Maths for ICSE Board Class 10th. Concise Solutions Matrices Chapter – 10 for ICSE Maths Class 10 is available here. All Solutions of Concise Selina of Chapter 10 Arithmetic Progression has been solved according instruction given by council. This is the Solutions of Chapter-10 Arithmetic Progression for ICSE Class 10th. ICSE Maths text book of Concise is In series of famous ICSE writer in maths. Concise is most famous among students.
Arithmetic Progression Concise Solutions for ICSE Maths Chapter-10
The Solutions of Concise Mathematics Chapter 10 ,Arithmetic Progression for ICSE Class 10th have been solved. Experience teachers Solved Chapter-10 Arithmetic Progression to help students of class 10th ICSE board. Therefore the ICSE Class 10th Maths Solutions of Concise Selina Publishers Maths helpful on various topics which are prescribed in most ICSE Maths textbooks
–: Select Toipc:–
Exercise-10(A), Arithmetic Progression Concise Solutions
Question 1
Which of the following sequences are in arithmetic progression?
(i) 2, 6, 10, 14, …….
(ii) 15, 12, 9, 6,…….
(iii) 5, 9, 12, 18, ……
(iv) ,…..
Answer 1
(i) 2, 6, 10, 14,
Here, d = 6 – 2 = 4
10 – 6 = 4
14 – 10 = 4
∴ In each case (d) is same.
∴It is an arithmetic progression.

Question 2
The nth term of a sequence is (2n – 3), find its fifteenth term.
Answer 2
Tn = 2n – 3
T15 = 2 x 15 – 3
= 30 – 3
= 27
Question 3
If the pth term of an A.P. is (2p + 3); find the A.P.
Answer 3
Tp = 2p + 3
and T1 = 2 x 1 + 3 = 2 + 3 = 5
so T2 = 2 x 2 + 3 = 4 + 3 = 7
then T3 = 2 x 3 + 3 = 6 + 3 = 9
∴A.P. is 5, 7, 9,…..
Question 4.
Find the 24th term of the sequence : 12, 10, 8, 6,…
Answer 4
A.P. is 12, 10, 8, 6,……
Here a = 12, d = 10 – 12 = – 2
Tn = a + (n – 1)d
T24 = 12 + (24 – 1) x ( – 2)
= 12 + 23 x ( – 2)
and = 12 – 46
hence = – 34
Question 5.
Find the 30th term of the sequence :
, 1,
,….
Answer 5

Question 6
Find the 100th term of the sequence :
√3, 2√3, 3√3,….
Answer 6
3, 2√3, 3√3,….
Here a = √3, d = 2√3 – √3 = √3
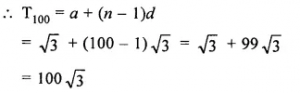
Question 7
Find the 50th term of the sequence :
,
,
,……
Answer 7
,
,
,……
=>,
,
,…..

Question 8
Is 402 a term of the sequence :
8, 13, 18, 23,…?
Solution: 8
In sequence 8, 13, 18, 23,…..
a = 8 and d = 13 – 8 = 5
Let 402 be the nth term, then
402 = a + (n – 1)d = 8 + (n – 1) x 5
= n – 1
=> = n – 1
394 is not exactly divisible by 5,
∴ 402 is not its term.
Question 9.
Find the common difference and 99th term of the arithmetic progression :
,
,
,…..
Solution 9
,
,
,…..
here a =
=

Question 10.
How many terms are there in the series:
(i) 4, 7, 10, 13,…..148 ?
(ii) 0.5, 0.53, 0.56,…..1.1 ?
(iii) , 1,
,….3 ?
Answer 10
(i) 4, 7, 10, 13,…..148 ?
Here a = 4, d = 7 – 4
= 3
Let 148 be the nth term, then


Question 11.
Which term of the A.P. 1 + 4 + 7 + 10 +……is 52 ?
Answer 11
A.P. is 1, 4, 7, 10,……is 52
Here a = 1, d = 4 – 1 = 3
Let 52 be the nth term, then

Question 12.
If 5th and 6th terms of an A.P. are respectively 6 and 5, find the 11th term of the A.P.
Answer 12
5th term (T5) = 6
=> 6 = a + 4d
and T6 = 5
=> 5 = a + 5d

Question 13.
If tn represents nth term of an A.P., t2 + t5 – t3 = 10 and t2 + t9 = 17, find its first term and its common difference.
Answer 13
Let first term of an A.P. be a
and common difference = d
Then Tn is the nth term

Question 14.
Find the 10th term from the end of the A.P. 4, 9, 14,…. 254.
answer 14
We know that rth term from the end
= (n – r + 1)th from the beginning
Here, a = A, d = 9 – 4 = 5,
nth term = 254

Question 15.
Determine the arithmetic progression whose 3rd term is 5 and 7th term is 9.
Answer 15
Let a be the first term and d be the common difference, then
T3 = a + 2d – 5
T7 = a + 6d = 9

Question 16.
Find the 31st term of an A.P. whose 10th term is 38 and 16th term is 74.
Answer 16
Let a be the first term and d be the common difference, then
T10 = a + 9d =38
and T16 = a + 15d = 74

Question 17.
Which term of the series :
21, 18, 15, is – 81 ?
Can any term of this series be zero ? If yes, find the number of term.
Answer 17
21, 18, 15, 1….. – 81
Here a = 21,d = 18 – 21 = – 3,
nth term = – 81

Question 18.
An A.P. consists of 60 terms. If the first and the last terms be 7 and 125 respectively, find the 31st term.
Answer 18
Given : An A.P. consists of 60 terms
a = 7
and a60 = 125
=> a + (60 – 1 )d = 125
and => 7 + 59 d = 125
=> 59d= 125 – 7
then => d = = 2
now, a31 = a + (n – 1)d
= 7 + (31 – 1) x 2
and = 7 + 30 x 2
Hence = 67
Question 19.
The sum of the 4th and the 8th terms of an A.P. is 24 and the sum of the sixth term and the tenth term is 34. Find the first three terms of the A.P.
Answer 19
In an A.P.
T4 + T8 = 24
T6 + T10 =34
Let a be the first term and d be the common difference
Question 20.
If the third term of an A.P. is 5 and the seventh terms is 9, find the 17th term
Answer 20
Let the first term of an A.P. = a
and the common difference of the given A.P. = d
As, we know that,

exercise 10 (B), Solutions of Chapter-10 Arithmetic Progression Concise Maths
Question 1
In an A.P., ten times of its tenth term is equal to thirty times of its 30th term. Find its 40th term
Answer 1
In an A.P.
10T10 = 30T30
We know that,
If m times of mth term = n times of nth term
Then its (m + n)th term = 0
∴ 10T10 = 30T30
Then T10+30 = 0 or T40 = 0
Question 2
How many two-digit numbers are divisible by 3 ?
Answer 2
Two digits numbers are 10 to 99
and two digits numbers which are divisible
by 3 are 12, 15, 18, 21,….99
Here a = 12, d = 3 and l = 99
Now, Tn = l = a + (n – 1 )d
99 = 12 + (n – 1) x 3
=> 99 – 12 = 3(n – 1)
=> 87 = 3(n – 1)
=> = n – 1
=> n – 1 = 29
n = 29 + 1 = 30
Number of two digit number divisible by 3 = 30
Question 3
Which term of A.P. 5, 15, 25, will be 130 more than its 31st term?
Answer 3
A.P. is 5, 15, 25,….
Let Tn = T31 + 130
In A.P. a = 5, d = 15 – 5 = 10
∴ Tn = a + 30d + 130 = 5 + 30 x 10 +130
= 5 + 300 + 130 = 435
∴ n + (n – 1)d = 435
=> 5 + (n – 1) x 10 = 435
=> (n – 1) x 10 = 435 – 5 = 430
n – 1 = = 43
n = 43 + 1 = 44
∴ The required term is 44th.
Question 4.
Find the value of p, if x, 2x + p and 3x + 6 are in A.P.
Answer 4
A.P. is x, 2x + p, 3x + 6
2x + p – x = 3x + 6 – 2x + p
x + p = x – p + 6
=>2p = 6
=>p = = 3
Hence p = 3
Question 5.
If the 3rd and the 9th terms of an arithmetic progression are 4 and – 8 respectively, which term of it is zero?
Answer 5
In an A.P.
T3 = 4 and T9 = – 8
Let a be the first term and d be the common difference, then

Question 6
How many three-digit numbers ate divisible by 87 ?
Answer 6
Three digits numbers are 100 to 999

999 – 42 = 957
Numbers divisible by 87 will be 174,….., 957
Let n be the number of required three digit number.
Here, a = 174 and d = 87
l = 957 = a + (n – l)d
= 174 + (n – 1) x 87
=> (n – 1) x 87 = 957 – 174 = 783
n – 1 = = 9
n = 9 + 1 = 10
There are 10 such numbers
Question 7.
For what value of n, the nth term of A.P. 63, 65, 67,….. and nth term of A.P. 3, 10, 17,…… are equal to each other?
Answer 7
We are given,
nth term of 63, 65, 67, …….
=> nth term of 3, 10, 17,…….
=> In first A.P., a1 = 63, d1 = 2
and in second A.P., a2 = 3, d2 = 1

∴ Required nth term will be 13th
Question 8.
Determine the A.P. whose 3rd term is 16 and the 7th term exceeds the 5th term by 12.
Answer 8
T3 = 16, and T7 = T5 + 12
Let a be the first term and d be the common difference
T3 = a + 2d – 16 …(i)
T7 = T5 + 12
a + 6d = a + 4d + 12
=> 6d – 4d = 12 => 2d – 12
so => d = = 6
and in (i),
a + 6 x 2 = 16
=> a + 12 = 16
hence =>a = 16 – 12 = 4
A.P. will be 4, 10, 16, 22,……
Question 9.
If numbers n – 2, 4n – 1 and 5n + 2 are in A.P., find the value of n and its next two terms.
ANSWER 9
n – 2, 4n – 1 and 5n + 2 are in A.P.
(4n – 1) – (n – 2) = (5n + 2) – (4n – 1)
=> 4n – 1 – n + 2 = 5n + 2 – 4n + 1
=> 4n – n – 5n + 4n = 2 + 1 + 1 – 2
and =>2n = 2 =>n = = 1
4n – 1 = 4 x 1 – 1 = 4 – 1 = 3
n – 2 = 1 – 2 = – 1
and 5n + 2 = 5 x 1 + 2 = 5 + 2 = 7
A.P. is – 1, 3, 7, ……
and next 2 terms will 11, 15
Question 10.
Determine the value of k for which k2 + 4k + 8, 2k2 + 3k + 6 and 3k2 + 4k + 4 are in A.P.
Answer 10:
k2 + 4k+ 8, 2k2 + 3k + 6 and 3k2 + 4k + 4 are in A.P.
(2k2 + 3k + 6) – (k2 + 4k + 8)
= (3k2 + 4k + 4) – (2k2 + 3k + 6)
and => 2k2 + 3k + 6 – k2 – 4k – 8
= 3k2 + 4k + 4 – 2k2 – 3k – 6
so => k2 – k – 2 = k2 + k – 2
=> k2 – k – k2 – k = – 2 + 2
therefore => – 2k = 0
=> k = 0
Hence k = 0
Question 11.
If a, b and c are in A.P. show that :
(i) 4a, 4b and 4c are in A.P.
(ii) a + 4, b + 4 and c + 4 are in A.P.
Answer 11
a, b, c are in A.P.
2b = a + c
(i) 4a, 4b and 4c are in A.P.
If 2(4b) = 4a + 4c
and If 8b = 4a + 4c
2b = a + c which is given
(ii) a + 4, b + 4 and c + 4 are in A.P.
If 2(b + 4) = a + 4 + c + 4
and If 2b + 8 = a + c + 8
If 2b = a + c which is given
Question 12.
An A.P. consists of 57 terms of which 7th term is 13 and the last term is 108. Find the 45th term of this A.P.
Answer 12
Number of terms in an A.P. = 57
T7 = 13, l= 108
To find T45
Let a be the first term and d be the common difference
=> a + 6d = 13 …(i)
a + (n – 1 )d= 108
=> a + (57 – 1 )d= 108
=> a + 56d = 108
Subtracting,


Question 13
4th term of an A.P. is equal to 3 times its term and 7th term exceeds twice the 3rd term by 1. Find the first term and the common difference.
Answer 13
In A.P
T4 = 3 x T1
T7 = 2 x T3 + 1
Let a be the first term and d be the common
difference

Question 14.
The sum of the 2nd term and the 7th term of an A.P. is 30. If its 15th term is 1 less than twice of its 8th term, find the A.P.
Answer 14
In an A.P.
T2 + T7 = 30
T15 = 2T8 – 1
Let a be the first term and d be the common difference
a + d + a + 6d = 30

Question 15.
In an A.P., if mth term is n and nth term is m, show that its rth term is (m + n – r)
Answer 15
In an A.P.
Tm = n and Tn = m
Let a be the first term and d be the common difference, then
Tm = a + (m – 1 )d = n

Question 16.
Which term of the A.P. 3, 10, 17,…..will be 84 more than its 13th term?
Answer 16
A.P. is 3, 10, 17,….
Here, a = 2, d = 10 – 3 = 7

Exe- 10(C), Concise Maths Solutions of Chapter-10 Arithmetic Progression (AP)
Question 1.
Find the sum of the first 22 terms of the A.P. : 8, 3, – 2,…..
Answer 1
A.P. = 8, 3, – 2,…..
Here, a = 8, d = 3 – 8 = – 5, n = 22

Question 2.
How many terms of the A.P. : 24, 21, 18, must be taken so that their sum is 78 ?
Answer 2
Let n term of the given A.P. be taken
and A.P. = 24, 21, 18……
Let n be the number of terms.
Here, a = 24, d = 21 – 24 = – 3, Sn = 78

Question 3.
Find the sum of 28 terms of an A.P. whose nth term is 8n – 5.
Answer 3
nth term (Tn) = 8n – 5
T1 = 8 – 5 = 3
and T2 = 8 x 2 – 5 = 16 – 5 = 11
therefor T3 = 8 x 3 – 5 = 24 – 5 = 19
so A.P. is 3, 11, 19,
hence , a = 3,d = 11 – 3 = 8 and n = 28

Question 4.
Find the sum of :
(i) all odd natural numbers less than 50.
(ii) first 12 natural numbers each of which is a multiple of 7.
Answer 4
(i) Sum of all odd natural numbers less then 50
1 + 3 + 5 + 7 +…….+ 49
Here a = 1, d = 3 – 1 = 2
Let n be the number of term, then
49 = a + (n – 1)d
=> 49 = 1 + (n – 1) x 2
=> 49 – 1 = (n – 1) x 2

Question 5.
Find the sum of first 51 terms of an A.P. whose 2nd and 3rd terms are 14 and 18 respectively.
Answer 7
Sum of first 51 terms of an A.P. in which T2 = 14 and T3 = 18
d = T3 – T2 = 18 – 14 = 4
and T2 = a + d
=> 14 = a + 4
=> a = 14 – 4 = 10
A.P. = 10, 14, 18, 22,….

Question 6.
The sum of first 7 terms of an A.P. is 49 and that of first 17 terms of it is 289. Find the sum of first tt terms.
Solution:
S7 = 49,
S17 = 289

Question 7.
The first term of an A.P. is 5, the last term is 45 and the sum of its terms is 1000. Find the number of terms and the common difference of the A.P.
Answer 7
First term of an AP (a) = 5
Last term = 45
and Sn = 1000

Question 8.
Find the sum of all natural numbers between 250 and 1000 which are divisible by 9.
Answer 8
All natural numbers between 250 and 1000 which are divisible by 9 are
252, 261, 270……999

Question 9.
The first and the last terms of an A.P. are 34 and 700 respectively. If the common difference is 18, how many terms are there and what is their sum?
Answer 9
In an A.P.
T1 = a = 34, l = 700, d = 18
Let n be the number of terms, then
Tn = a + (n – 1 )d
=> 700 = 34 + (n – 1) x 18
=> 700 – 34 = 18(n – 1)
=> 180 – 0 = 666

Question 10.
In an A.P. the first term is 25, nth term is – 17 and the sum of n terms is 132. Find n and the common difference.
Answer 10
In an A.P.
First term (a) = 25
nth term = – 17
and Sn = 132
Let d be the common difference


Question 11.
If the 8th term of an A.P. is 37 and the 15th term is 15 more than the 12th term, find the A.P. Also, find the sum of first 20 terms of this A.P.
Answer 11
In an A.P.
8th term = 37
15th term = 12th term + 15
Let a be the first term and d be the common difference, then

Question 12.
Find the sum of all multiples of 7 lying between 300 and 700.
Answer 12
Multiples of 7 lying between 300 and 700 are 301, 308, 315, 322,…., 693

Question 13.
The sum of n natural numbers is 5n² + 4n. Find its 8th term
.Answer 13
Sum of n natural number = 5n² + 4n
Sn = 5n² + 4n
S1(a) = 5 x (1)² + 4 x 1
= 5 + 4 = 9
S2 = 5(2)² + 4 x 2 = 20 + 8 = 28
S2 – S1 = T2 = 28 – 9 = 19
=> a + d = 19 => 9 + d = 19
d = 19 – 9 = 10
a = 9, d = 10
T8 = a + (n – 1 )d = 9 + (8 – 1) x 10
= 9 + 7 x 10 = 9 + 70 = 79
Question 14.
The fourth term of an A.P. is 11 and the eighth term exceeds twice the fourth term by 5. Find the A.P. and the sum of first 50 terms.
Answer 14
In an A.P.
T4 = 11
T8 = 2T4 + 5
Now, a + 3d = 11
a + 7d = 2 x 11 + 5 = 22 + 5 = 27
Subtracting, 4d = 16
=> d = = 4

EXE- 10 (D), Concise (Selina Publications) Solutions for ICSE Maths Chapter-10 Arithmetic Progression
Question 1.
Find three numbers in A.P. whose sum is 24 and whose product is 440.
Answer 1
Let three numbers be a – d, a, a + d
a – d + a + a + d = 24

Question 2.
The sum of three consecutive terms of an A.P. is 21 and the slim of their squares is 165. Find these terms.
Answer 2
Let three consecutive numbers in A.P. are
a – d, a, a + d
a – d + a + a + d = 21


Question 3.
The angles of a quadrilateral are in A.P. with common difference 20°. Find its angles.
Answer 3
Let the angles of a quadrilateral are
a, a + d, a + 2d, a + 3d
d= 20°
a + a + d + a + 2d + a + 3d = 360°
(Sum of angles of a quadrilateral)
=> 4a + 6d = 360°
=> 4a + 6 x 20° = 360°
=> 4a + 120° = 360°
=> 4a = 360 – 120° = 240°
a = = 60°
Angles are 60°, 80°, 100°, 120°
Question 4.
Divide 96 into four parts which are in A.P. and the ratio between product of their means to product of their extremes is 15 : 7.
Answer 4
Number = 96
Let its four parts be a, a + d, a + 2d, a + 3d
a + a + d + a + 2d + a + 3d = 96
=> 4a + 6d = 96
=> 2a + 3d = 48 …(i)
Product of means : Product of extremes = 15 : 7

Question 5.
Find five numbers in A.P. whose sum is and the ratio of the first to the last terms is 2 : 3.
Answer 5
Let 5 numbers in A.P. be
a, a + d, a + 2d, a + 2d, a + 4d
a + a + d + a + 2d + a + 3d + a + 4d =


Question 6.
Answer 6
Number = 207
Let part be a – d, a, a + d
a – d + a + a + d = 207

Question 7.
The sum of three numbers in A.P. is 15 the sum of the squares of the extreme is 58. Find the numbers
Solution:
Let three numbers in A.P. be a – d, a, a + d
a – d + a + a + d = 15
=> 3a = 15


Question 8.
Find four numbers in A.P. whose sum is 20 and the sum of whose squares is 120.
Answer 8
Let four numbers in A.P. be
a – 3d, a – d, a + d, a + 3d
a – 3d + a – d + a + d + a + 3d = 20

Question 9.
Insert one arithmetic mean between 3 and 13.
Answer 9
Let A be the arithmetic mean between 3 and 13
A =
=
= 8
Question 10.
The angles of a polygon are in A.P. with common difference 5°. If the smallest angle is 120°, find the number of sides of the polygon.
Answer 10
Angles of a polygon are in A.P.
and common difference (d) = 5°
Smallest angle (a) = 120°
Let n be the number of sides of the polygon then sum of angles = (2n – 4) x 90°
a = 120° and d = 5°

Question 11.
,
and
are in A.P
S.T : bc, ca and ab are also in A.P
Solution:
,
and
are in A.P
We have to show that

EXERCISE- 10 (E) Arithmetic Progression Solutions for Concise Maths
Question 1.
Two cars start together in the same direction from the same place. The first car goes at uniform speed of 10 km h-1. The second car goes at a speed of 8 km h-1 in the first hour and thereafter increasing the speed by 0.5 km h-1 each succeeding hour. After how many hours will the two cars meet ?
Answer 1
Speed of first car = 10 km/hr
Speed of second car = 8 km/hr in first hour

Question 2. Arithmetic Progression Concise Solutions
A sum of Rs 700 is to be paid to give seven cash prizes to the students of a school for their overall academic performance. If the cost of each prize is Rs 20 less than its preceding prize; find the value of each of the prizes.
Answer 2
Total amount (Sn) = Rs 700
Cost of each prize is Rs 20 less than its preceding price or d = – 20
d = – 20 and n = 7

Question 3.
An article can be bought by paying Rs 28,000 at once or by making 12 monthly installments. If the first installment paid is Rs 3,000 and every other installment is Rs 100 less than the previous one, find :
(i) amount of installment paid in the 9th month
(ii) total amount paid in the installment scheme.
Answer 3
Total price of an article = Rs 28000
No. of installments (n) = 12
First installment (a) = RS 3000
d = Rs 100

Question 4.
A manufacturer of TV sets produces 600 units in the third year and 700 units in the 7th year. Assuming that the production increases uniformly by a fixed number every year, find :
(i) the production in the first year.
(ii) the production in the 10th year.
(iii) the total production in 7 years.
Answer 4
A manufacture of TV sets, he produces
No. of units in 3rd year = 600
No. of units in 7th year = 700
Let a be the first term and d be the common difference, then

Question 5.
Mrs. Gupta repays her total loan of Rs 1.18,000 by paying installments every month. If the installment for the first month is Rs 1,000 and it increases by RS 100 every month, what amount will she pay as the 30th installment of loan? What amount of loan she still has to pay after the 30th installment?
Answer 5
Total loan to be paid by Mrs. Gupta = Rs 118000
Installment for the first month (a) = Rs 1000


Question 6. Arithmetic Progression Concise Solutions
In a school, students decided to plant trees in and around the school to reduce air pollution. It was decided that the number of trees, that each section of each class will plant, will be five times of the class to which the respective section belongs. If there are 1 to 10 classes in the school and each class has three sections, find how many trees were planted by the students?
Answer 6
Number of classes = 10
Number of sections of each class = 3
Total number of sections = 10 x 3 = 30
Each section plant tree = 5 times of the class
Each section of 1st class will plant = 1 x 15 = 15

EXERCISE -10 (F), Arithmetic Progression Concise Solutions for ICSE Maths
Question 1.
The 6th term of an A.P. is 16 and the 14th term is 32. Determine the 36th term.
Answer 1
Let the first term and common difference of an A.P. be a and d
As, we know that,

Question 2.
If the third and the 9th terms of an A.P. term is 12 and the last term is 106. Find the 29th term of the A.P.
Answer 2
Let the first term and common difference of an A.P. be a and d.
As, we know that,
an = a + (n – 1 )d
a3 = a + (3 – 1 )d = a + 2d
Similarly,


Question 3.
An A.P. consists of 50 terms of which 3rd term is 12 and the last term is 106. Find the 29th term of the A.P.
Answer 3
Number of terms in an A.P. = 50
T3= 12, l = 106
To find T29
Let a be the first term and d be the common difference
=> a + 2d = 12 …(i)

Question 4.
Find the arithmetic mean of :
(i) – 5 and 41
(ii) 3x – 2y and 3x + 2y
(iii) (m + n)² and (m – n)²
Answer 4
(i) Arithmetic mean between – 5 and 41
=
=
Hence = 18

Question 5.
Find the sum of first 10 terms of the A.P. 4 + 6 + 8 +…..
Answer 5
A.P. = 4 + 6 + 8 +…….
Here, a = 4, d = 6 – 4 = 2, n = 10

Question 6.
Find the sum of first 20 terms of an A.P. whose first term is 3 and the last term is 60.
Answer 6
Sum of first 20 terms of an A.P. in which
a = 3 and a20 = 60
a20 = a + (20 – 1) x d
60 = 3 + 19 x d
19d = 60 – 3
d =
= 3

Question 7.
How many terms of the series 18 + 15 + 12 +…..when added together will give 45 ?
Answer 6
A.P. is 18 + 15 + 12 +…..
Here, a = 18, d = 15 – 18 = – 3
Given : Sn = 45

Question 8. Arithmetic Progression Concise Solutions
The nth term of a sequence is 8 – 5n. Show that the sequence is an A.P.
Answer 8
Given, an = 8 – Sn
a1 = 8 – 5 x (1) = 8 – 5 = 3
a2 = 8 – 5 x (2) = 8 – 10 = – 2
a3 = 8 – 5 x (3) = 8 – 15 = – 7
We see that

Question 9.
The the general term (nth term) and 23rd term of the sequence 3, 1, – 1, – 3,……
Answer 9
The progression 3, 1, – 1, – 3,…..is A.P.
with first term (a) = 3 and common difference (d) = 1 – 3 = – 2

Question 10.
Which term of the sequence 3, 8, 13,…..is 78 ?
Answer 10
Let 78 be the nth term
a = 3, d = 8 – 3 = 5, an = 78, n = ?
a + (n – 1)d = an

Question 11.
Is – 150 a term of 11, 8, 5, 2,….. ?
Answer11
11, 8, 5, 2,….1st term, a = 11
Common difference, d = 8 – 11 = – 3
an = – 150
=> a + (n – 1 )d = – 150
=> 11 + (n – 1) ( – 3) = – 150

Question 12.
How many two digit numbers are divisible by 3 ?
Answer12
Numbers divisible by 3 are 3, 6, 9, 12,….
Hence, lowest two digit number divisible by 3 = 12
and highest two digit number divisible by 3 = 99


Question 13.
How many multiples of 4 lie between 10 and 250 ?
Answer 13
Multiples of 4 between 10 and 250 are
12, 16, 20, 24,……, 248
Here, a = 12, d = 4, l = 248

Question 14.
The sum of the 4th term and the 8th term of an A.P. is 24 and the sum of 6th term and the 10th term is 44. Find the first three terms of the A.P.
Answer 14
In an A.P.
T4 + T8 = 24
T6 + T10 = 44
Let a be the first term and d be the common difference


Question 15.
The sum of first 14 terms of an A.P. is 1050 and its 14th term is 140. Find the 20th term.
Answer 15
Given a14 = 140
we know, an = a + (n – 1) x d

Question 16 Arithmetic Progression Concise Solutions
The 25th term of an A.P. exceeds its 9th term by 16. Find its common difference.
Answer 16
nth term of an A.P. is given by tn= a + (n – 1) d.
⇒ t25 = a + (25 – 1)d = a + 24d and
t9 = a + (9 – 1)d = a + 8d
According to the condition in the question, we get
t25 = t9 + 16
⇒ a + 24d = a + 8d + 16
and ⇒ 16d = 16
Hence ⇒ d = 1
Question 17
For an A.P., show that:
(m + n)th term + (m – n)th term = 2 × mthterm
Answer 17
Let a and d be the first term and common difference respectively.
⇒(m + n)th term = a + (m + n – 1)d …. (i) and
(m – n)th term = a + (m – n – 1)d …. (ii)
From (i) + (ii), we get
(m + n)th term + (m – n)th term
= a + (m + n – 1)d + a + (m – n – 1)d
and = a + md + nd – d + a + md – nd – d
= 2a + 2md – 2d
so = 2a + (m – 1)2d
Hence = 2[ a + (m – 1)d]
= 2 × mthterm
Hence proved.
Question 18
If the nth term of the A.P. 58, 60, 62,…. is equal to the nth term of the A.P. -2, 5, 12, …., find the value of n.
Answer 18
In the first A.P. 58, 60, 62,….
a = 58 and d = 2
tn = a + (n – 1)d
⇒ tn = 58 + (n – 1)2 …. (i)
In the first A.P. -2, 5, 12, ….
a = -2 and d = 7
tn = a + (n – 1)d
⇒ tn= -2 + (n – 1)7 …. (ii)
Given that the nth term of first A.P is equal to the nth term of the second A.P.
⇒58 + (n – 1)2 = -2 + (n – 1)7 … from (i) and (ii)
and ⇒58 + 2n – 2 = -2 + 7n – 7
⇒ 65 = 5n
Hence ⇒ n = 15
Question 19
Which term of the A.P. 105, 101, 97 … is the first negative term?
Answer 19 Arithmetic Progression Concise Solutions
Here a = 105 and d = 101 – 105 = -4
Let an be the first negative term.
⇒ an < 0
and ⇒ a + (n – 1)d < 0
⇒ 105 + (n – 1)(-4)<0
So ⇒ 105 – 4n + 4 <0
⇒ 109 – 4n < 0
Hence ⇒ 109 <4n
⇒ 27.25 < n
The value of n = 28.
Therefore 28th term is the first negative term of the given A.P.
Question 20
How many three digit numbers are divisible by 7?
Answer 20
The first three digit number which is divisible by 7 is 105 and the last digit which is divisible by 7 is 994.
This is an A.P. in which a = 105, d = 7 and tn = 994.
We know that nth term of A.P is given by
tn = a + (n – 1)d.
⇒ 994 = 105 + (n – 1)7
and ⇒ 889 = 7n – 7
So ⇒ 896 = 7n
Hence ⇒ n = 128
∴ There are 128 three digit numbers which are divisible by 7.
Question 21
Divide 216 into three parts which are in A.P. and the product of the two smaller parts is 5040.
Answer 21
Let the three parts of 216 in A.P be (a – d), a, (a + d).
⇒a – d + a + a + d = 216
⇒ 3a = 216
⇒ a = 72
Given that the product of the two smaller parts is 5040.
⇒a(a – d ) = 5040
⇒ 72(72 – d) = 5040
and ⇒ 72 – d = 70
Hence ⇒ d = 2
∴ a – d = 72 – 2 = 70, a = 72 and a + d = 72 + 2 = 74
Therefore the three parts of 216 are 70, 72 and 74.
Question 22 Arithmetic Progression Concise Solutions
Can 2n2 – 7 be the nth term of an A.P? Explain.
Answer 22
We have 2n2 – 7,
Substitute n = 1, 2, 3, … , we get
2(1)2 – 7,
and 2(2)2 – 7,
so 2(3)2 – 7,
therefore 2(4)2 – 7, ….
Hence -5, 1, 11, ….
Difference between the first and second term = 1 – (-5) = 6
And Difference between the second and third term = 11 – 1 = 10
Here, the common difference is not same.
Therefore the nth term of an A.P can’t be 2n2 – 7.
Question 23
Find the sum of the A.P., 14, 21, 28, …, 168.
Answer 23
Here a = 14 , d = 7 and tn = 168
tn = a + (n – 1)d
⇒ 168 = 14 + (n – 1)7
and ⇒ 154 = 7n – 7
then ⇒ 154 = 7n – 7
so ⇒ 161 = 7n
Hence ⇒ n = 23
We know that,
Therefore the sum of the A.P., 14, 21, 28, …, 168 is 2093.
Question 24
The first term of an A.P. is 20 and the sum of its first seven terms is 2100; find the 31st term of this A.P.
Answer 24
Here a = 20 and S7 = 2100
We know that,
To find: t31 =?
tn = a + (n – 1)d
Therefore the 31st term of the given A.P. is 2820.
Question 25
Find the sum of last 8 terms of the A.P. -12, -10, -8, ……, 58.
Answer 25 Arithmetic Progression Concise Solutions
First we will reverse the given A.P. as we have to find the sum of last 8 terms of the A.P.
58, …., -8, -10, -12.
Here a = 58 , d = -2
Therefore the sum of last 8 terms of the A.P. -12, -10, -8, ……, 58 is 408.
The first term of an A.P. is 20 and the sum of its first seven terms is 2100; find the 31st term of this A.P.
Solution 24
Here a = 20 and S7 = 2100
We know that,
To find: t31 =?
tn = a + (n – 1)d
Therefore the 31st term of the given A.P. is 2820.
Return to :- Concise Selina Maths Solutions for ICSE Class-10
Thanks
Please share with your friends