ML Aggarwal Circles MCQs Class 10 ICSE Maths Solutions

ML Aggarwal Circles MCQs Class 10 ICSE Maths Solutions. We Provide Step by Step Answer of MCQs Questions for Circles as council prescribe guideline for upcoming board exam. Visit official Website CISCE  for detail information about ICSE Board Class-10.

ML Aggarwal Circles MCQs Class 10 ICSE Maths Solutions

Board	ICSE
Subject	Maths
Class	10th
Chapter-15	Circles
Writer / Book	Understanding
Topics	Solutions of MCQs
Academic Session	2024-2025

Solutions of MCQs

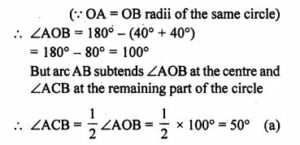
 Question 1. In the adjoining  figure, O is the centre of the circle. If ∠OAB = 40°, then ∠ACB is equal to

(a) 50°
(b) 40°
(c) 60°
(d) 70°

Answer :

In the given figure, O is the centre of the circle.
In ∆OAB,
∠OAB = 40°
But ∠OBA = ∠OAB = 40°

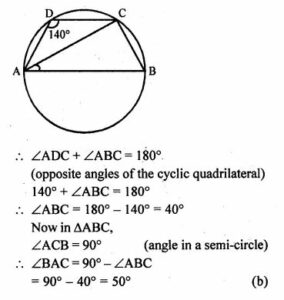
Question 2. ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC = 140°, then ∠BAC is equal to

(a) 80°
(b) 50°
(c) 40°
(d) 30°

Answer :ABCD is a cyclic quadrilateral,
AB is the diameter of the circle circumscribing it
∠ADC = 140°, ∠BAC = Join AC

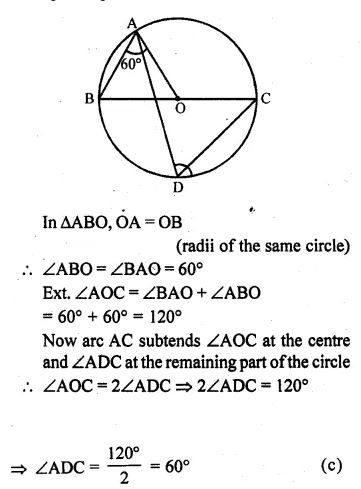
Question 3. In the adjoining figure, O is the centre of the circle. If ∠BAO = 60°, then ∠ADC is equal to
(a) 30°
(b) 45°
(c) 60°
(d) 120°

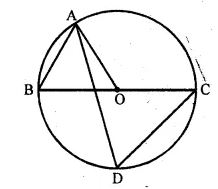
Answer :

In the given figure, O is the centre of the circle ∠BAO = 60°

Question 4. In the adjoining figure, O is the centre of a circle. If the length of chord PQ is equal to the radius of the circle, then ∠PRQ is
(a) 60°
(b) 45°
(c) 30°
(d) 15°

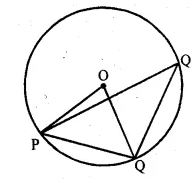
Answer :

In the given figure, O is the centre of the circle
Chord PQ = radius of the circle
∆OPQ is an equilateral triangle
∴∠POQ = 60°
Arc PQ subtends ∠POQ at the centre and
∴∠PRQ at the remaining part of the circle
∴∠PRQ = 1/2 ∠POQ = 1/2 x 60° = 30° (c)

Question 5. In the adjoining figure, if O is the centre of the circle then the value of x is
(a) 18°
(b) 20°
(c) 24°
(d) 36°

Answer :

In the given figure, O is the centre of the circle.
Join OA.

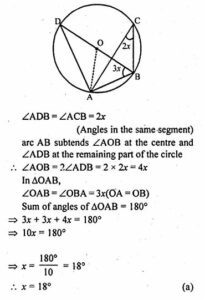
Question 6. From a point which is at a distance of 13 cm from the centre O of a circle of radius 5 cm, the pair of tangents PQ and PR to the circle are drawn. Then the area of the quadrilateral PQOR is

(a) 60 cm²
(b) 65 cm²
(c) 30 cm²
(d) 32.5 cm²

Answer :

Let point P is 13 cm from O, the centre of the circle
Radius of the circle (OQ) = 5 cm
PQ and PR are tangents from P to the circle
Join OQ and OR

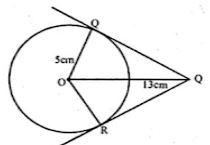
∵ PQ is tangent and OQ is the radius

∴ PQ² = OP² – OQ²

= 13² – 5²

= 169 – 25

= 144

= (12)²

∴ PQ = 12 cm

Now area of ΔOPQ = ½ PQ × OQ

= (1/2 × base × height)

= ½ × 12 × 5

= 30 cm²

∴ area of quad. PQOR = 2 × 30

= 60 cm²

Question 7. In the adjoining figure, PQ and PR are tangents from P to a circle with centre O. If ∠POR = 55°, then ∠QPR is
(a) 35°
(b) 55°
(c) 70°
(d) 80°

Answer :

In the given figure,
PQ and PR are the tangents to the circle from a point P outside it

∠POR = 55°

∵ OR is radius and PR is tangent

∴ OR ⊥ PR

∴ In ΔOPR

∠OPR = 90° – 55° = 35°

∠OPR = 2 × 35°

= 70°

Question 8. In the adjoining  figure, PA and PB are tangents from point P to a circle with centre O. If the radius of the circle is 5 cm and PA ⊥ PB, then the length OP is equal to
(a) 5 cm
(b) 10 cm
(c) 7.5 cm
(d) 5√2 cm

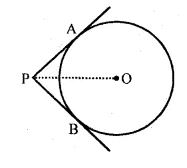
Answer :

In the given figure,
PA and PB are tangents to the circle with centre O.
Radius of the circle is 5 cm, PA ⊥ PB.

A is radius and PA is tangent to the circle

∴ OA ⊥ PA

∵ ∠APB = 90° (∵ PA ⊥ PB)

∴ ∠APO = 90° × ½ = 45°

∴ ∠APO = 90° – 45°

= 45°

i.e., OA = AP = 5 cm

---

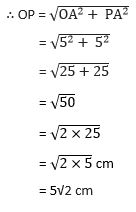
Circles MCQs

ML Aggarwal Class 10 ICSE Maths Solutions

(Page 370)

Question 9. At one end A of a diameter AB of a circle of radius 5 cm, tangent XAY is drawn to the circle. The length of the chord CD parallel to XY and at a distance 8 cm from A is

(a) 4 cm
(b) 5 cm
(c) 6 cm
(d) 8 cm

Answer :

AB is the diameter of a circle with radius 5 cm
At A, XAY is a tangent to the circle
CD || XAY at a distance of 8 cm from A
Join OC

In right ΔOEC,

OE = 8 – 5 = 3 cm

OC = 5 cm

= 4 cm

∴ CD = 2 × CE

= 2 × 4

= 8 cm

Question 10. If radii of two concentric circles are 4 cm and 5 cm, then the length of each chord of one circle which is tangent to the other is
(a) 3 cm
(b) 6 cm
(c) 9 cm
(d) 1 cm

Answer :

Radii of two concentric circles are 4 cm and 5 cm
AB is a chord of the bigger circle
which is tangent to the smaller circle at C.
Join OA, OC

OC = 4 cm, OA = 5 cm

And OC ⊥ ACB

∴ In right ΔOAC

OA² = OC² + AC²

⇒ 5² = 4² + AC²

⇒ 25 = 16 + AC²

⇒ AC² = 25 – 16

= 9

= (3)²

∴ AC = 3 cm

∴ Length of chord AB = 2 × AC

= 2 × 3

= 6 cm

Question 11. In the adjoining figure, O is the centre of a circle and PQ is a chord. If the tangent PR at P makes an angle of 50° with PQ, then ∠POQ is
(a) 100°
(b) 80°
(c) 90°
(d) 75°

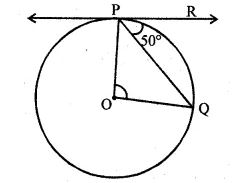
Answer :

In the given figure, O is the centre of the circle.
PR is tangent and PQ is chord ∠RPQ = 50°
OP is radius and PR is tangent to the circle

∠RPQ = 50°

∵ OP is radius and PR is tangent to the circle

∴ OP ⊥ PR

But ∠OPQ + RPQ = 90°

⇒ ∠OPQ + 50° = 90°

⇒ ∠OPQ = 90° – 50°

= 40°

∵ OP = OQ (radii of the same circle)

∴ ∠OQP = ∠OPQ = 40°

And ∠POQ = 180 ̊ – (∠OPQ + ∠OQP)

= 180° – (40°+40°)

= 180° – 80°

= 100°

Question 12. In the adjoining figure, PA and PB are tangents to a circle with centre O. If ∠APB = 50°, then ∠OAB is equal to
(a) 25°
(b) 30°
(c) 40°
(d) 50°

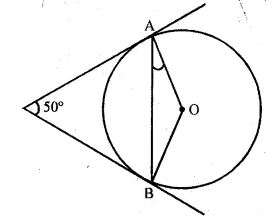
Answer 

(a) 25°

In the given figure,

PA and PB are tangents to the circle with centre O.

∠APB = 50°

But ∠AOB + ∠APB = 180°

∠AOB + 50° = 180°

∠AOB = 180° – 50°

= 130°

In ΔOAB,

OA = OB (radii of the same circle)

∠OAB = ∠OBA

But ∠OAB + ∠OBA = 180° – ∠AOB

= 180° – 130°

= 50°

∠OAB = 50 ̊/2

= 25°

Question 13. In the adjoining figure, sides BC, CA and AB of ∆ABC touch a circle at point D, E and F respectively. If BD = 4 cm, DC = 3 cm and CA = 8 cm, then the length of side AB is
(a) 12 cm
(b) 11 cm
(c) 10 cm
(d) 9 cm

Answer :

In the given figure,
sides BC, CA and AB of ∆ABC touch a circle at D, E and F respectively.
BD = 4 cm, DC = 3 cm and CA = 8 cm

∵ BD and BF are tangents to the circle

∴ BF = BD = 4 cm

Similarly, CD = CE = 3 cm

∴ AE = AC – CE = 8 – 3 = 5 cm

And AF = AE = 5 cm

Now AB = AF + BF

= 5 + 4

= 9 cm

Question 14. In the adjoining figure, sides BC, CA and AB of ∆ABC touch a circle at the points P, Q and R respectively. If PC = 5 cm, AR = 4 cm and RB = 6 cm, then the perimeter of ∆ABC is
(a) 60 cm
(b) 45 cm
(c) 30 cm
(d) 15 cm

Answer :

In the given figure, sides BC, CA and AB of ∆ABC
touch a circle at P, Q and R respectively
PC = 5 cm, AR = 4 cm, RB = 6 cm

∴ AR and AQ are tangents to the circles

∴ AQ = AR = 4 cm

Similarly CQ = CP = 5 cm

And BP = BR = 6 cm

Now AB = AR + BR

= 4 + 6

= 10 cm

BC = BP + CP

= 6 + 5

= 11 cm

AC = AQ + CQ

= 4 + 5

= 9 cm

∴ Perimeter of the ΔABC = AB + BC + CA

= 10 + 11 + 9

= 30 cm

Question 15. PQ is a tangent to a circle at point P. Centre of circle is O. If ∆OPQ is an isosceles triangle, then ∠QOP is equal to
(a) 30°
(b) 60°
(c) 45°
(d) 90°

Answer :

PQ is tangent to the circle at point P centre of the circle is O.

ΔOPQ is an isosceles triangle

OP = PQ

∵ OP is radius and PQ is tangent to the circle

∴ OP ⊥ PQ i.e., ∠OPQ = 90°

∵ OP = PQ (∵ ΔOPQ is an isosceles triangle)

∴ ∠QOP = ∠PQO = 90°/2

= 45°

Question 16. In the adjoining figure, PA and PB are tangents at points A and B respectively to a circle with centre O. If C is a point on the circle and ∠APB = 40°, then ∠ACB is equal to
(a) 80°
(b) 70°
(c) 90°
(d) 140°

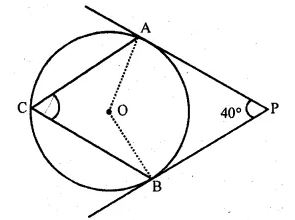
Answer :

In the given figure,
PA and PB are tangents to the circle at A and B respectively
C is a point on the circle and ∠APB = 40°
But ∠APB + ∠AOB = 180°

⇒ 40° + ∠AOB = 180°

⇒ ∠AOB = 180° – 40° = 140°

Now arc AB subtends ∠AOB at the centre and ∠ACB is on the remaining part of the circle

∴ ∠ACB = ½ ∠AOB

= ½ × 140°

= 70°

---

Circles MCQs

ML Aggarwal Class 10 ICSE Maths Solutions

 (Page 371)

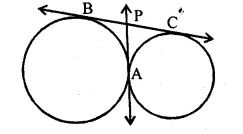
Question 17. In the adjoining figure, two circles touch each other at A. BC and AP are common tangents to these circles. If BP = 3.8 cm, then the length of BC is equal to
(a) 7.6 cm
(b) 1.9 cm
(c) 11.4 cm
(d) 5.7 cm

Answer :

In the given figure, two circles touch each other at A.
BC and AP are common tangents to these circles
BP = 3.8 cm

∵ PB and PA are the tangents to the first circle

∴ PB = PA = 3.8 cm

Similarly PC and PA are tangents to the second circle

∴ PA = PC = 3.8 cm

BC = PB + PC

= 3.8 + 3.8

= 7.6 cm

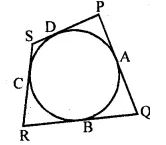
Question 18. In the adjoining figure, if sides PQ, QR, RS and SP of a quadrilateral PQRS touch a circle at points A, B, C and D respectively, then PD + BQ is equal to
(a) PQ
(b) QR
(c) PS
(d) SR

Answer :

In the given figure,
sides PQ, QR, RS and SP of a quadrilateral PQRS
touch a circle at the points A, B, C and D respectively
PD and PA are the tangents to the circle
∴ PA = PD …(i)
Similarly, QA and QB are the tangents
∴ QA = QB …(ii)
Now PD + BQ = PA + QA = PQ (a)
[From (i) and (ii)]

Question 19. In the adjoining figure, PQR is a tangent at Q to a circle. If AB is a chord parallel to PR and ∠BQR = 70°, then ∠AQB is equal to
(a) 20°
(b) 40°
(b) 35°
(d) 45°

Answer :

In the given figure, PQR is a tangent at Q to a circle.

Chord AB || PR and ∠BQR = 70°

BQ is chord and PQR is a tangent

∠BQR = ∠A (Angles in the alternate segments)

∵ AB || PQR

∴ ∠BQR = ∠B (alternate angles)

∴ ∠A = ∠B = 70°

∴ ∠AQB + ∠A + ∠B = 180° (Angles of a triangle)

⇒ ∠AQB + 70° + 70° = 180°

⇒ ∠AQB + 140° = 180°

∴ ∠AQB = 180° – 140°

= 40°

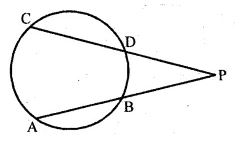
Question 20. Two chords AB and CD of a circle intersect externally at a point P. If PC = 15 cm, CD = 7 cm and AP = 12 cm, then AB is
(a) 2 cm
(b) 4 cm
(c) 6 cm
(d) none of these

Answer :

In the given figure,
two chords AB and CD of a circle intersect externally at P.
PC = 15 cm, CD = 7 cm, AP = 12 cm
Join AC and BD

In ΔAPC and ΔBPD

∠P = ∠P (common)

∠A = ∠BDP {Ext. of a cyclic quad. is equal to its interior opposite angles}

∴ ΔAPC ~ ΔBPD (AA axiom)

PA/PD = PC/PB

PA.PB = PC.PD

12.PB = 15 × 8 (PD = 15 – 7 = 8)

PB = (15 × 8)/12 = 10

∴ AB = AP – PB

= 12 – 10

= 2 cm

—  : End of ML Aggarwal Circles MCQs Class 10 ICSE Maths Solutions : –

Return to:   ML Aggarwal Solutions for ICSE Class-10

Thanks

Please Share with Your Friends